Guest Post by Willis Eschenbach
For those who enjoy mathematical puzzles, I’m putting this one out there for your pleasure.
Suppose we have a 1 metre by 1 metre by 1 metre concrete block floating in outer space. For the purposes of the puzzle, let’s suppose that there is no longwave background radiation at all.
The block is insulated on four sides, as shown in blue below, with the front and back of the block uninsulated. We’ll further suppose that the insulation is made of Unobtanium, which is a perfect insulator, so no heat at all is lost from the four insulated sides.
Next, let’s assume the emissivity “epsilon” of the concrete block is 0.95. [And as a commenter pointed out, let’s assume that the emissivity and absorptivity across the spectrum are both 0.95 everywhere. Yes, I know this isn’t reality, but it’s a thought experiment.] And we’ll say that the thermal conductivity “k” of the concrete is equal to 0.8 watts per metre per kelvin (0.8 W/m K^-1)
Finally, let’s assume that it gets full-time sunshine on the front side at a rate of 1360 watts per square metre (W/m2). Figure 1 shows the experimental setup.

Figure 1. Setup for the thought experiment. The concrete block (gray) is a one-metre cube. The blue insulation prevents any heat from escaping from the four sides. However, the block is free to gain heat by radiation on the front side, and to lose heat by radiation from both the front and the back sides.
Here’s the puzzle. If the concrete block starts at absolute zero, it will slowly warm up until it is at steady-state, neither warming nor cooling.
So the question is: at steady-state, what will be the temperature T_hot of the hot side and the temperature T_cold of the opposite cold side?
w.
REQUESTS: First, let me ask that when you comment, please quote the exact words you’re discussing. It avoids many problems.
Next, as my high school math teacher would say, please show your work.
Finally, please focus on the question and the answers, and leave out all ad hominems, personal comments, and insults, as well as abjuring any discussion of your opponent’s education, age and species of likely progenitors, improbable sexual habits, or overall intelligence.
Psst! Hey there, nerd at the desk to my right- could you move your left arm so I can see what you’re writing? I have no idea how I got into this exam, and I don’t want to look like a total moron.
The Stephan-Boltzmann Constant = 5.670 374 419 x 10^-8 W m^-2 K^-4 Exact.
See Numerical value 5.670 374 419… x 10-8 W m-2 K-4>NIST Fundamental Physical Constants CODATA 2018
https://physics.nist.gov/cgi-bin/cuu/Value?sigma|category=physchem_in
Willis
can you give me the answer on the back page, i got another class i have to get to.
Stefan-Boltzman, Watson, Stefan-Boltzman.
Assuming an emissivity coefficient = 1 (at steady state the block behaves as a perfect black body, according to the statement), then:
T = 393.54 °K
T = 120.4 °C
Impossible to write the equation here.
That’s what I made it on the hot side and -270.4K on the cold side.
-270.4 K would not be radiating anything. So it would be very similar to having the backside completely insulated, and remove this insulation after 10,000 hour of the sunlight shining on front.
And before removing insulation the back side should be about 120 C. After insulation on back side is removed, the surface rapidly cools. And with insulation removed for 10,000 hours, most of block will be hot {120 C} and it seems backside will be warmer than -270 K, seems likely it’s about 100 K.
Oh, -270.4 K is wrong it should -270.4 C to make any sense.
-270.4 K could be the outside temperature of the unobtanium sides. 😉
Since 0 C = 273.15 K, you have one side at 393.54 K, and the other at 120.4 + 273.15 = 393.55 K. That’s the same to almost 5 significant digits. I assume that’s what you intended?
Front and back the same to almost 5 significant digits?
42
Lol
+1
So are you saying 1 or 43?
..and thanks for the fish?
I think Tyler is correct. Since we know that is the answer to the ultimate question. that no one was able to answer. Judging from the myriad responses below it would appear to meet that criteria. So, I say we name him President of the Galaxy as his reward for the right answer.
Correct – but, what I need to know is, What happened to the CO2 released whilst the concrete was setting – was it left on Earth???!!!
T-hot 260F
T-cold -280F
You have the hot side being colder than the cold side?
Is 260 lower than -280?
Hot side 126dC; cold side 107dC.
126dC?
dC = dangCold?
Space – the Hotter Frontier
One of the heated issues underlying greenhouse theory is whether space is hot or cold.
Greenhouse theory says that without an atmosphere the earth would be exposed to a near zero outer space and become a frozen ice ball at -430 F, 17 K.
Geoengineering techniques that increase the albedo, the ISS’s ammonia refrigerant air conditioners, an air conditioner in the manned maneuvering unit, space suits including thermal underwear with chilled water tubing, UCLA Diviner lunar data and Kramm’s models (Univ of AK) all provide substantial evidence that outer space is relatively hot.
But outer space is neither hot nor cold.
By definition and application temperature is a relative measurement of the molecular kinetic energy in a substance, i.e. solid, liquid, gas. No molecules (vacuum), no temperature. No kinetic energy (absolute zero), no temperature. In the void & vacuum of outer space the terms temperature, hot, cold are meaningless, like dividing by zero, undefined. Same reason there is no sound in space – no molecules.
However, any substance capable of molecular kinetic energy (ISS, space walker, satellite, moon, earth) placed in the path of the spherical expanding solar photon gas at the earth’s average orbital distance will be heated per the S-B equation to an equilibrium temperature of: 1,368 W/m^2 = 394 K, 121 C, 250 F.
Like a blanket held up between a camper and campfire the atmosphere reduces the amount of solar energy heating the terrestrial system and cools the earth compared to no atmosphere.
This intuitively obvious as well as calculated and measured scientific reality refutes the greenhouse theory which postulates the exact opposite even incorrectly claiming the naked earth would be a -430 F ice ball.
Zero greenhouse effect, Zero CO2 global warming and Zero man caused climate change.
–Nick Schroeder February 28, 2020 at 6:58 am
Space – the Hotter Frontier
One of the heated issues underlying greenhouse theory is whether space is hot or cold.–
Space is neither hot or cold. The lunar surface, which very close to vacuum of space, is about 120 C in sunlight and after 2 weeks the surface is about 100 K.
But the top surface of Moon surface is about 0 C just before before the sun goes down- or sun at low angle above horizon is not lunar surface which is level {a rock vertical to surface can be he heated by the sun by a lot {120 C}.
–Greenhouse theory says that without an atmosphere the earth would be exposed to a near zero outer space and become a frozen ice ball at -430 F, 17 K.–
No greenhouse theory doesn’t say this. But people might imagine that Earth without an atmosphere could be similar to the Moon. But the Moon is quite different than Earth and the Moon has slow rotation. And if Moon had enough atmosphere to make any difference {a Mars atmosphere would not make much difference} then the daytime lunar surface temperature would be cooler and night time temperature would be much warmer.
But what is as important as Earth’s dense atmosphere is that 70% of Earth is covered by oceans. Or if Moon had thick atmosphere and an ocean covering 70% of the surface, it would quite similar to Earth.
And like Earth is matters where the Land areas are, within the global ocean.
Or we currently in an Ice Age, this is related to where the land is related to the ocean, in non Ice Age conditions on Earth, Earth has much higher average temperature than 15 C.
Yes, without an atmosphere, the Earth would be hot. Similar to but not identical to the Moon on the Day Side. The Moon’s daylight side remains in Solar Radiation for about 356 hours before It’s Tidally Locked orbit begin to remove the effects of Solar Radiation for a period of 356 hours. The Earth will only heat from Solar Radiation for a period of around 12 hours at the equator before cooling for the next 12 hour period.
So the Earth will not get as hot as the Moon during daylight hours or cool as significantly during the nighttime hours
Nick,
One hundred kilometers up, at the edge of space, there are about one million million million molecules per cubic meter.
Further up at the space station, there are a hundred times less…only ten trillion per cubic meter.
Halfway to the Moon, away from the influence of the atmosphere, there are still a lot of molecules.
Some 7,000,000 per cubic meter.
I do not know where this empty place with no temperature is.
And what does it mean to say at a temperature of absolute zero, there is no temperature?
You said what the temperature is in the same sentence you said there was not one.
If we are doing science, let’s do science.
If you want to demonstrate that 7 million molecules per cubic meter is not a lot of molecules…do that.
But if the point is that with no molecules there is no temperature, and yet there are molecules, what exactly are you saying?
The upper atmosphere of the Earth is called the thermosphere cause it is hot, and it gets hotter when solar activity is high.
This is because the average velocity of the molecules is high, even though there are not a lot of them.
BTW…here is enough air there (at the height that low Earth orbit objects are placed) to have caused the crash of the Spacelab satellite when the atmosphere heated up and expanded more than anticipated back in the 1970s, IIRC.
I am wondering if anyone who knew little to nothing about this subject would be able to learn anything by reading these comments.
I feel like I just got stupider.
Space has no temp because it has no molecules. But it does have some.
Space is hot because stuff in the unfiltered sunshine above the Earth gets real hot on one side?
Huh?
That has nothing to do with the temperature of space.
Even here in Earth we do not count the temperature of an object in direct sun to be an actual air temp.
All measurements are specifically understood to be, and measured in, the shade.
Because otherwise you are measuring a radiation effect, not anything to do with the motion of the molecules.
I am not complaining or anything…you guys comment however you want.
I just do not understand what the point is.
Personally, I like to say things that inform people.
There are 3.3455×10^22 molecules of water in a gram of water. 334,550,000,000,000,000,000,000 (334 sextrillion molecules…
A gram of water is approximately equal to +/- 1/4 teaspoon.
7,000,000 molecules of water takes up 1/4.9357143e+15 (1/4,935,714,300,000,000) 1-5 quadrillionth of a teaspoon. 7,000,000 is a very large number unless you are talking about a very small object
7,000,000 water molecules will fit on the
HeadPoint of a NeedleYour examples would be more apt if you used gas molecules in air.
Nick S
You are making a conceptual error of great importance:
“Greenhouse theory says that without an atmosphere the earth would be exposed to a near zero outer space and become a frozen ice ball at -430 F, 17 K.”
Greenhouse gas theory makes no such assertion. The Greenhouse gas theory (as expounded by the IPCC and Gavin and lots of others who are conceptually misdirected) reads more like this:
“Greenhouse theory says that without any GHG’s in the atmosphere the near-earth average air temperature would the same as the average temperature of the surface of the moon, which has no atmosphere.”
“Greenhouse gas theory” has nothing to say about planets without any atmosphere at all. Greenhouse gas theory is limited to the effects of greenhouse gases. Greenhouse gas theory is subject to the laws of physics, not a progenitor of them.
It the Earth had an atmosphere containing no GHG’s at all, Gavin says (contravening physical laws governing convective heat transfer) the air would be as cold as the surface of the moon. That is how screwed up is the conceptual framework within which NASA/GISS operates.
Thank You.
I have had the same thoughts since I first heard of this CC Propaganda.
As a Nuclear Engineer I learned about flow in a pipe, and Nucleate Boiling. Even with flows of hundreds of feet per second a “film” will persist on the surface of the pipes in coolers or heat exchangers. This film is an insulator, that is, it slows down the transfer of heat from the pipe to the liquid or vice versa. This same effect is present in the atmosphere surrounding the earth. The reason for dimples on golf balls is to minimize this persistent film on the golf ball. Relatively, the Earth is a thousand times smother than the dimpled gulf ball thus the atmosphere is relatively stagnate close to the surface creating an insulator several feet thick of poorly conducting AIR. three to six feet of air is a very good insulator. Period. This is clearly shown in the temperature gradient of atmosphere. There are many charts on the internet. This will be present with or without CO2. It has been much to long since I took advanced thermodynamics courses so someone else can calculate the mu or thermal resistance of this effect.
Uzurbrain
I caution against describing any “stationary” layer or film or clinging when it comes to describing a surface with a flowing fluid (air is also a fluid). The reason is that what you describe is a representation of reality, is a way to think about a problem, not that there is an actual stationary layer, even one molecule thick.
I had the company of a newly graduated young French engineer in Dakar during the design of a concrete cooking stove and the modeling of the equilibrium outside surface temperature was that day’s task. The engineer had been taught the most recent approach to making this calculation which was to assume that there was a stationary “layer” that was conductive only, with no convective heat transfer at all, and that the default thickness was 0.1mm. This “layer” does not exist, it is only a mathematical convenience that approximates what really happens and is, as they say, good enough for government work.
Willis’ proposed problem is interesting for a student: how literally should we take the problem description? The material is given as “concrete”. Heat flow through concrete is like what happens with a porous matrix filled with fluid. If we take it that the 1 metre cube was cut from a long 1×1 m beam, the faces would have a number of sliced stone pieces bound in a limestone matrix. The emissivity of the face is given as 0.95 (average) and that is fine, however a good student will ask what the aggregate size is. The radiation from both faces will be affected by a factor being the aggregate size divided by the length (because the heat conductive properties of the aggregate and the matrix are different). The effective length of the block will be less than one metre if the heat conduction is the average of 0.8 W /sq m/K. In reality (not in effect) the aggregate will mine heat from within the body and radiate it to space more effectively that would a homogenous material (which concrete is not).
For an engineer to ignore the difference, they should first calculate the effect of the granularity and then show that the difference is or is not significant. If it was like a hydro dam containing 76mm aggregate, the difference is probably significant given that the input power was provided to 4 significant digits and the constants are presumed to be perfect.
All gaseous molecules absorb radiation under some circumstances. For instance, N2 and O2 are actually statistically significant absorbers of radiation due to collisional perturbation at the same time as they are impinged upon by a photon. The collision perturbs their magnetic dipole, allowing them to absorb radiation, which they ordinarily wouldn’t do.
There’s a recent study somewhere online, I’ll try to find it. I think I saw it on NoTricksZone.
{ Ten Minutes Later… }
Here it is:
Scientists: Oxygen & Nitrogen ‘Radiatively Important’ Greenhouse Gases With IR Absorption Temps Similar To CO2
https://notrickszone.com/2020/02/10/scientists-oxygen-nitrogen-radiatively-important-greenhouse-gases-with-ir-absorption-temps-similar-to-co2/
Of course, any molecule which can absorb radiation must also be able to emit that radiation.. and given the predominant heat transport means of convection, a N2 or O2 molecule would likely absorb a photon, become excited in one of its available quantum states with some of that energy equipartitioned into translational energy, thus be convectively transported higher in the atmosphere, then release that energy in the form of a photon… so gases actively “pump” energy upward via convection.
And given that the mean free path length of radiation increases with altitude, this means there is more upward terrestrial LW flux than downward. There is no “greenhouse effect” in the atmosphere as the leftists claim it to be. Once again, the leftists and climate loons have turned reality on its head and gone off squawking about the end of the world, when in reality it’s exactly opposite to what they claim. I’ve noticed they tend to do that a lot.
If Earth had no “greenhouse gases”, then no gases could radiatively emit energy to space. Radiative emission to space is the *only* means by which the planet can shed energy. So Gavin Schmidt claiming the atmosphere sans “greenhouse gases” would be cold is, yet again, diametrically opposite to reality. In reality, the gases would heat up via conduction upon contacting the surface and convect upward… but they couldn’t emit that energy to space. The whole of the atmosphere would heat up, with a very small lapse rate.
The problem I have is that the AGW Crowd keeps talking about the absorption od energy in CO2 and ignoring all of the rest of the energy hitting the earth. The percentage of energy from sun in the IR spectrum is 1/10^23 of the energy spectrum emitted by the sun. Even one tenth of 10^23 times the IR energy absorbed is definitely significant.
They also ignore all of the energy from cosmic ray interactions, One source said that the typical cosmic ray impact releases more energy into the atmosphere than all of the power generated on Earth! ! The article also claims that there are hundreds of thousands cosmic ray strikes strikes per hour. What is all of that energy doing? The article was describing Cosmic Rays and had no discussion at all on “Climate Change.” Surely the location of the Earth in our Galaxy affects the number of Cosmic rays hitting us. Also the activity on the Sun also affects the magnetic field around the earth and also affects the number of cosmic rays hitting the Earth. I can hear this activity as background noise while using my Amateur Radio. There are times when this noise is more than an S9 signal. Even the noise from Jupiter rises and falls in a predictable manor. And that “Noise” is strong enough at times to operate a one transistor radio. [Radio JOVE – NASA]
Henrik Svensmark a professor at Danish Technical Univeristy has been investigating the cosmic ray formation of cloud nuclei, after he found out, that the weather changes seen in phase with the solar cycle of approx 11 years could not be explained by TSI wich only vary approx 0.1 pct. He found that the solar wind blows away the cosmic rays and in low solar activity periods it do not. So cosmic rays forms cloud nuclei, which form the clouds, which controls the temperature of the earth.
Sorry, Usurbrain, but your numbers for cosmic ray energy are far, far too high. Here’s the reality:
SOURCE
That’s the energy in a baseball dropped from about 100m (330 feet) …
w.
Oh come on, assume a spherical Earth.
Nick Schroeder wrote, “any substance capable of molecular kinetic energy… placed in the path of the spherical expanding solar photon gas at the earth’s average orbital distance will be heated per the S-B equation to an equilibrium temperature of: 1,368 W/m^2 = 394 K, 121 C, 250 F.”
From the fact that there are actually many such objects which have average temperatures much lower than that you should realize that your statement cannot possibly be correct.
For example, the average temperature of the moon (which has no atmosphere) is much lower than the average temperature of the Earth (which does have an atmosphere), even though they receive the same solar irradiance.
In fact, there is not single, predictable temperature for an object one AU from the Sun, because there are many factors which affect such an object’s temperature.
Differences between the radiation emitted by an object orbiting one AU from the Sun, and the radiation absorbed by that object, can cause the object’s temperature to vary greatly.
One obvious difference is “color.” Solar radiation is mostly much shorter wavelengths than radiation emitted by objects orbiting the Sun. An object which is has high emissivity for long wavelength radiation, but high reflectivity to the shorter wavelengths which dominate solar radiation, will end up being cooler than a similar object which has low emissivity for long wavelengths, but low reflectivity to the shorter wavelengths that dominate solar radiation.
Another difference is that radiation is absorbed by our orbiting object only on the side facing the Sun, but it is emitted in every direction. Manmade satellites are often engineered to make use of that fact. An object which is reflective on the side facing toward the Sun, but black on the side facing away from the Sun, will stay much cooler than an object of uniform color.
Another difference is rotation. Consider the Earth and its Moon. Although the Earth and Moon receive the same level of solar insolation, even if the Earth had no atmosphere, and even if its albedo was identical to that of the moon, the Earth’s average temperature would still be higher than the average temperature of the moon.
That’s because the Moon rotates only 1/27-th as fast as the Earth. That means each side of the Moon is heated by the Sun for 27 times as long as each side of the Earth is heated; and then cools for 27 times as long, as well.
That means that, even if the Earth had no atmosphere, the temperature extremes on the Moon would be much greater than on the Earth. The highs would be higher, and the lows lower.
But since radiative emissions are proportional to the 4th power of surface temperature, there would be more rapid energy loss during the Moon’s higher highs, so the difference between high temperatures on the Moon and Earth would be less than the difference between low temperatures on the Moon and Earth. In other words, the Moon’s average temperature would be lower than the Earth’s average temperature.
Nick Schroeder continued, “Like a blanket held up between a camper and campfire the atmosphere reduces the amount of solar energy heating the terrestrial system and cools the earth compared to no atmosphere.”
That’s not working very well for Venus, is it?
Psst! …. Do you do it by S = UT + 1/2 AT squared….?
Nope. Cuz U is a kluged term to combine the heat transfer resistance against radiation, conduction and convection. This problem deals only with radiant heat transfer, so all you need is emissivity.
If T1 = temp (K) at sunny side and T2 is the temp at opposite side then heat will flow by conduction to the cold side until T1=T2.
Then T2 and T2 will be 255.15K
I would say it would end up at half the sum of the temperature of space and the object that is illuminating it
I am not sure that the thermal conductivity is particularly relevant. That will just act to raise the upstream a bit and lower the downstream a bit
What is the albedo of ‘grey’?
Distance to the illuminating object would not matter?
The Sun is thousands of degrees.
Nothing gets 1/2 that hot in Sunshine, 90 something million miles away.
Nicholas
The distance is unimportant because the flux of the source is not given, just what is impinging on the concrete block, which coincidentally is the same as what arrives at the top of Earth’s atmosphere. By deduction, the distance is probably 93 million miles; however, it could be different if the source were different.
Yes, I understand the problem as stated perfectly well.
I was responding to this sentence:
“I would say it would end up at half the sum of the temperature of space and the object that is illuminating it…”
Which seems to be saying that only the temperature of the source of the incoming energy need be considered in order the known the temp.
And thank you for the reply, Clyde.
Leo
You asked, “What is the albedo of ‘grey’? Probably about the same as the moon.
Answer: the block never existed because it started at absolute zero. But let’s say it did exist at 0.01 K, then it never warmed up because it is surrounded by a perfect insulator and receives no insolation.
It is only surrounded by the perfect insulator on 4 sides.
Oh I see.
What Chair, i mean block?
Willis: What’s the absorption coefficient, alpha, of the surface of the concrete,?…
Yeah, was just gonna ask that. You can’t solve this problem without knowing how much solar irradiance is absorbed. We can assume that the absorption is constant across the incident wavelengths, but the problem should at least provide an absorption a.
Otherwise, I assume a = 0 and then it remains at the initial state.
Joe, Kirschoff’s Law says that at any given frequency, absorptivity = emissivity. So the absorption coefficient = 0.95. But you’re right, I should have specified that the absorptivity and emissivity are invariant across the spectrum … I’ll modify the head post to reflect that.
Good question,
w.
Sorry Willis, but if that was the case spacecraft would roast their electronics.
I’d put some links here but am not sure if that is allowed. Nevertheless, just google spacecraft thermal emissions and absorption materials and I am sure that you’d find examples.
I run a lot of research on this and can confirm they are different. We are getting some very nice materials (with lwoer cost ) soon to be qualified that have emissions >0.9x while their absorption is <.1, sorry can't be more specific. This is key to improving thermal radiator designs.
It’s a thought experiment, Frenchie. You are correct that in the real world, spacecraft are generally highly reflective and often insulated as well. Keeping their temperature down is an ongoing problem.
However, this thought experiment is about concrete. Low-albedo concrete has a visual light absorptivity on the order of 0.7, perhaps more depending on the surface treatment.
w.
Willis
You assumed that there was no dispersion of emissivity with wavelength, i.e. the behavior is the same with visible-light as with IR. So, we have a contradiction. If the absorptivity in the visible region is 0.7, then the albedo has to be 0.3; however, if the absorptivity is 0.95. then the albedo has to be 0.05! You can’t have it both ways unless you change the parameters of the problem by allowing the visible-light behavior to be different from the IR behavior.
Joe, Frenchie, and Willis,
If the absorptivity is 95%, then the reflectivity is 100%-95% or 5%. This is then not a grey body, but a black body, literally, with a reflectance on the order of magnitude of coal, or that mythical Arctic “Dark Water.”
Kirchhoff’s Law is a ratio, not an equality.
In Kirchhoff’s original parlance:
E/A = e
Not E=A.
In modern parlance:
Eν/αν= f(T, ν)
In other words, the ratio between emissive power and absorptivity is equal to specific intensity.
In describing his original formula, Kirchhoff wrote “emissivity”, when he meant “emissive power”. They are not the same.
Only at thermodynamic equilibrium is emissive power and absorptivity equal. If they were always equal, an object could never change temperature.
You’ll note the IPCC assumes in its equations that emissive power = 1 and thus absorptivity = 1, which is clearly unphysical. CO2 is not a hypothetical perfect blackbody.
The correct formula: h = (e (A) (σ)(Ts ^4 – Ta ^4)) / (A * ΔT)
The incorrect IPCC formula, applied to gray bodies: h = ((σ)(T^4)) / (A * ΔT)
Note the lack of e in the IPCC formula… they assume emissive power = 1 and thus absorptivity = 1 at thermal equilibrium, a hypothetical perfect blackbody.
I once owned the textbook to be able to solve this. You may have just copied one of the example problems. But I’ll be darned if I know where it is at the moment. And at least 30 minutes of good hard thought just to narrow down where to look for it.
Maybe the book is John Lienhard’s “A Heat Transfer Textbook”
I’d have to find it to tell you the author.
The chart of thermal conductivities has the formulas at the bottom of the page.
https://www.engineeringtoolbox.com/thermal-conductivity-d_429.html
Hint: Make heat budget equations for the front and back sides, each with heat gains equal to heat losses. Two equations in 2 unknowns (the temperatures).
Roy: +100
ROY the problem is the sides .. he has tried to get around them by say they are insulating but unless they don’t conduct as well they will have a gradient and they will re-radiate thru the back face anyhow. You basically end up with a soldering iron tip situation the front face and the sidewalls make up a larger area than the back face so it’s hotter than you would directly calculate.
Thanks, LdB. I specified that they are perfectly insulating. Because of that, they must also be perfectly non-conducting, because if they could conduct they couldn’t insulate perfectly …
Regards,
w.
That doesn’t stop the material re-radiating as a conduction. So let guess you are trying to use this forumla for the conduction
http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/thercond.html#c3
Note the last part of the statement
What is happening is the material re-radiates each point in the material becomes it’s own heat source. By insulating you stop the external bleed but it doesn’t stop the same thing happening internally. So I am sorry there simply is no way to remove the sidewalls from the calculation.
The only true answer to your question is a rather complicated gradient calculation.
LdB, it’s a thought experiment. Clearly your text doesn’t deal with the wonderful properties of Unobtanium … it neither conducts nor radiates energy.
However, I don’t understand your claim that “the material re-radiates each point in the material becomes its own heat source”. Are you saying that there is radiative heat transfer within the body of a solid object?
Thanks,
w.
Due to the delay these are going to end up in wrong order. However I suggest you read why you need a gradient calculation even on the conduction start by READING THE LINK.
It would appear you want the naive calculation which will pop up above this one which is make the dam thing a large flat sheet because you want to use the flat sheet approximation.
This is a bit like D..slayers not getting the whole re-radation thing, if yuou haven’t worked out the concept by now I am not sure I can help.
These walls are the equivalent of saying that the heat flow is in one direction only, which makes the problem much easier. No finite element analysis required. I’ll pull out my heat transfer book tomorrow and give it a try.
Willis if you do want the naive answer then just make it a sheet 20m x 20m sheet and you want the formula for the middle square meter so the side faces are 10m away and we can ignore the gradient. You don’t need unobtainium then either because you entitled to use the flat sheet approximation.
LdB February 28, 2020 at 9:13 am
Sure, we could do it that way, LdB, or make it 1000m x 1000m. Either your way or the Unobtanium way should give us the same answer … what answer do you get?
Thanks for the attention to detail, and best regards,
w.
Willis,
Yeah, sure, I’m misleading people. Nice!
If CSR = CHF, you would think Kirchoff, Boltzmann, and Planck would have noticed it. But no, when the walls of the cavities they inserted carbon or lamp soot stabilized (CHF=0), B & P discovered the laws of thermal emission. CSR != CHF
But no, they are dumb and you are a genius who figured it all out with your armchair physicist assertions.
Can you walk and chew gum at the same time?
Molecules can move and emit radiation at the same time, and this emission doesn’t slow them down.
“Molecules can move and emit radiation at the same time, and this emission doesn’t slow them down.”
There you violating conservation of energy again.
Greg,
“There you violating conservation of energy again.”
EM radiation is a REFLECTION of Kinetic Energy, not its SPENDING.
Molecular motion disturbs EM fields, causes radiation.
If radiation were to eliminate motion, then you couldn’t get motion at all, and thus no radiation.
I can observe you walking without you slowing down, so to speak.
Great idea, Roy. But Willis wants to include conductive heat flux, otherwise, the solution is too easy (394K).
Hot Side Radiation = Conductive Heat Flux = Cold Side Radiation
HSR => [ CHF ] => CSR
Conduction Formula: q = KA(Th-Tc)/L
Radiation Formula q = ɛσT⁴
Simplify:
Set Absorptivity = Emissivity = 1
Set K = L = A = 1
HSR = σ(Th)⁴
CSR = σ(Tc)⁴
CHF = Th-Tc
Assuming HSR = CHF = CSR:
σ(Th)⁴ = Th-Tc = σ(Tc)⁴
There is only one solution!
Th = Tc = 0
HSR = CHF = CSR = 0
Zoe:
You are demonstrating your complete inability to analyze what is really a very simple problem. You obviously have never taken even an introductory thermodynamics course, because you can’t get even the most basic analysis correct. You can’t do even trivial energy balance (1st Law) calculations.
To satisfy the 1st Law for the cube as a whole in steady-state conditions, HSR + CSR = AbsorbedSolar.
To satisfy the 1st Law for the far end of the cube in steady-state conditions, CHF = CHR.
Those are the constraints of the problem. Many of us here did this exact analysis, as we learned in the first couple of weeks of our first thermo courses. It’s not that hard.
Agreed Ed Bo
It is a first term mechanical engineering thermo problem. It introduces the concept of an albedo for the receiving and re-radiating surface, heat conduction at a given rate and an albedo for the cold side radiating into a space with no return.
So why hide the evidence?
Show it in the book.
Zoe
I am not sure what question you are asking. In order to each the elementary principles of heat transfer there are set problems with many simplifications in order to concentrate the mind on one or two factors. Another task is to calculate the equilibrium temperature of a hollow cube which is insulated and has a window of given dimensions, and a heat source inside with power [Watts].
The question posed by Willis is of this instructional type. The answer has been provided by several contributors. There are on-line calculators for such problems that have all the necessary factors included. These problem usually involve air or water flow and are much more complex than radiative problems.
Remember that the great author of heat transfer textbooks, Adrian Bejan, looked at the GHG-based global warming calculation and said that is as so simple it was not even interesting. His book on Convection Heat Transfer has problems that are extraordinarily difficult that explain things like the development of sets of thunderstorms on hot days and how convective cell structure changes with temperature, and why (meaning the physical and mathematical basis for the changes).
I can add that the temperature response of the atmosphere to an increase in CO2 concentration is very modest, and we are far better off spending our time developing “forever” energy production methods than we are worrying about ending the use of a set of declining resources. We have to have this problem solved within 200 years, 5 or 6 generations.
Zoe says: “Great idea, Roy. But Willis wants to include conductive heat flux, otherwise, the solution is too easy (394K).”
Yes, with no heat conduction through the cube, the front side is 394K. And the back side is 0K. Good work!
With NO conduction, the front is 394K and the back side is 0K.
With a TINY conduction, the front will get slightly cooler and the back will get slightly warmer.
With a bit more conduction, we get the answers provided by multiple people.
With PERFECT conduction, then the front and back are the same.
I forgot to knock off the 5% reflection and just took the 1360 W/m^2 as the flux absorbed at the front surface. Your two equations are a bit messy, but can be solved iteratively in about a dozen steps. I came up with 388.5 K at the front and 222.7 K on the cold side. Anyone who can write the correct equations can easily verify the solution without doing much work.
If you take off the 68 W/m^2 reflected from the front, it changes the front and back temperatures to 383.8 K and 221.4 K, respectively.
To Stan Robertson: I agree (383.3 K and 221.4 K).
Stefan-Boltzman, Watson, Stefan-Boltzman.
Assuming an emissivity coefficient = 1 (at steady state the block behaves as a perfect black body, according to the statement), then:
T = 393.54 °K
T = 120.4 °C
Impossible to write the equation here.
The solar absorptance of rough concrete is 0.60. The total hemispherical emittance at 300K is 0.91.Your problem statement requires the use of a gray body model with an emittance of 0.95.Your result will be meaningless.The essential feature of radiation heat transfer problems involving solar radiation is the non gray behavior of most surfaces .Check an engineering heat transfer textbook.
Will,
I reckon that the hot side to cold side temperature difference has to be large enough to transmit the 1360W. As you’re using 1square metre for the area and 1 metre for the length of transmission then the temperature difference is 1360÷0.8. Which is 1700’C
So if the cold side doesn’t heat then its
Hot = 1427’C
Cold _ – 273’C
Next I will have to work out how the block heats up which is more tricky and will take a bit of tbought
@Red94ViperRT10
Maybe John Leinhard’s excellent “A Heat Transfer Textbook” ?
Most have got the front side because it is relatively easy with SB
I suspect whatever answer you derived is actually wrong Willis because this requires a complex Integral.
The issue is the graphic and question text Willis misleads there are 5 cold sides not 1 🙂
The back side if you ignored the actual 4 sides would be straight forward it is just thermal conductivity thru 1 square meter . The sides create the problem they get progressively colder the further they are away from the hot face creating a gradient along them. The front face to back face conduction is what 98 watts/sec so the side face re-radiation are also going to be significant. Each of the hotter gradient along the sides will also radiate out the back face because it is the furthest away and hence coldest.
So my question is do you really want the hard answer or were you trying to do the naive answer ignoring the sides?
I should say adding a perfect insulator around then sides does not simplify the problem they represent because they conduct. As I stated to Roy you have made a soldering iron tip and it’s a little more difficult than the naive answer.
“The front face to back face conduction is what 98 watts/sec ”
I have a general rule that when people get the units wrong , they probably don’t understand the physics.
So do I. So either you don’t get why it has to have a time component or you don’t get the difference between say KW and KWh.
Firstly it’s kWh not KWh. Second you have the equivalent of kW/h not kWh, I rest my case.
ROFL
In case you are wondering why he is ROFLing…”The kilowatt-hour is a composite unit of energy equal to one kilowatt (kW) of power sustained for one hour. ” in other words kW per hour or kW/h. Even I knew that one.
He was trying to play like some do with English and spelling, that they are more superior. We have a non flattering word for him in the industry, its the old case of those who can’t teach.
Units:
“… in other words kW per hour or kW/h”
The Watt has a time component so there are never W/hr (Watts per hour) in any form.
kWH, often written KWH is one thousand Joules per second for an hour. 1 kWH is 3.6m Joules of energy moved or dissipated or released in the course of one hour.
Any number of Joules per second divided by 3.6 million = kWH which converts the energy component (Joules) and time component (seconds) into kJ and Hr.
Always some to WUWT – learn or teach something every day without finger pointing.
” … The sides create the problem they get progressively colder the further they are away from the hot face creating a gradient along them. …”
—
LDB, the entire block is going to have the same gradient, illuminated side to shadowed side. I don’t know why you’re getting snarled-up on a side-issue. 😉
Hot 125C
Cold -145C
One comment, which may or may not be helpful:
The only part of the block that matters is the face.
The other three sides receive nothing and radiate nothing.
So rather than being 1m deep, it could be infinitely thin.
The only difference that would make is the length of time taken to reach steady state.
Radiation in = radiation out.
What temperature does an object with a known emissivity have to be to emit X watts/m^2
A block has 6 sides
Group problem solving in history.
So correlation IS causation, exactly as AGW hypothesises, and King Arthur proves via witch = duck deduction.
Take that, den1ers!
emissivity of .95, with constant influx of heat on one side (1360 should eventually heat the core to critical temp of planet explosion right ? Blowing out the sunny side. leaving a much cooler half meter thick block which will then start reheating until in half the time cause the reduced thickness remaining block to once again blow off the heated side. ad infinitum eventually exfoliating the block to a thin post card of concrete. cause the ocean stole the heat. and stored it at 4 degree above freezing in the deep.
Alternative answer: The stray CO2 molecule that arrived on the heated face super heated the concrete block driving it to critical temp near infinite Kelvin and it totally vaporized.
Wait the heated side caused the block to start rotating eventually reaching overspin condition and threw off a large mass of material from the prior heated side forming a smaller less dense satellite which began orbiting its reduced spinning source and they lived happily ever after, until the smaller satellite got its faced locked toward the block remanent.
Or something like that.
Willis
Sorry spell checker got your name last time
I went off on a tangent mentally there. The 0.95 emissivity gives the answer to the cold side. So the temperature difference cold side to Absolute Zero has to be
1431’C using the same calculation as before
So my bizarre answer is
Hot = 3131’K
Cold=1431’K
But most likely wildly incorrect
I look forward to seeing the solution
Simple. Use Stefan-Boltzmann equation to compute temperature for given energy flux and albedo.
Then apply Fourier’s Law (not to be confused with Fourier Series, but Fourier had to discover the series to solve the equation) to compute heat conducted through the cube with the given dimesions and thermal conductivity.
I’ll leave the details and final result as an exercise for you students. :-]
120C
44C
Typo, that is 4.4 C
Oh, conductivity is w/m.k not w/m/k
firstly watt = W , not w ; kelvin is K not k .
secondly W/(m.K) is identical to W/m/K , whoever gave you w/m.k is wrong on three counts.
The formula for conductivity is QL/A deltaT. This tells you the units. Wm/m^2K.
W/mK.
If you divide m by K you end up flipping K to become WK/m.
“w/m.k…”
W/m•K is correct.
The • character is produced by holding the Alt key down and typing 0149 on the number pad.
Or full stop when written. It replaces the ‘x’ symbol for multiplication of course, to avoid confusion with variables called ‘x’.
I spent many years studying and working in engineering (Mechanical, also Thermodynamics and fluid dynamics). it is watts / (meter x temperature).
And yes, James Watt was the inventor of the steam engine, I am fully aware they symbol for power and temperature are in upper case.
I also speak French, and m well aware of the use of the alt+numeric keypad number to generate characters form the ASCII table, alt+132 for example.
In fact I am am now a software engineer, I am fully aware of the ASCII table in it’s entirety.
In software it would be W/m*K. The asterisk is used for multiplication in software calculations.
‘^’ is used for the power of, so it can also be written: W*m^-1*K^-1 if you understand software notation.
In fact I know rather a lot about all of this sort of stuff.
Using W/m*K for conductivity is ambiguous at best, but actually wrong.
The rules of algebraic precedence have multiplication and division at the same level of precedence, with operations from left to right. So in that format, it is equivalent to (W/m)*K, and the K ends up in the numerator, which is wrong.
W/m/K is correct, as is W/(m*K).
Operator precedence in the C language is hardly relevant.
10/10/10 is not the same as 10/ 10 x 10
I was referring to the standard algebraic rules of precedence, which have been followed for centuries. There is a reason all serious programming languages follow them.
You say: “10/10/10 is not the same as 10/ 10 x 10”
My point exactly. “W/m*K” is not the same as “W/m/K”. The second is correct. The “K” term must “end up” in the denominator.
Don’t have time to work this out, but as the block warms up the energy absorbed will decrease. It is proportional to the difference of the 4th powers of the emitting and receiving surfaces (i.e. sun, block) per S-B. I think we can assume a constant sun surface temperature. I suspect Willis intended his hypothetical block to have a 0.95 absorbtivity as well as a 0.95 emissivity.
Also, Dr, Spencer is correct, I think, that it requires solving two simultaneous equations.
389K and 327K, as long as there no gas inside conducting heat from the front to the back.
Oh it’s a solid block! silly me.
Once the block is in equilibrium, the hot and cold side would be the same temperature.
The perfect insulator guarantees this, so long as the conductivity is greater than zero.
Correction. I thought the cube was insulated on 5 sides.
correction to correction. Got the same answer using 4 sided insulation, by treating block as a part of much larger sphere. In the absence of a greenhouse effect, the material is predicted to be isothermal.
Fred:
Like many, you are confusing static equilibrium with dynamic steady-state conditions. This problem is clearly the second case.
The sun-facing side is (net) absorbing power, and the back side is (net) outputting power. So there will be a temperature difference between the two ends that leads to ongoing conduction.
First, kirchoffs law states that absorbtivity equals emissivity. There fore, use boltzman to calculate the absorbed surface energy.
The use boltzman to calculate the required surface temperature to emit that energy flux, assuming radiation to a 0 degree sink. That will give you Tc of the concrete.
Next apply fourier’s law of conductivity to determine the temperature gradient across the block of concrete (conduction coefficient provided by Willis). That will give you the temperature on the hot side.
This neglects surface back radiation to other cold sinks that may be available on the hot side of the block because that side will emit as well unless there is no “visible” low temperature sink.
Sorry gents, you misunderstand kirchoff’s law, maybe better is you are misapplying it. Google the sort of bible of material properaties, nasa ref pub 1121, dated april1984, “Solar absorption and thermal emittance of some common spacecraft thermal coatings.”
It is not the most up-to-date complete list, but it is a good reference to begin with.
Needless to say, we design many materials to have quite differnt a and e.
@Frenchie77
No, it appears you do not understand that Kirchoff’s Law states that absorption and emittance are equal at the same wavelength.
Pub 1121 defines ‘a’ and ‘e’ at different wavelengths (i.e. black-body temperatures): absorption => 5800K and emittance => 300K. In other words, sunlight is primarilly absorbed at wavelengths less than 1 micron, but emitted from 5 to 35 microns.
Well, do you think that the concrete is going to get as hot as the sun? Hence, why I think you are misapplying it here.
125.46 C Hot
3.27 C Cold
Is it anywhere near a black hole?
Hi Willis,
To solve this problem you need to know both the optical absorbance and the infrared emissivity.
Thanks, Steve. Another commenter pointed this out. I’d just assumed 0.95 for both, but I hadn’t specified that. I’ve modified the head post to clarify that.
w.
You can’t all be right but you CAN all be wrong.
Is this problem something to do with why no one makes concrete frying pans? Otherwise while I am sure it is fascinating as an academic exercise I will stick to my iron, non-Teflon coated, steak pan.
In other news, the Chinese lunar explorer fried an egg on the surface of the moon.
The wanted sunny side up, but it wound up scrambled.
Your cube is completely possible as a model of the Eartha atmosphere without GHG, because the ‘sides’ join each other in a sphere. You don’t need unobtainium.
And in an atmosphere without GHG (or circulation) the temperature is isothermal.
So the hot and cold side must be the same temperature. So my original answer was right, but for the wrong reason.
He could have a front and a back fixed apart with poles at the corners and with no sides at all, that saves obtaining the unobtainium.
is that true on mercury?
No it’s the same as the earths crust, is the surface the same temperature as the core?
Simple students problem from 2nd course.
Why?
Alex February 28, 2020 at 8:47 am
True. Why? Three reasons.
1) For fun, and
2) For folks who are interested in such matters but haven’t taken the “2nd course”, and
3) For Zoe Phin.
Zoe has a blog where in my opinion she’s misleading lots of folks. I couldn’t get through to her, and I hate to see folks on the skeptical side putting out incorrect information. It doesn’t do scientific scepticism any good when that happens.
So I thought that if folks here worked out and discussed the issues in public it might help her see that her theories are incorrect. In particular, she thinks that at steady-state, the energy flux passing through a solid object is different than the flux going out of the far end of the object … me, I say that’s not possible.
Best regards,
w.
“steady-state” is what’s not possible.
Kurt, I don’t understand this. After some period of equilibration, the concrete block will be neither warming nor cooling, i.e. “steady state”. Why do you say that’s not possible?
w.
The ISS and most satellites seem to managed it but apparently it is impossible because Kurt says so.
Hey Willis, there is no helping Zoe. She’s off into flat-earther mentality. The rest of the world is mad and only here little clique of fans” seem to be able “understand” this amazing discovery. This thread has its own merits but don’t imagine it will help Zoe, she’s needs to be special and ain’t going to give it up.
I explained where she mistakenly assumes that the “cold end” of her bar is cooled by emission simply because it is not specified. That is her fundamental mistake. She avoids addressing that. I explained that her idea the you can have “two different fluxes” at a surface violates conservation of energy. She can’t deal with that either.
She is convinced that a flow of 2.5W/m^2 in the bar can lead to 557W/m^2 at the other end. I suggested she patent the technique. If I can have over 1kW of space heating from 5W of electrical input, I’ll pay good money for one those concrete bars.
Greg February 28, 2020 at 10:33 am
Jim, I truly don’t know what Zoe can and can’t learn, and it is my practice to do my best to avoid hindering someone with my thoughts. Heck, in my youth I used to believe that Chairman Mao Tse-Tung was one of the good guys, and I got over that, so anything’s possible …
Next, I suspect that many readers of her blog also read WUWT. So although she may or may not change her mind, those readers might do so. In particular, she claims that a geothermal heat flux on the order of a few tenths of a watt per square metre is keeping the surface at ~ 15°C … and I hate to see people walk away foolish thinking that that is true.
Best regards,
w.
Willis, I also like to start from your generous view of someone. My current view comes from trying patiently to explain to Zoe where she went wrong and her stubborn refusal to engage in a logical argument to arrive at a commonly agreed position.
She seems very young and I expect it will take her about the same length of time to work this one out as it took you with the Mao thing.
It’s a fair point that this may be enlightening to others, that is also why I put some time into her blog trying to straighten it out. I final realised I was peeing into the wind.
Hi Willis!
It would be nice to see a new quest:
1. You have a ball shape size like earth, with no condutivity recieving an energy radiation of 1361w/m2, albedo = 0,3 – but now rotating with a speed of 360degrees/24hours. T-hot and T-cold?
2. Same as 1. but now divided into 3 zones. Zone a= +-30degrees longtude, zone b=+-60degrees logitude – zone a and zone c = +-90 degrees logitude – zone a – zone b
kind regards
SteenR
Your “perfect insulator” is just a perfect mirror.
The solution is straightforward.
The transverse size of the block does not matter.
We have incident Power flux on the front surface.
There is a temperature Tf established at this surface.
The front surface radiates a power flux according to the Stefan-boltzmann.
The back surface equilibrates at temperature Tb.
So, there is power flux inside the block due to the temperature gradient.
The back surface also radiates due to the Stefan-boltzmann.
We have two temperatures to find and two equations: total power fluxes at the both surfaces must be zero.
The system is well defined and solvable.
Will not work out numbers.
Too trivial.
Alex, certainly it may be “too trivial” for you. And I’m sure that for some folks, problems that you find very difficult are “too trivial” for them.
Me, I write for a mythical person that I call the “interested layman” … and as the number of different answers proposed here shows, the problem is not “too trivial” for many folks reading this, whether they are in that category or not.
Finally, I may be wrong myself, which is always worth writing about … but nobody so far has gotten the answer that I get. They are close to my answer, but not exactly the same, which is curious in itself.
Perhaps you could unbend a bit and give us your answer, so we can see how it should be done and whether I’m wrong or not.
Thanks,
w.
Willis,
I agree,.
Not actually solving the problem does not win the cookie.
Anyone can say “I know how to do it but it is too easy to bother with.”
Personally, I do not know the equations for solving this, and there is no point in me looking them up because other people here know much more about this than me.
But I am struck by how many people here claim to know exactly how to do this exercise and yet disagree with each other.
I do have a question…maybe I overlooked something.
Do you not need to know the specific heat of concrete to be able to say how hot it gets and thus how much it radiates from the sunny side of the block?
Or maybe that cancels out by the time thermal equilibrium is reached?
But what if it is rotating?
Nicholas
You said, “But I am struck by how many people here claim to know exactly how to do this exercise and yet disagree with each other.” Yes, I’m also struck by the fact that there is often disagreement over what seems to be basic science or engineering, between people who claim or act as though they experts in the field. I’d be happier if there was more “consensus” on basic physics calculations.
Alex February 28, 2020 at 8:59 am
The system is well defined and solvable.
There’s a very funny story about an Engineer, a Physicist, a Mathematician and a midnight fire in the bathroom wastebasket.
“A solution exists.”
w.
Alex
Yes, if the Unobtainium has an absorptivity of zero, and hence an emissivity of zero, [at all wavelengths] then you have your “perfect mirror” with a reflectivity of 100%. Your can get that with a material with a complex refractive index with a very high real index of refraction (n>>10) and an imaginary (as in sq rt of -1) extinction coefficient approaching infinity. With nothing in empty space to conduct to, all the internal energy has to be confined to the channel between the front and back, and can therefore only exit by radiation.
60.2ºC for both
Divide incoming by 2
I would post the correct answer and the associated math behind the numbers.. but I don’t want to appear superior, so I won’t. Good Luck!
1what would happen if you replaced the earths atmosphere with a perfectly transparent material 1 meter thick?
Sunlight would warm the surface of the earth, and the earth would warm the transparent material. The question now becomes, is there a difference in temperature between the inside and outside of the transparent material.
If there iis a difference how does one explain the prediction of an isothermal atmosphere. Inn the absence of GHG?
Using Stefan-Boltzmann T-hot = 398.8K
If the thermal conductivity “k” of the concrete is equal to 0.8 watts per metre per kelvin (0.8 W/m K^-1) then the T-cold side must be finally emitting 0.8×398.6 W/m2 which is 318.9W/m2.
Put that 318.9W/m2 back into the S-B equation with the same emissivity of concrete and T-cold = 277.4K
leitmotif
You appear to be touching on an essential part of the problem that is not well defined. If the rate of conduction is less than the rate of arrival, then the surface facing the source of energy will continue to heat, irregardless of the emissivity of the face radiating. This strikes me as being similar to the classic differential calculus mixing problems where one needs to know most of the variables. Conductivity seems to be a key parameter here. If the thermal conductivity is too small, it becomes a ‘bottleneck’ and the front surface will continue to heat, while the back surface reaches an equilibrium determined by the conductivity and emitting flux. I’m not sure that the “Thought Problem” is adequately posed to calculate the result.
Assume a Tcold for the unlighted side.
Calculate how much heat is radiated to space from Tcold. 0.95*5.67e-8*Tc^4
Call this Qrcold
This amount of heat had to be conducted through the concrete from Thot
So by heat conduction from hot to cold (Thot-Tcold)*0.8 is equal to Qrcold….
So your calculated Thot is Tcold+(Qrcold/.8)
Calculate how much heat is radiated to space from Thot …..0.95*5.67e-8*Th^4
Call this Qrhot
Qtotal =Qrhot+Qrcold
Select new Tcold until Qtotal is 1360
Answer…I got Tcold=222.7 Kelvin, Thot=388.3 Kelvin
This assumes the “albedo” of the concrete is 0 in keeping with Willis’s definition of the problem. Actual albedo of concrete is about 0.5, which makes about 15 degrees difference.
This problem is equivalent to the infinite sheet problem, where the sheet is 1 m thick (see Carslaw and Jaeger, or Crank). Since it’s steady state, the solution for the temperature profile has a zero time derivative. That solution will have linear dependence on T with respect to depth within the sheet. So the solution will be one that satisfies the boundary conditions. On the hot side, you have a flux in (from the sun), a flux out (S-B thermal radiation) and a flux through to the cold side. These have to sum to zero (taking direction into account). The flux through will be determined by the temperature difference between hot and cold and the thermal conductivity. On the cold side, there is flux from the hot side and flux out to space (I’m neglecting leftover radiation from the big bang, but if you like, you could factor that in). The 2 fluxes on the cold have to sum to zero. I haven’t done the calculation, but it should be straightforward.
Of course, the above assumes that the thermal conductivity is temperature independent (often not true) and it assumes that the block is totally opaque (also sometimes not true at some wavelengths), so there is the possibility of reality biting one in a “fundamental” way.
Plugging in the numbers and assuming constancy of heat conduction, no big bang, epsilon emit = epsilon absorb) etc., I get T(hot) = 383.3 K (110.1C) and T(cold) = 221.4 K (-51.7C).
Thanks, Michi. You’re the first person to get the exact answer I got (see here), so at least we’re either both right or both wrong …
w.
That makes a bunch of us including Roy if you take Glen’s comment as being correct.
As a sanity check, I set the emission/absorption at 1. The hot side was 383.7 K and the cold side was 219.4.
Indeed, Bob, many folks have gotten the correct answer.
Regards,
w.
Yes Willis. As I write this there are 453 comments on your story. The number of comments that show a more-than-rudimentary understanding of thermodynamics is truly impressive. Is there an alarmist blog that could muster such a performance?
MichiCanuck
You assumed “constancy of heat conduction.” Is that reasonable? I think that the rate of conduction would be driven by the temperature differential between the two faces. It further assumes that the material is capable of any rate of conduction.
It’s unlikely to be realistic, but it’s a necessary assumption given the problem as stated. For many materials, some of the heat conduction is actually via radiation across internal voids or because the material isn’t perfectly opaque at important wavelengths. I remember hearing an interesting talk a long time ago about how important blocking IR radiation was to making effective fabrics for cold weather survival suits. Once blackbody radiation enters the picture, non-linearity rears its ugly head. The problem’s still soluble, but the temperature profile across the block/sheet would no longer be linear. You’d also have to use numerical methods to obtain a solution to even the steady state equation.
One dramatic effect that always impresses me about a temperature dependent parameter is electrical conductivity in metals. If you’ve ever seen an RF coil used to heat up a metal bar, you’ll have seen that it heats very slowly at first. That’s because at low temperature, its conductivity is high and Ohmic heating isn’t very effective. However, as temperature climbs, resistance in the metal increases and heating speeds up. Eventually, it only reaches steady state when heat losses equal the absorbed RF energy. In air, conduction and convection can be important. In a vacuum, it’s good old black body radiation that has to do the cooling, which isn’t all that effective until things get quite toasty.
h=temp hot surface
c=temp cold
s=stefan’s constant
(1)Incident radiation=.95×1360 (some reflected)
(2)Emitted rad hot=.95sh^4
(3)Emitted rad cold=.95sc^4
(4)Conducted heat=k(h-c)/1 {temp gradient 1m}
Balance at 2 surfaces
(1)=(2)+(4) at hot
(3)=(4) at cold
Solve numerically: 2 equations in 2 variables h and c – ignore complex and negative temps 🙂
I was going to post the same thing but you got in first. Exactly right and easier to solve numerically than exactly. Guess C, use equn (3)=(4) to calculate H and then use H to to calculate (1)-(2)-(4). Adjust C until (1)-(2)-(4) equals zero. A couple of minutes in excell
Nice exercise Willis. I get Thot = 383.3 K, Tcold = 221.4 K
Work in Excel shown here in a screenshot with formulas written out. As Roy Spencer notes simulaneous equations are used.
Qh and Qc are watts out for the hot face and cold face.
Procedure: Enter trial Th, calculate Qh out via S-B, calculate initial Qc out by conservation of energy, calculate initial Tc via S-B, calculate resulting Qc out by conduction and iterate trial values of Th until the two Qc values converge.
Instead of solving it algebraically, I programmed the heat budget equations for the front and back surfaces in Excel. I whipped it up quickly, but I get 388.52K for the front surface and 222.74K for the back surface. I had to specify a heat capacity (I used granite), but the final answer does not depend on that, the heat capacity only affects the time it takes for the front and back surfaces to come into thermal equilibrium (with granite it took over 30 days to approach equilibrium).
Thanks, Dr. Roy. Kudos, by the way, to all who solved it iteratively in Excel. I use the “Solver” function in Excel for lots of these kinds of problems, it works like magic.
w.
Here’s an R solution if Word Press doesn’t chew up the code:
epsilon = 0.95
sigma = 5.670374419e-8
R = 1360
k = 0.8
err = function(T.hot){
T.cold.1 = (R / epsilon / sigma – T.hot ^ 4) ^ (1/4)
T.cold.2 = T.hot + (epsilon * sigma * T.hot ^ 4 – R) / k
(T.cold.1 – T.cold.2) ^ 2
}
T.hot = nlm(err, 389)$estimate
T.cold = T.hot + (epsilon * sigma * T.hot ^ 4 – R) / k
Joe, always good to hear from you. Thanks for the sweet R code, always more for me to learn.
w.
Roy,
Do you think Boltzmann and Planck were dummies?
Why didn’t they get this solution?
I would accept either if you use flat sheet laminar flow and just to be clear because Zoe seems messed up on this there is a gradient thru the block.
Dr. Spencer,
The temperatures you posted indicate no correction of incoming heat duty from 1360 watts to 1292 watts with 0.95 emittance. If you correct to 1292 watts, you should get the 383.3 K and 221.4 K temperatures that David posted above.
I was going by Willis’ original post, which suggested the heated side was absorbing 1360 W/m2.
I made hot side 385.5K and cold side 222K
At the hot side, at 389 K, there is 1227 W/m2 of radiation to space and 133 W/m2 of conduction to the cold side of the cube, at 223 K, from which there is 133 W/m2 of radiation to space.
Kind Regatds
Assuming there is no matter past the cold end, the final temperature of both sides will be equal.
Ah, the old “radiation needs to know where it will land before it leaves” hypothesis. You obviously are ready with an explanation of how radiation emitted 13 billion year ago “knew” it was going to find Hubble space telescope before it left home.
Please lets us know how that works.
‘the old “radiation needs to know where it will land before it leaves” hypothesis’
Yes, very one Boltzmann and Planck used to derive their laws.
Tell me the new junk science that can’t derive them.
http://hyperphysics.phy-astr.gsu.edu/hbase/mod6.html
Please tell me which scientists originated what you claim, and why did you believe him?
So how does light from 13bn years ago “know” it is going to “resonate” with HST before leaving home? You never answer a question. You always divert with a different question.
Greg,
The space between the EM tethered objects got stretched, if you believe in the big bang.
There’s no EM rope trying to find an end. All was tied up in the beginning, then got stretched.
Zoe is right.
It is a popular misconception that light leaves A at time zero and arrives at B at times 1. Even calling c the “speed” of light is an inaccurate colloquialism. Light travels at the speed of – well – light, and thus experiences no time.
Light is outside of time. An EM photon does not experience the passing of time.
Take Betelgeuse, 700 light years away. Did light leave Betelgeuse 700 years ago? No. Light took no time at all to get from there to here. But time over at Betelgeuse is 700 years different from here.
It’s hard to get your head around, but this is correct cosmology and a full understanding of what Einstein illucidated. Time and space are one. The “light year” is actually a very good term since the distance between earth and Betelgeuse can just as well be described as 700 years as a number of meters.
Sorry but it’s just WUWT old boy science to talk about light taking time to travel a distance. Light experiences no time so it takes no time for get anywhere. As Matt O’Dowd of PBS SpaceTime YouTube channel explains very nicely, the speed of light is not even about light. It’s the speed of causality.
https://youtu.be/msVuCEs8Ydo
Phil Salmon
So in Willis’ example if the universe the sun and block are in is curved in such a way that light from the sun is on one side 93,000,000 miles away and on the other side 13 billion light years away in Zoe’s system when we place the block in its position when does the far side of the cube start to cool or radiate, instantaneously or after 13 Billion years?
Phil Salmon wrote:
“Light experiences no time so it takes no time for get anywhere.”
That’s so wrong that “wrong” is the wrong word to describe how wrong it is. LOL
First, we’ll explore what a photon “is”.
A photon is the interaction of the electronic and magnetic fields, oscillating in quadrature, geometrically transformed into a spiral (because a sinusoid is a circular function and a circular function spread axially over space-time is a spiral). When we see electromagnetic energy as a sinusoid on our oscilloscopes, we’re literally looking at a shadow of reality, because we don’t have 3-D oscilloscopes, and the oscilloscope only looks at the electronic field, not the magnetic field.
The photon, being massless and circularly polarized when considered singularly, doesn’t carry its energy in its linear momentum, it carries it in its angular momentum.
http://staff.washington.edu/bradleyb/spiralsynth/fig3.1.gif
This is why, when traveling through transparent mediums of differing refractive indexes, the photon energy does not change, while the apparent photon speed does. If its energy were carried in its linear momentum, photon energy would necessarily change as it transited different mediums.
E^2 = p^2 c^2 + m^2 c^4
pc is the magnitude of the momentum vector. Since c is fixed in vacuum, p must change for the photon’s energy to change.
p=ħk
where k is the wave vector (where the wave number k = |k| = 2π/λ), ω = 2πν is the angular frequency, and ħ = h/2π is the reduced Planck constant.
The energy and momentum of a photon depend only on its frequency (ν) or inversely, its wavelength (λ):
E= ħν = hc/λ
This is why Planck’s constant has units of angular momentum (J-s), and the reduced Planck constant represents the quantum of angular momentum.
———-
The photon itself (if it is traveling at c, which it may not necessarily do… it is dependent upon the medium it is transiting. c is for vacuum.) _experiences_ no time, which is why it is persistent and doesn’t entropy into the background zero-point quantum vacuum field (at least, until after it impinges upon invariant-mass matter or experiences a sufficient gravitational field (because light doesn’t travel in a straight line, it follows the path of least time, and gravity is a manifestation of the warpage of space-time, which is why light ‘bends around’ large celestial objects, because invariant-mass matter expands the surrounding space-time and slows down time.).
But just because the photon _experiences_ no time doesn’t mean no time has _elapsed_.
Only for entities at c would the photon seem to not experience any time… for objects at anything less than c, that photon does indeed take time to traverse space. It’s called the theory of *Relativity* for a reason.
If “Light… takes no time for get anywhere.”, then causality is irreparably broken.
Light travels at 299792458 m/s in vacuum… it transits 299792458 meters for every second in our frame of reference.
Now we have to describe what we mean by ‘vacuum’…
hard vacuum (no invariant-mass matter, quantum vacuum zero point field exists)
perfect vacuum (no invariant-mass matter, no QVZP field)
In fact, it is the quantum vacuum zero point field which is the medium which limits the speed of light. In a hypothetical perfect vacuum (which cannot exist, because the metastability of invariant-mass matter is dependent upon the existence of the QVZP field, thus we could never construct a machine capable of a perfect vacuum without the atoms / molecules of that machine’s vacuum-facing components undergoing beta capture and thus transmutation [1][2][3][4]), there would be no speed limit except for the energy field the photon itself lent to the perfect vacuum in transiting that vacuum.
[1] https://journals.aps.org/prd/abstract/10.1103/PhysRevD.11.790
[2] https://web.archive.org/web/20190713220130/https://arxiv.org/ftp/quant-ph/papers/0106/0106097.pdf
[3] https://web.archive.org/web/20190713225420/https://www.researchgate.net/publication/13330878_Ground_state_of_hydrogen_as_a_zero-point-fluctuation-determined_state
“We show here that, within the stochastic electrodynamic formulation and at the level of Bohr theory, the ground state of the hydrogen atom can be precisely defined as resulting from a dynamic equilibrium between radiation emitted due to acceleration of the electron in its ground-state orbit and radiation absorbed from zero-point fluctuations of the background vacuum electromagnetic field, thereby resolving the issue of radiative collapse of the Bohr atom.”
[4] https://web.archive.org/web/20180719194558/https://ntrs.nasa.gov/archive/nasa/casi.ntrs.nasa.gov/20150006842.pdf
“The energy level of the electron is a function of its potential energy and kinetic energy. Does this mean that the energy of the quantum vacuum integral needs to be added to the treatment of the captured electron as another potential function, or is the energy of the quantum vacuum somehow responsible for establishing the energy level of the ‘orbiting’ electron? The only view to take that adheres to the observations would be the latter perspective, as the former perspective would make predictions that do not agree with observation.”
LOL this I have to hear.
So how does the emission leaving the cold end know ahead of time there is matter out there somewhere?
Does it send out some special faster than light communication asking for all matter to respond?
Or perhaps it just omipotent know?
The ISS is up there right now and I am seriously wondering why it isn’t all the same temperature given your statement.
Zoe: It might help to use the form of S-B:
Qrad = e A sigma (T1^4-T2^4)
(In Willis’ problem A =1 , e = 0.95 , Sigma = 5.67 E-8)
Where T1 is the warmer T2 is the colder surface temperature.
You will find that T1= 398.59 K and T2 = 0, you get 1360 watts/m2
With T1 = 398.59 and T2 = 388.5 you get 132.5 watts/m^2, the heat Flux to the hot side of the cube.
With T1 = 222.7 and T2 = 4 you get 132.5 watts/m^2, the heat Flux from the cold side of the cube to space.
And 0.8 * (388.5 – 222.7) = 132.5 watts/m^2, the heat Flux from the warm side to the cold side.
So the temperatures are all reasonable and the 3 heat flows are equal as required for a system in thermal equilibrium. I think this exercise has been a good demonstration that being an excellent computer programmer is not enough to get correct answers. You must also understand the problem and apply the correct solution method.
Rick,
You missed the part where 90% of energy from the source was rejected, while pretending absorptivity is 0.95.
You can’t solve HSR = CHF = CSR
So you solve the cold end, CSR = CHF, and ignore HSR = CHF or you reject 90% of incoming HSR to get your equality, but then your Thot is fake.
Zoe: No, you missed the basics of thermodynamics. Everything with a temperature above absolute zero (which is literally ‘everything’) will radiate energy. If you have two objects at different temperatures, there will be a NET heat flux from the warmer to the colder. The 90% of the heat you’re talking about is not rejected, it is absorbed raising the surface temperature of the block from 0 to 388 K which results in 90% 0f the heat absorbed being radiated back out to space. The net rate of heat flow will be proportional to the difference in the 4th powers of each object’s absolute temperature. Thus, a 1000 C radiant source surface can heat a 975 C surface, but not very much. But both surfaces would radiate a lot of heat to a cold object. They don’t stop radiating energy when you position them to face each other. However, the same 1000 C source will heat a 20 C surface by 100’s of degrees. This is really basic thermodynamics stuff. Take a course – there are lots of them.
Rick, you should just ignore Zoe. She/he is just prankster blogger.
Correct DMac, I thought she was serious and just being dense but yep I worked that out as well.
Unfortunately, I fear that she truly and passionately believes what she’s saying. That’s the scary part. Go to her website and read some of the many pages. It’s far too large, complex, detailed, and too much work to be just a prank.
And sadly, people believe her. I was directed to her site by a very enthusiastic friend who claimed that she was revealing amazing stuff …
In large part, that’s why I wrote this—in the hopes that fewer people would be fooled. In that, I think I’ve been fairly successful because she’s revealed her contradictions and lack of understanding in very clear terms.
Regards,
w.
OK. At this point, I’ll give my answer, and indeed, I may be wrong.
Also, it seems some folks are using slightly different assumptions than I used, my bad, my lack of clarity in setting out the puzzle. So I’ll try to cover all of those.
We have two conditions that must be met at steady-state. First, the amount of energy entering the block must be equal to the amount of energy leaving the block. The amount entering is equal to 1360 * epsilon, which I’ve said is the emissivity (and thus the absorptivity) at all frequencies. The amount leaving the block is equal to sigma epsilon (T_hot^4 +T_cold^4). So the first equation is:
sigma epsilon (T_hot^4 + T_cold^4) == 1360 epsilon [eqn1]
The second condition at steady-state is that the flow through the block has to be equal to the flow out of the cold side. The flow through the block is k (T_hot – T_cold), and the cold side radiation is sigma epsilon T_cold ^4, so the second equation is:
sigma epsilon T_cold^4 == k (T_hot – T_cold) [eqn2]
Me, I use Mathematica for this kind of problem. This avoids me making stupid math errors. I’m good at abstract math, but I’ve been known to not be able to remember that 8 * 7 = 54 … just kidding, put down the mouse …
I’ve used “Th” and “Tc” for the hot and cold side temperatures. Here’s the Mathematica solution, with “In[N] being inputs and “Out[N] being the Mathematica outputs:
In[43]:= Clear["Global`*"] In[44]:= eqn1 = sigma epsilon (Th^4 + Tc^4) == 1360 epsilon Out[44]= epsilon sigma (Tc^4 + Th^4) == 1360 epsilon In[45]:= eqn2 = sigma epsilon Tc^4 == k (Th - Tc) Out[45]= epsilon sigma Tc^4 == k (-Tc + Th) In[46]:= sigma = 5.67 * 10^-8 k = .8 epsilon = .95 Out[46]= 5.67*10^-8 Out[47]= 0.8 Out[48]= 0.95 In[49]:= Last[Solve[{eqn1, eqn2}, {Tc, Th}]] Out[49]= {Th -> 383., Tc -> 221.}So, 383 K for the hot side, and 221 K for the cold side. Note that because of the fourth power in the terms, there are a number of either physically impossible (negative temperatures) or imaginary number solutions to the problem. Here is the full list of all the solutions:
{{Th -> -428. - 12.2 I, Tc -> 201. + 245. I}, {Th -> -428. + 12.2 I, Tc -> 201. - 245. I}, {Th -> -406. - 8.55 I, Tc -> -201. + 145. I}, {Th -> -406. + 8.55 I, Tc -> -201. - 145. I}, {Th -> 10.7 - 389. I, Tc -> -65. - 220. I}, {Th -> 10.7 + 389. I, Tc -> -65. + 220. I}, {Th -> 14.7 - 407. I, Tc -> 226. - 142. I}, {Th -> 14.7 + 407. I, Tc -> 226. + 142. I}, {Th -> 31.4 - 376. I, Tc -> -293. + 70.3 I}, {Th -> 31.4 + 376. I, Tc -> -293. - 70.3 I}, {Th -> 39.3 - 405. I, Tc -> 132. + 291. I}, {Th -> 39.3 + 405. I, Tc -> 132. - 291. I}, {Th -> 345., Tc -> -315.}, {Th -> 373. - 18.5 I, Tc -> 46.7 - 277. I}, {Th -> 373. + 18.5 I, Tc -> 46.7 + 277. I}, {Th -> 383., Tc -> 221.}}Mathematica puts the positive real-number solution last, so I’ve just shown that one.
If it is a perfect blackbody, on the other hand, then epsilon = 1 at all frequencies. In that case we get:
In[50]:= Clear["Global`*"] In[51]:= eqn1 = sigma epsilon (Th^4 + Tc^4) == 1360 epsilon Out[51]= epsilon sigma (Tc^4 + Th^4) == 1360 epsilon In[52]:= eqn2 = sigma epsilon Tc^4 == k (Th - Tc) Out[52]= epsilon sigma Tc^4 == k (-Tc + Th) In[53]:= sigma = 5.67 * 10^-8 k = .8 epsilon = 1 Out[53]= 5.67*10^-8 Out[54]= 0.8 Out[55]= 1 In[56]:= Last[Solve[{eqn1, eqn2}, {Tc, Th}]] Out[56]= {Th -> 384., Tc -> 219.}Finally, if the longwave emissivity is 0.95 but all of the incoming 1360 W/m2 is absorbed by the hot side, we have:
In[57]:= Clear["Global`*"] In[58]:= eqn1 = sigma epsilon (Th^4 + Tc^4) == 1360 Out[58]= epsilon sigma (Tc^4 + Th^4) == 1360 In[59]:= eqn2 = sigma epsilon Tc^4 == k (Th - Tc) Out[59]= epsilon sigma Tc^4 == k (-Tc + Th) In[60]:= sigma = 5.67 * 10^-8 k = .8 epsilon = .95 Out[60]= 5.67*10^-8 Out[61]= 0.8 Out[62]= 0.95 In[63]:= Last[Solve[{eqn1, eqn2}, {Tc, Th}]] Out[63]= {Th -> 389., Tc -> 223.}All comments gladly accepted.
w.
How nice, you were writing your comment at exactly the same time as me!
“The second condition at steady-state is that the flow through the block has to be equal to the flow out of the cold side. The flow through the block is k (T_hot – T_cold), and the cold side radiation is sigma epsilon T_cold ^4, so the second equation is:
sigma epsilon T_cold^4 == k (T_hot – T_cold) [eqn2]”
Uhuh, just as I predicted:
“This is so obviously silly, so he will have to pretend the solution is CHF = CSR (Forgetting HSR), he will get an answer that befuddles HSR and makes it physicially unreal.”
Do you see the problem?
Here is what you did with ”
that the flow through the block has to be equal to the flow out of the cold side ”
Model:
|[XXX]
The “|” represents a infinitesimally small sliver of molecules that are supposedly at 383K while the rest of the of the block “[XXX” is at 221K !!!
This is unphysical. Why can’t the 383K sliver pass this energy onto the next sliver, and next sliver, etc until the whole block is resonating at 383K. What happend to the 162K’s worth of energy? Did the block reject it? Did it go back to the source? If it did then the absorptivity is not 0.95 but MUCH less.
Sorry Willis, but math is not physics.
Conductive Heat Flux is not a conserved value, but goes to ZERO.
I dont get what you are saying Zoe?
The maths says there is a gradient all the way thru the block so it looks like this
383K 987654321 221K
Now that assumes flat sheet laminar flow and we have a slightly unrealistic cube setup.
Are you really saying you don’t think there will be a gradient all the way thru the block?
Why can’t the 9 heat the 8?
Why can’t the 6 heat the 5?
Where is the energy going?
Wllis believes in backradiation. He believes HSR will emit back to its heat source to make it warmer because the block prevented its cooling. This is incompatible with a gradient.
Remember, Willis only makes ideological sense when the pieces of his puzzle are in complete isolation.
He can’t handle all of them at once, because then the solution is all zeroes.
Notice he can’t do HSR = CHF at the same time as CSR = CHF. Even though this breaks his silly water through a hose analogy.
This is not “back radiation”, it’s radiation. It happens from all bodies in all directions, no matter who is looking.
Because each step is radiating in every direction.
“Willis believes in backradiation.”
He does Zoe. After all, it is the basis of the GHE hypothesis. Of course, there is no evidence to support such a belief but it suits alarmists and lukewarmers equally.
Sometimes I don’t know if the difference between alarmists and lukewarmers is only a matter of degree of commitment to a belief system.
Are they sort of like high and low church, Anglo-Catholic v Protestant proponents?
Or are they like those who believe that there are lots of unicorns as opposed to those who believe that unicorns are quite rare?
Sort of floating AGW voters?
leitmotif February 28, 2020 at 3:37 pm
No evidence? Say what? We have a couple of completely independent and very strong lines of evidence.
First, downwelling radiation from the atmosphere has been MEASURED thousands and thousands of times around the world. It’s measured directly by scientists. It’s measured automatically by things like the TAO buoys and the SURFRAD stations. It’s not some imaginary quantity as some people claim.
Second, we know that the surface of the planet is at something around 15°C. So it’s radiating at something like 380 W/m2 or so. However, we also know from both theory and from satellite measurements that only about 240 W/m2 of that escapes the atmosphere.
So the rest, perforce, must be absorbed by the atmosphere. Leaves the ground, doesn’t make it out to space, has to be absorbed by the atmosphere. Good so far?
Now take the final step. If that energy just stayed in the atmosphere, the atmospheric temperature would soon be thousands of degrees.
So that energy is not staying in the atmosphere, and it’s not going out the top of the atmosphere … you do the math. I and most everyone else says that it goes back down to the surface. It’s usually called “Downwelling longwave radiation” (DWLR), or “Downwelling infrared” (DWIR), or occasionally, “backradiation”. However, the last term is confusing, so most folks use another term.
Best regards,
w.
“First, downwelling radiation from the atmosphere has been MEASURED thousands and thousands of times around the world. It’s measured directly by scientists. It’s measured automatically by things like the TAO buoys and the SURFRAD stations. It’s not some imaginary quantity as some people claim.”
So what part of DWLR is back radiation i.e. radiation that emanated from the planet surface that is returned to the planet surface by GHGs? What part of that back radiation is anthropogenic? Feldman et al (2015)? I don’t think so. Not heard of transfer of energy by conduction by non-GHG molecules to GHG molecules. How many collisions between air molecules before a GHG molecule emits a photon? Tens of millions? Hundreds of millions? What?
“Second, we know that the surface of the planet is at something around 15°C. So it’s radiating at something like 380 W/m2 or so. However, we also know from both theory and from satellite measurements that only about 240 W/m2 of that escapes the atmosphere.So the rest, perforce, must be absorbed by the atmosphere. Leaves the ground, doesn’t make it out to space, has to be absorbed by the atmosphere. Good so far?
Now take the final step. If that energy just stayed in the atmosphere, the atmospheric temperature would soon be thousands of degrees.”
Wow! Total Bernie Sanders! You are actually averaging temperatures and fluxes? Is this your Roy Spencer moment?
“So that energy is not staying in the atmosphere, and it’s not going out the top of the atmosphere … you do the math. I and most everyone else says that it goes back down to the surface. It’s usually called “Downwelling longwave radiation” (DWLR), or “Downwelling infrared” (DWIR), or occasionally, “backradiation”. However, the last term is confusing, so most folks use another term.”
The cooler atmosphere heats the warmer planet surface? Can’t argue with that empirical evidence, Willis. Enjoy your fame. 🙂
I stumbled through almost a whole afternoon trying to solve simultaneous fourth-order polynomial equations mathematically, and of course I couldn’t. I looked up stuff on the internet and found mathematical methods that I’d never even heard of; understanding them was way beyond my simple abilities. So I did the “educated trial-and-error” method (we could all it iteration to make it sound respectable) and it only took five steps to get Th = 383.7°K and Tc = 221.4°K. Thanks for the post, Willis, it got my brain going on an otherwise lazy day.
I’m sorry Zoe, it looks as though you just don’t get it. Everything that has a temperature above 0°K and non-zero emissivity/absorbivity is both emitting and absorbing radiation all the time. The hot side is not radiating back only at the sun; it’s radiating in all directions so the fraction received by the sun is beyond infinitesimally small. The omnidirectional nature of black body radiation means that the equations we used in this case are only really accurate if they are applied to an infinite plane rather than a 1 m² concrete block, but the exercise was valuable and entertaining.
Zoe, you seem to be in thrall of the “a colder body cannot heat a warmer body” axiom that we keep seeing from less informed sceptics who want to deny the existence of the greenhouse effect. That is only true if you’re talking about black body radiation. In the atmosphere, radiation (photons) generated by molecules of water (and CO2 to a lesser extent) when they drop from an excited (higher energy) state to a relaxed (lower energy) state is not black body radiation. At least, that’s how my simple geology brain understands it. I could be wrong; I’ve been wrong before (although that may come as a surprise to those who know me).
“Willis believes in backradiation” OK here’s a simple example of what you might call “back radiation” that you can feel yourself. You have to be indoors, in winter, in a house with moderately well insulated walls but single-glazed, clear glass windows, i.e. an old house like mine. Stand in front of a window. The exposed skin of your face, normally at 37°C, is radiating outwards towards the window (and through the window assuming it’s transparent to LWIR). The window at maybe 5°C, and the snowy landscape outside, at maybe -5°C, are radiating back towards you, but at much less intensity than you are radiating at them, so there’s a net outward heat flux from your face. Your normally 37°C skin is now at (say) 30°C, and you feel a bit chilly. Now stand in front of a wall. The wall, at about 20°C, is radiating more heat towards you than the window/landscape did, so the net outward heat flux from your face is less, and your skin is now at (say) 35°C, and you feel warmer. Has the 20°C wall heated your face from 30° to 35°C? Not exactly; but it has lowered the net heat flux from your face, which has caused your skin temperature to rise. “Slows the rate of cooling” is another way of putting it.
You can feel the same effect if you compare how temperature drops after the sun goes down in a desert with how it cools in a more vegetated region where the air is humid. It cools down really fast in the desert compared with elsewhere. In a humid region, there is “back” radiation from water vapour in the atmosphere, and in the dry desert there is much less. This is the greenhouse effect, and the fact that it varies so much between deserts and jungles is clear proof that most of the greenhouse effect is due to water vapour. CO2 concentration is much the same everywhere (“a well mixed gas”) so the GHE should be almost the same in the desert and the jungle if CO2 was responsible. But it’s not.
The idea that everything radiates and absorbs all the time is a bit counter-intuitive, and it’s hard to grasp without taking the time and effort to learn how it works. It’s easy to observe radiation from hot bodies like “radiators” (if your house has hot-water heating) but the fourth-power relationship with temperature means that you have to think hard about it to observe radiative heating and cooling in everyday objects at ambient temperatures. It’s not as difficult as the clock paradox in relativity, but from the comments we see at WUWT, it’s clear that not everyone “gets it”.
LDB
The maths says there is a gradient all the way thru the block so it looks like this 383K 987654321 221K
Zoe
Why can’t the 9 heat the 8? … Why can’t the 6 heat the 5? … Where is the energy going?
Now Moi (Robert K)
I think that 9 DID heat 8, but 8 cooled to heat 7, which cooled to heat 6, which cooled to heat 5, and so on. Now 9 can no longer keep up with 8’s cooling rate to heat 7, 6, 5, etc.
9 has done all the heating of 8 it can do, and likewise on down the gradient. The energy is “going” towards the end, cooling from one place to heat another, via the successive increments of resistance that it must traverse to get through the entire LENGTH of the concrete block.
Somebody (preferably an experienced engineer), please fix me, if I’ve flubbed this. Thanks.
Smart Rock
“I’m sorry Zoe, it looks as though you just don’t get it. Everything that has a temperature above 0°K and non-zero emissivity/absorbivity is both emitting and absorbing radiation all the time. The hot side is not radiating back only at the sun; it’s radiating in all directions so the fraction received by the sun is beyond infinitesimally small. ”
OMG! Do you have any evidence of this or even a mathematical proof? The earth heats the sun? What would the earth do if the sun was not there? Heat the next nearest sun?
“Zoe, you seem to be in thrall of the “a colder body cannot heat a warmer body” axiom that we keep seeing from less informed sceptics who want to deny the existence of the greenhouse effect. That is only true if you’re talking about black body radiation. In the atmosphere, radiation (photons) generated by molecules of water (and CO2 to a lesser extent) when they drop from an excited (higher energy) state to a relaxed (lower energy) state is not black body radiation. At least, that’s how my simple geology brain understands it. I could be wrong; I’ve been wrong before (although that may come as a surprise to those who know me).”
What are “less informed sceptics who want to deny the existence of the greenhouse effect”? Do you have a scale of informed sceptics?
““Willis believes in backradiation” OK here’s a simple example of what you might call “back radiation” that you can feel yourself. You have to be indoors, in winter, in a house with moderately well insulated walls but single-glazed, clear glass windows, i.e. an old house like mine. Stand in front of a window. The exposed skin of your face, normally at 37°C, is radiating outwards towards the window (and through the window assuming it’s transparent to LWIR). The window at maybe 5°C, and the snowy landscape outside, at maybe -5°C, are radiating back towards you, but at much less intensity than you are radiating at them, so there’s a net outward heat flux from your face. Your normally 37°C skin is now at (say) 30°C, and you feel a bit chilly. Now stand in front of a wall. The wall, at about 20°C, is radiating more heat towards you than the window/landscape did, so the net outward heat flux from your face is less, and your skin is now at (say) 35°C, and you feel warmer. Has the 20°C wall heated your face from 30° to 35°C? Not exactly; but it has lowered the net heat flux from your face, which has caused your skin temperature to rise. “Slows the rate of cooling” is another way of putting it.”
Or it could be you reach thermal equilibrium quicker with a warm wall than a cold window? Hmmmm?
If it a still cold night, open the window and see how much back radiation warms you up.
Commonsense law: That which heats cannot be heated by that which it heats. This has to be a fundamental law of heat transfer or we are in a runaway heating scenario that has never happened in the existence or the knowledge of the human race. Heat, the transfer of internal energy, does not flow spontaneously from a cooler object to a warmer object.
Put two lamps 100W and 40W facing each other. Do they both get brighter or hotter because of back radiation?
Shine a light onto a white surface and then reflect the surface light spot onto itself using a mirror. Does the surface light spot get brighter?
Stop talking Bernie Sanders.
“Shine a light onto a white surface and then reflect the surface light spot onto itself using a mirror. Does the surface light spot get brighter?”
Are your eyes equipped with a light meter?
Smart Rock wrote:
“The idea that everything radiates and absorbs all the time is a bit counter-intuitive, and it’s hard to grasp without taking the time and effort to learn how it works.”
Your wording could be less ambiguous. It leads neophytes to the conclusion that objects emit as though they’re in a 0 K ambient, and absorb as though they’re in an ∞ K ambient.
In reality, the underlying physical mechanism which regulates emission and absorption of photons is radiation pressure. If the object has a higher potential than the ambient radiation pressure, it can emit photons into that ambient. If an object has a lower potential than the ambient radiation pressure, it can absorb photons from that ambient.
Here’s some web pages addressing the topic:
https://objectivistindividualist.blogspot.com/2018/08/the-nested-black-body-shells-model-and.html
https://objectivistindividualist.blogspot.com/2015/05/the-greenhouse-gas-hypothesis-and.html
LOL@Klimate Katastrophe Kooks March 1, 2020 at 8:31 pm Edit
According to LOL, nothing can radiate if it is in an environment with higher “ambient radiation pressure”.
You know, like a candle can’t radiate if it is out in the bright sunlight … oh … wait …
Or how if you shine a really bright flashlight at a dim flashlight, the dim flashlight immediately goes out … oh … wait …
Sometimes, I’m simply stunned by the level of nonsense that people believe.
Think about a standoff infrared thermometer. It works by measuring the thermal infrared (longwave) radiation from objects.
Now, according to LOL (an appropriate name given the laughable nature of his claims), the IR thermometer could only measure the temperature of objects that are warmer than the ambient temperature. His claim is that if objects are colder than the ambient they don’t radiate at all because of some mysterious “ambient radiation pressure”.
But of course, that’s obviously not true—IR thermometers can measure the temperature of any object regardless of the ambient temperature.
His whole claim about “ambient radiation pressure” makes no sense at all. The reality is that all solid objects above absolute zero radiate all the time, no matter what the ambient temperature might be.
Best regards to all,
w.
Willis wrote, “Sometimes, I’m simply stunned by the level of nonsense that people believe. … [LOL’s] whole claim about “ambient radiation pressure” makes no sense at all…”
Amen! Thank you for that.
I wondered where on earth LOL “learned” such nonsense, and of course I immediately suspected PSI. So I did a google site search of their site for “radiation pressure,” and found I two people saying such things in the comments. The second went by the handle of “Jonas,” just a few days ago. The first, on November 18, 2019, was (take a guess!)…
.
.
.
…yep, as you probably guessed, it was none other than Zoe Phin. She wrote, “If rafiation pressure from H (hot) is greater than radiation pressure from C (cold), then the photons from H “beats back” photons from C, until C doesn’t emit any photons in the direction of H. A stronger force (pressure/area) beats a weaker force and drives it back home … essentially the weaker force never leaves the house.”
So LOL is probably an alias of Zoe Phin.
I think that’s good news. It means the evident insanity has not spread to so many people, after all.
LOL@Klimate Katastrophe Kooks March 5, 2020 at 12:46 pm
Huh? You said that “radiation pressure” keeps objects from radiating. I asked, if that is the case then how can we see a candle in the huge “radiation pressure” from sunlight?
And how can a strong flashlight and a weak flashlight shine right at each other? Why doesn’t the “radiation pressure” prevent the weak one from radiating?
Your pathetic response is to wave your hands and say the magic word “piffle”? That’s it?? That’s your idea of a scientific argument?
Next, a LINK to whatever “Charles R. Anderson, Ph.D.” uses as “standard equations” would make your response at least understandable … you continue:
Glad to. Here you go:
Net flows and individual flows. The individual flows are from me to you, $100, and from you to me, $75. The net flow is from me to you, $25. Both are equally valid ways of discussing the real flows of money.
What does this have to do with cold and warm objects? It points out a very important distinction, that of the difference between individual flows of energy and the net flow of energy, and it relates to the definition of heat.
Looking at Figure 1, instead of exchanging dollars, think of it as two bodies exchanging energy by means of radiation. This is what happens in the world around us all the time. Every solid object gives off its own individual flow of thermal radiation, just as in the upper half of Figure 1. We constantly radiate energy that is then being absorbed by everything around us, and in turn, we constantly absorb energy that is being radiated by the individual objects around us.
“Heat”, on the other hand, is not those individual flows of energy. Heat is the net flow of energy, as represented in the bottom half of Figure 1. Specifically, a heat flux is the net flow of energy that occurs spontaneously as a result of temperature differences.
Now, the Second Law of Thermodynamics is only about net flows. It states that the net flow of thermal energy, which we call “heat”, goes from hot to cold each and every time without exception. However, the Second Law says nothing about the individual flows of energy, only the net flow. Heat can’t flow from cold to hot, but radiated energy absolutely can.
Do you ignore polite requests? Because that’s what you’re doing. From above in the head post:
Thanks,
w.
LOL@…, Willis has already handled the scientific arguments very well, so this comment is just about your personal remarks.
LOL@… wrote, “Dave Burton… and I have been in a months-long dispute on CFACT, wherein he’s claimed that there are no restrictions on 2LoT violations at the quantum level…”
I have no idea what you’re talking about. I do not recall ever encountering you before this month, neither on CFACT, nor anywhere else. What alias did you use?
Perhaps you’ve been arguing with a different Dave Burton. Will you please post a link to the argument?
LOL@… wrote, “…Dave Burton had his deluded arse drop-kicked by physicist Dr. Charles R. Anderson, PhD on this very topic, which is why Dave so hates the good Dr. that he’s attempted doxing him in the past.”
I don’t know who Dr. Charles R. Anderson is, and I certainly don’t hate him. To the best of my knowledge, I’ve never had any interaction with him. Will you please post links to the alleged arse-kicking and alleged attempted doxing?
To Dave Burton:
I wouldn’t put it past you to claim you are not who you are, Dave… I’d be embarrassed if I were you, too… but your phraseology, interests and denials of reality coincide too closely to the “other” Dave Burton”:
https://disqus.com/home/discussion/cfact/un_climate_roadshow_opening_in_nyc/
Have you experienced a dissociation, Dave? LOL
To Willis Eschenbach:
CLAES JOHNSONprofessor of applied mathematics
http://www.csc.kth.se/~cgjoh/ambsblack.pdf
Notice the requirement in (14.3) that T2 > T1. In the literature one finds the
law without this requirement in the form
Q_12 = σT4_2 − σT4_1, Q_21 = σT4_1 − σT4_2 = −Q_12 (14.5)
where Q_21 is the heat transfer from B1 to B2 as the negative of Q_12.
This form has led to a misinterpretation of Stefan-Boltzmann’s Law as
expressing heat transfer from B2 to B1 of size σT4_2 balanced by a transfer
−σT2_1 from B1 to B2, as if two opposing transfers of heat energy is taking
place between the two bodies with their difference determining the net flow.
Such a misinterpretation was anticipated and countered in Stefan’s original article [42] from 1879:
• The absolute value the heat energy emission from a radiating body cannot be determined by experiment. An experiment can only determine the surplus of emission over absorption, with the absorption determined by the emission from the environment of the body.
• However, if one has a formula for the emission as a function of temperature (like Stefan-Bolzmann’s Law), then the absolute value of the emission can be determined, but such a formula has only a hypothetical meaning.
Stefan-Boltzmann’s Law (14.3) thus requires T2 > T1 and does not contain
two-way opposing heat transfer, only one-way heat transfer from warm to
cold. Unfortunately the misinterpretation has led to a fictitious non-physical
”backradiation” underlying CO2 global warming alarmism.
Ready to give up and acknowledge reality yet, Willis? LOL
LOL@Klimate Katastrophe Kooks March 5, 2020 at 7:48 pm
Pass, thanks. Now that you’ve linked to it, I can see that you’re just pushing the bog-standard Slaying the Sky Dragon nonsense, and my life is far too short to engage in disputing childish misunderstandings of that nature.
w.
To Willis Eschenbach:
From my prior comment:
Such a misinterpretation was anticipated and countered in Stefan’s original article [42] from 1879:
I suggest you educate yourself, Willis. Now you’re implying that Stefan was a “sky dragon slayer”? LOL
LOL@… wrote, “To Dave Burton: I wouldn’t put it past you to claim you are not who you are… https://disqus.com/home/discussion/cfact/un_climate_roadshow_opening_in_nyc/ “
That’s not me. I have not participated in that discussion, at all. That’s “DaveBurton72,” who I suspect might be the same person who impersonated me on WUWT.
I had a conversation with that “DaveBurton72” in a different thread; here’s a screenshot:
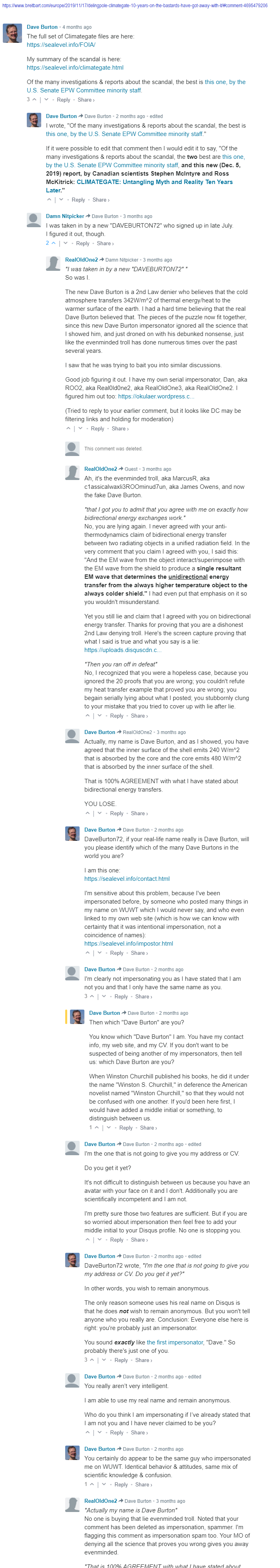
DaveBurton72 denied that he was intentionally impersonating me. He claimed it was just a coincidence of names.
LOL@… also previously wrote, “…Dave Burton had his deluded arse drop-kicked by physicist Dr. Charles R. Anderson, PhD on this very topic, which is why Dave so hates the good Dr. that he’s attempted doxing him in the past.”
As I wrote before, I don’t know who Dr. Charles R. Anderson is, and I certainly don’t hate him. To the best of my knowledge, I’ve never had any interaction with him. My guess is that it was “DaveBurton72” again, but I’d like to know. So, will you please post links to the alleged arse-kicking and alleged attempted doxing?
LOL@Klimate Katastrophe Kooks March 5, 2020 at 9:01 pm Edit
I, on the other hand, suggest that you are far too arrogant and self-important to follow my polite request that you quote whatever it is that you are babbling about. In fact, I was reacting to the statement in your link that his work was instrumental in the Sky Dragon fantasies …
Hard pass. I have ZERO interest in your Sky Dragon madness.
w.
To Willis Eschenbach:
You left out a bit on your image… I’ve helpfully included the requisite information. You’re welcome. LOL
Mheh, it’s late. Need to sleep.
To Willis Eschenbach:
You left out a bit on your image. I’ve helpfully included the requisite information. You’re welcome. LOL
To Dave Burton:
Yes, that screen shot certainly sounds exactly like the Dave Burton I’ve been drop-kicking over on CFACT… he’s easy to spot, his hubristic attitude combined with his confused take on scientific topics is such that even when he was socked up as ‘Anonymous’ on physicist Dr. Charles R. Anderson’s website, he was easy to spot. LOL
Apologies if I’ve offended. Clearly he’s been ‘impersonating-not-impersonating’ you… he’s very passive-aggressive that way.
There you have it that is the bit why Zoe doesn’t understand 🙂
So lets do this the the layman way.
Zoe can I ask why can’t one bit be 100 then?
Why can’t one tiny bit of the metal go to many thousand of whatever unit we are using and explode into a ball of plasma ?
Why have we never seen this on the ISS and satellites?
Have you ever seen it or heard of it?
You see the problem you are setting up and just by thinking about it as a thought experiment it is obvious your answer is wrong.
“Why have we never seen this on the ISS and satellites?
Have you ever seen it or heard of it?”
Show me the telemetry data. Don’t quote me the max temp on hot side vs. min temp on cold side. We’re not told if this is concurrent. We’re not told what both sides are facing and their angles and view factors.
There’s not enough information to reach a conclusion, and I could explain it with that information.
Thanks leitmotif !
As usual Willis ignores that satellites can’t detect IR moving away from it, and all surface based instruments are measuring Upwelling-from-the-instrument-IR (which came from surface upwelling IR) which he believes is DWLWR.
He’s not shown what the instruments emit because it’s the same as DWLWR.
” … Upwelling [IR PHOTONS] -from-the-instrument-IR (which came from surface upwelling IR) which he believes is DWLWR.
—
How can an IR sensor facing the zenith detect it’s own self-emission of IR photons that are traveling away from the sensor? That’s absurd nonsense Zoey.
The photon has to be directed at the sensor, not away from it, for the sensor to detect such photons. They are only detected if coming toward the sensor’s field of view. What you are claiming would not and could not be detected!
But detectable photons do come from above, and are being measured.
And as ‘smart rock’ just explained, namely, everything absorbs and emits all of the time in every direction, at all temperatures. That is a fairly basic EM concept.
Zhoe
If what you say is true the earths crust would be the same temperature as the core.
“How can an IR sensor facing the zenith detect it’s own self-emission of IR photons that are traveling away from the sensor? That’s absurd nonsense Zoey.”
Hello, there’s a voltage gain/loss by instrument according to how much the instrument heats the environment or cooled by environment.
If the instrument is warmer than the atmosphere, the instrument LOSES temeprature, and warms the atmosphere.
A photon sensor at 10C in an environment at 10C would detect NOTHING, if it didn’t have a local thermometer.
Zoe Zhoe Zoey
They can’t even get your name right and it’s only 3 letters! :-DDD
“How can an IR sensor facing the zenith detect it’s own self-emission of IR photons that are traveling away from the sensor? That’s absurd nonsense Zoey.”
Hello, there’s a voltage gain/loss by instrument according to how much the instrument heats the environment or cooled by environment. If the instrument is warmer than the atmosphere, the instrument LOSES temeprature, and warms the atmosphere. A photon sensor at 10C in an environment at 10C would detect NOTHING, if it didn’t have a local thermometer.
—
Hi.
Which answers nothing about how it senses a whole spectrum of photons moving away from its sensor.
You suppose the designers and testers of such IR sensors didn’t think of self-noise and how to deal with it? The IR sensor will have a temperature sensor integrated at the chip level to correct for and filter self-noise. Then integrated cooling to minimize such self-noise altogether and get the sensor and apparatus below the temperature of the targeted gases in the atmosphere.
But according to your interpretation of physics, there are no thermal photons from the atmosphere coming downward toward a ground based IR sensor pointing at the zenith anyway unless the IR emitting object or molecule above is marginally warmer than the Earth.
All objects above absolute zero can and do emit photons in any direction irrespective of the temp of an object within the (cosmic) ray-path of an emitted photon. How would or does the emitting molecule, atom or particle, know the thermal geography of the entire probably infinite cosmos, and even takes into account gravitational-lensing effects on ray path, and the locations of all rarefied ‘hot’ particles, in the periphery of galactic clusters, and can plot their future location for when the photon finally reached them, BEFORE the emitting molecule chooses an emission direction which can only ever go towards a colder emission cosmic pathway?
Seems complicated.
You make this ‘inescapable’ interpretation of the physics that atmospheric gases do not emit photons in all the available directions when they have enough energy to emit one, unless the direction of the emission is at a lower temperature than the emitting object … even at cosmic scale radii!
Thus such ground-based sensors must (according to you) necessarily be presumed to not be detecting IR at all from the atmosphere’s emission, no matter what the temperature of the cooled IR sensor and its apparatus is. As it could only ever be self-noise masquerading as an atmospheric IR spectral signature, due to photons moving away from the sensor as no photons could ever emit towards a warmer atmosphere and earth below the emission altitude.
But what if all the molecules in the cosmos instantly learned that we cunningly chilled the IR sensor molecules to below the temperature of the atmosphere, and thus the atmosphere does emit toward the chilled sensor … just to mess with us?
I have to go now.
“This is unphysical. Why can’t the 383K sliver pass this energy onto the next sliver, and next sliver, etc until the whole block is resonating at 383K. What happend to the 162K’s worth of energy? Did the block reject it? Did it go back to the source? If it did then the absorptivity is not 0.95 but MUCH less.”
Beginning to get the gist of this Zoe. Say, on an abandoned city street, 383K beating down. I can’t imagine that a metre below the surface it would be 100 to 150 degrees Celsius lower no matter what insulation was used.
More sh1te physics from warmists and lukewarmists.
Hi Willis. This would make a good problem for the Mechanical Engineering PE exam. I got 388.3K hot surface and 223.6K cold surface (115.1 and -49.5 C). Quite close to Roy Spencer’s answers. I did my calculation in Inch-Pound units (BTU, feet, degrees Rankin. etc.) so some rounding and conversion differences. Also I used S-B to calculate an apparent source surface temperature for the 1360 watt Flux to an absolute 0 surface as specified in the problem. I also assumed a 4 C temperature for empty space that the cold surface would radiate to. The net heat fluxes in all three areas – sun to hot surface, hot surface to cold surface, cold surface to space – work out to about 134 watts.
Part of the challenge was to explain how you got the answer.
Yes Rick, fellow PE.,our first year mechanical engineering courses have not let us down. I got the same as you somewhere in the above blogroll. But Willis is assuming 0.95 x 1360 for heat input giving him a few degrees different temperatures. Other minor numerical differences between replied answers are a result of using 5.67037e-8 for the SB constant, or 5.6704e-8 or 5.67e-8 which also result in a degree of disparity.
Correct.
One needs two equations on the two temperatures.
Either using power power balance separately at the both sides, or select one side (you choose the cold side) and the total energy balance for the block.
The solution is naturally the same.
Yours is the same method I used except I used a simple binary goal seeking process in Excel yet got a result slightly different but not insignificantly different to yours. See 3 posts above. I did use a finer value for sigma (=5.670367*(10^(-8)) which I hope accounts for the slight difference.
Now with that answer we can calculate a average temperature of 302 K.
This is 471 W/2. So over the surface the calculated emission would be less than what is incoming.
We can calculate the temperature for which the incoming solar radiation is valid for the average and it is 335K.
The interesting part is that it calculates a 33K difference. Does this sound familiar?
So climate physics and real physics differ by 33K.
Not sure what you make of this. Let us know.
Willis obviously took my example from:
https://phzoe.wordpress.com/2019/12/04/the-case-of-two-different-fluxes/
Willis believes that there must be conservation of heat flow.
He believes that
Hot Side Radiation = Conductive Heat Flux = Cold Side Radiation.
Forget his parameters, and simplify:
HSR => [ CHF ] => CSR
Conduction Formula: q = KA(Th-Tc)/L
Radiation Formula: q = ɛσT⁴
Set Absorptivity = Emissivity = 1
Set K = L = A = 1
HSR = σ(Th)⁴
CSR = σ(Tc)⁴
CHF = Th-Tc
Assuming HSR = CHF = CSR:
σ(Th)⁴ = Th-Tc = σ(Tc)⁴
There is only one solution!
Th = Tc = 0
HSR = CHF = CSR = 0
This is so obviously silly, so he will have to pretend the solution is CHF = CSR (Forgetting HSR), he will get an answer that befuddles HSR and makes it physicially unreal.
Thanks, Zoe. I think our last two comments crossed in the ether. I actually linked to that post of yours in a comment upthread.
Best regards,
w.
PS—I don’t “pretend” anything. I tell the truth as best I know how. I asked politely, with you in mind, to lay off the ad hominem attacks. Please do so.
Willis
I wonder, based on style and muddy thinking, wether Zoe is actually Sou of Hotwhopper losing traffic.
You seem to have ignored the input radiation on the hot side.
Zoe, what is physically unreal is you example where you choose an arbitrary heat flow of 2W/m^2 and then , for no reason, assume that this is compatible with the “cold end” being cooled by SB radiation. That is not the case, it is not compatible with that heat flow.
You then use both the thermal conduction equation and the SB equation as though they represent the same physical system and come to the ridiculous conclusion that you can radiate 557W from the “cold end” of a bar which is receiving on 2W.
The fact that this is a flagrant violation of one of the foundational axioms of physics should have been a clue that you screwed up somewhere.
Greg
”
the “cold end” being cooled by SB radiation.
”
What object is the cool end heating? None
You’re confusing a radiating potential with heat transfer.
Waving EM waves in space is not a cooling mechanism. Get another piece of matter and then you have cooling.
Good Lord. For the second time today.
I just finished whacking an idiot “economist” for his belief that throwing a wad of money at people will cause them to ignore their (unfounded, but very human, especially considering the political hacks like Nasty Nancy and Micro Mike fanning the flames) fear of contracting the coronavirus, and rush into the retail stores to buy stuff and avert a recession.
Is there such a thing as Keynesian Physics? If not, I take credit for properly naming this field of foolishness.
Energy is energy. Whether it is in the physical motion of a molecule (kinetic) or the “waving” of a photon (radiation), it is the same thing.
Energy out = energy in – energy retained*. At steady state, energy out = energy in – otherwise, an object is either gaining kinetic energy (heating) or losing it (cooling). If the energy in is radiation, the energy out is also radiation.
*Before a pedant gets around to me – yes, I know that it is actually mass/energy out = mass/energy in – mass/energy retained. But under any but extreme conditions, we can happily ignore the Book of Albert.
Zoe, a potential is not a flux. The object radiating does not care where the energy is going.
Cool. You debunked Quantum Mechanics. All photons are possible, then.
Funny, Boltzmann and Planck thought that if the separation distance was 1 meter, no 2m wavelength photon will form. But I guess that assumption was wrong and so B & P didn’t derive their correct formulas.
Remember, all photons are possible and they don’t care where their going ! LOL
B & P were wrong !!! LOL
what is a “radiating potential” anyway?
Boltzmann and Plank did not use that, they did not say you needed to have another body waiting to be warmed before black body radiation would occur. The idealised cavity is not the only thing which emits thermally generated photons.
EM Radiation is a form of energy, so transformation of thermal kinetic energy into radiation is not a “potential” it actually happens. When a photon is emitted the thermal energy ( temperature ) of the object is reduced.
“Boltzmann and Plank did not use that, they did not say you needed to have another body waiting to be warmed before black body radiation would occur.”
So what how did they measure the radiation?
Was it not with another object?
“it actually happens”
Yes, to another object, and no one had observed otherwise!
I’m a photon sitting at the cool end, waiting for something to appear so that I can leave. I think I can see something a few light years away, but I can’t risk leaving in case it’s no longer there when I get there. It might not even be there now (whatever “now” means). I’m starting to get the feeling that I’m never going to leave.
Good one.
Zoe
“You’re confusing a radiating potential with heat transfer.”
Isn’t this the fundamental misunderstanding of the whole AGW hypohesis?
Some radiation falls on stony ground.
The thing is bash-awk-wards.
σ(Th)⁴ = Th-Tc = σ(Tc)⁴
This is just wrong, because the first term is of opposite direction than the two others. You need to put in power P = σ(Th)⁴ + σ(Tc)⁴ to get the ends meet. Zoe appears to think 1360 W equals σ(Th)⁴. We didn’t say that. Conservation of energy is what the calculation is based on.
‘He believes that
Hot Side Radiation = Conductive Heat Flux = Cold Side Radiation.’
Wrong conclusion. power out = HSR+ CSR = power in = ɛ 1360W.
PS I constructed the equations but didn’t bother to solve 16th grade polynomial on pen and paper. Cool.
And where does CHF fit in?
Zoe posted: “Assuming HSR = CHF = CSR:”
In Wilis’ problem statement, there is no reason to make this assumption, and in fact it is wrong. The hot side surface receives incoming radiation, re-radiates a large fraction of that energy ON THE HOT SIDE and transmits (via conduction) a relatively small amount of that received radiation to the cold side, where is is radiated to deep space.
Uhuh,
So only CSR = CHF, HSR can remain completely disconnected?
Gordon,
“The hot side surface receives incoming radiation, re-radiates a large fraction of that energy ON THE HOT SIDE and transmits (via conduction) a relatively small amount of that received radiation to the cold side, where is is radiated to deep space.”
Then absorptivity on the hot side is NOT 0.95 or the albedo is insane.
But even if that’s not the case,
Willis has a fake 1-molecule thick sliver at 383K.
Why an object would refuse energy is a mystery!
To first-order, albedo = (1- absorptivity). Therefore, a true blackbody has a absorptivity of 1.0 and an albedo of zero. Likewise, for Earth (with clouds) the average albedo can be 0.30, meaning that 70% of incoming solar radiation is absorbed in the clear atmosphere, the clouds or at Earth’s water and land surfaces. And the Moon, having no atmosphere, has an albedo of 0.12, meaning that 88% of the incoming solar energy is absorbed directly at its surface. There is nothing “insane” about any of these albedos.
Furthermore, albedo has little to do, per se, with the emissivity of a given object’s surface . . . albedo involves INCOMING radiation from objects across any range of temperatures, whereas emissivity involves OUTGOING radiation at the specific (or relatively narrow range) of absolute temperature of the emitter.
Willis’ postulated insulated concrete block is not “refusing” energy . . . it is absorbing the 1360 watts (or 1360*0.95 watts if you want to account for, and say, absorptivity ~ emissivity, and it is thus naturally reflecting back to space 1360*.05 = 68 watts in this case).
There is no mystery here.
I don’t think this is so complicated, the total out going from BOTH sides of the cube has to equal the in coming. So there can and will be a difference in temperature on either side.
Language drift is an interesting phenomenon. I’ve always been fascinated at how the Romance languages sprang up and matured so quickly after the fall of Rome.
Is it acceptable now, in American English, to use “metre” instead of “meter”?
Really just curious.
Matthew, “metre” is definitely not the American usage. I use it, quite idiosyncratically, because otherwise it gets confused in my own mind with a meter meaning a device used to measure something. I will say that it drives my spelling checker nuts.
I believe someone once commented that the US and the UK are two countries separated by a common language …
Regards,
w.
English metre comes from french metre, which ironically can also mean a length or a measuring device. The british English use of both has the advantage of removing the ambiguity, as W. says.
Thanks for setting us such an intriguing problem.
Maybe that is why in the USA you drive on the right, and in the UK we dont…
There is a more logical answer but that can wait for another time.
There is a more logical answer but that can wait for another time.
Cutting down your ‘opponent’ with the sword in your right hand. Left hand controlling the Horse.
Contrast that to the french habit of passing on the right. Similarly derived from the days of horses and swords, their convention was to pass in a way which impeded an attack by either side, since the sword hand was not on the side of he who was passing.
British English ‘Meter’ is the measuring device (Water, Gas, Electricity quantity)
British English ‘Metre’ is a distance measurement (Km, Cm or even (converted) Miles)
To help non-native English speakers, context (and inflection) is all.
(many words sound the same … to, too, two but have very different meanings and only context separates them in speech)
3×2
Such as “micrometer” — a distance — and a “micrometer” for measuring distance. The meaning depends on where the emphasis is placed (mi and e) in the first instance, or (cro) in the second. I’ve never understood why Brits place the emphasis on “lo” in kilometer. Bad habit, I suppose.
I measured the width (dimension) to the nearest micrometer (millionth of an SI metre) using my micrometer (instrument used to measure said dimension) …
English. Madness converted to a language.
But compare “nanometre” and “manometer”.
First, It is impossible to answer the question precisely without knowing what percent of the visual hemisphere of the front side of the block is occupied by the Sun that is providing the 1360 W/m^2 of incident radiation (sunshine, as specified).
However, if we ASSUME the radiating object is far enough away that it subtends of angle like that of the Sun as seen from Earth (0.5 degrees), equivalent to 6.8×10^-5 steradians, or .001% of a hemisphere, we can basically neglect this small area and then assume the front face radiates, as does the back face, to a full hemisphere of deep space that has a uniform “background” temperature of 3 K.
Thus we have three equations to solve simultaneously in order to derived the EQUILIBRIUM temperature of the concrete block:
Letting Tf = the front side of the concrete block and Tr = the rear side of the block,
>Front face net power: net radiation power received equals power radiated back to space plus power conducted to the back face, or [(1360 W/m^2) * A] = [A*e*sigma*(Tf^4-3^4)] + Pk
>Power conducted thru block (Pk): Pk = k*A*(Tf-Tr)
>Back face total power radiated: must equal power conducted thru block at equilibrium temperatures), or [A*e*sigma*(Tr^4-3^4)] = Pk,
where:
A is the cross-sectional area of the concrete block (= 1 m^2),
e is the emissivity of the block at its radiating temperature (= 0.95)
sigma is the Stefan-Boltzman constant (= 5.7*10^-8 W*m^-2*K^-4),
k is the block’s thermal conductivity from front face to back face (= 0.8 W/m/K),and
the block surfaces are assumed to behave as ideal radiators across the spectrum (i.e., are “grey bodies”) and all angles relative to the surface.
Above equations reduce to (in units of watts):
1360 = [0.95*sigma*(Tf^4-3^4)+0.8*(Tf-Tr)]
and
[0.95*sigma*(Tr^4-3^4)] = [0.8*(Tf-Tr)]
or to the single equation: (1360/(0.95*sigma)] = [(Tf^4-3^4)+(Tr^4-3^4)].
Setting up a spreadsheet to calculate the solution of this equation across a range of plausible Tf’s, we arrive at the solution of Tr = 222.5 K when Tf = 388 K, with the conducted and rear face radiation powers equalling about 132.5 W each.
Gordon,
The total emission of the two faces at the temperatures you have calculated is: 1285 (front) + 139 (back) = 1424 watts. You have an error in your calculation.
No, using the values and precision of the units that I gave (and specifying that the heat input to the concrete block’s front face was 1360 watts, not 1360*0.95 watts to account for postulated absorptivity . . . there is some confusion on this), I get 1227.2 watts radiated off the front face, 132.5 watts conducted = 132.5 watts radiated from back side and the total of these two radiations is 1360 watts, which is the net input (that I started with). It all balances.
Please recheck YOUR calculations to make sure we are using the same constants.
Gordon, I don’t see the relevance of the steradians. It is given that there is a heat flux of 1360 W/m^2. The front face will radiate to the full hemisphere whatever the size of the source. You seem to be thinking that it is not allowed to radiate back to the sun because it’s hotter. People have been banned from this site for such silliness ( when carried to momentous extremes ).
You’re making it way too difficult. While Willis did say “…sunshine…” the remainder of the text, and the sketch, both indicate uniform radiation to me. And therefore, regardless of any “back radiation” (a mythical concept, if you have taken a heat transfer class) the net result is 1,360 W/m² incoming radiation.
The only reason “back radiation” is mythical in this thought-experiment is that there are no radiatively active gases (in fact, no gases at all) surrounding the mini Borg Cube.
On the Earth, back-radiation is real and measurable (albeit with some challenges).
Red94ViperRT10, you posted “. . . regardless of any ‘back radiation’ (a mythical concept, if you have taken a heat transfer class) . . .”
OK, what then in the full S-B equation for radiation power transfer FROM flat plate 1 of area A TO parallel flat plate 2 also of area A , P=A*e*sigma*(T1^4-T2^4), would you deign to call the power quantity associated with A*e*sigma*T2^4?
Gordon
He specified that 1361W/sq.m was entering the surface. He does not need to specify anything else about the source.
He would need to frame the problem differently if required the view of the heat source to be considered.
Sorry, RickWill, but this issue in not the energy input, which as you say Willis specified as 1360 W/m^2 (although there is some confusion if this was meant to be the total input going directly into the block’s from face, or if it the energy into the block’s face needed to be knocked down to 1360*0.95 W/m^2 to account for the block surface absorptivity).
On the other hand, one does have to account for the integrated (T^4)*dA of the space that energy is being radiated toward FROM the front face of the block for accounting. For example, if the postulated concrete block was, say, orbiting a 100,000 km brown dwarf at 100 km altitude, the back-radiation would basically toward the surface temperature of the brown dwarf (say 5,000 K), not deep space at 3 K, even though the net incoming power flux could be identical at 1360 W/m^2.
Radiant energy flux is unidirectional. It exists by virtue of THE electro-magnetic field – singular. There is no two way energy. A proper solution requires solving Maxwell’s equations for the geometry but you can get close using radiant heat transfer equations setting up the view factors then simplifying assumptions as you have done.
Sorry RickWill, here’s the thought experiment for you: there is a heat lamp shining normally on a mirror, which is 90% reflective, located five feet in front of the lamp. You stand directly behind the heat lamp (receiving no radiation directly from it), facing the mirror, and notice that your face is getting warm quite rapidly. How is this possible if radiant energy flux is unidirectional and exists solely within a singular EM field.
Also, since photons are emitted off any infinitesimally small hot surface in a random directions (at least within a hemisphere), how can “radiant energy flux” be unidirectional? Wouldn’t that violate the law that light intensity falls off as 1/(distance^2) . . . as is commonly known to be the case for sunlight?
The mirror example does not make your case. What I stated is that EMR is unidirectional at any point in space and time. You would need to look at the field that is created by the mirror in combination with the lamp and the face experiencing the EMR. The presence of the mirror simply alters the field.
Please provide experimental evidence that “photons” are emitted in random directions. EMR can only emit toward regions of lower electrical potential. That is the nature of electricity.
No such thing as EMR travelling against the electric field – always down potential. No going against potential. It is not a balance of energy flows; it is a singular energy flow from region of high potential to region of lower potential.
RickWill posted: “Please provide experimental evidence that ‘photons’ are emitted in random directions.”
There is no need to go to the lab when the very basics of the underlying physics suffices:
“A blackbody is a diffuse emitter which means it emits radiation uniformly in all
directions. . .”, and
“The emissivity of a surface is defined as the ratio of the radiation emitted by the
surface to the radiation emitted by a blackbody at the same temperature. Thus,
0 ≤ ε ≤ 1
Emissivity is a measure of how closely a surface approximates a blackbody . . .
The emissivity of a surface is not a constant; it is a function of temperature of the
surface and wavelength and the direction of the emitted radiation, ε = ε (T, λ, θ)
where θ is the angle between the direction and the normal of the surface.
The total emissivity of a surface is the average emissivity of a surface over all
directions and wavelengths . . .”
—source of above-quoted text: http://www.mhtl.uwaterloo.ca/courses/ece309_mechatronics/lectures/pdffiles/summary_ch12.pdf
Of course, you could author a ground breaking paper showing that this is all wrong and all diffuse surfaces must emit unidirectional radiation per Maxwell’s equations.
Unless you can prove it in a lab it is speculation. You cannot emit EMR against the potential field even if it is an energy quantum.
This lecture might give you more insight regarding EMR:
I look forward to seeing your peer-reviewed paper of your claims enjoy all the success that it deserves.
For those that interpret Willis’s problem setup to mean that 0.95*1360 W/m^2 is the energy absorbed on the cube’s front (“hot”) face: the answers I gave above I get changed slightly to Tr = 220 K with Tf = 383 K, with the conducted and rear face radiation powers equalling about 127 W each.
Willis,
Roy is correct about the formulation, it is two equations and two unknowns.
The first equation is the overall energy balance:
0.95 * K * Tf^4 +0.95 * K * Tb^4 = 1360 *0.95 The 0.95’s cancel, so:
K * Tf^4 + K * Tb^4 = 1360 (Eq 1)
where Tf – front face temperature, Tb is back face temperature, and K is the Stefan-Boltzman constant (5.67 *10^-8)
This allows expression of the Tb value in terms of the Tf value:
Tb = (1360/K -Tf^4)^0.25 (Eq 2)
The second equation comes from the requirement that the difference in thermal emission between the two plates must be equal to the rate of heat flux from front to back:
0.95 * K * Tf^4 – 0.95 * K * Tb^4 = 0.8 * (Tf – Tb) (Eq 3)
You substitute for Tb using equation 2 above, and get this rather messy equality expressed in Tf alone:
2*(0.95/0.8) * K * Tf ^4 – (0.95/0.8) * 1360 = Tf – (1360/K – Tf^4)^0.25
Then through the magic of Excel, you can calculate the left and right sides of this equation in two adjacent cells, and try assumed front temperatures until the value of the two columns matches. The result is (to an accuracy of 0.1 degree):
Tf = 347.5 kelvin
Tb = 312.1 kelvin
Your Eq. 3 is wrong
Sorry, typo:
2*(0.95/0.8) * K * Tf – (0.95/0.8) * 1360 = Tf – (1360/K – Tf^4)^0.25
should be
2*(0.95/0.8) * K * Tf ^4 – (0.95/0.8) * 1360 = Tf – (1360/K – Tf^4)^0.25
Fixed, Steve. I hate typos, and since WordPress doesn’t offer an option to edit a post, by default I’m the WordPress Editor.
w.
The correct values are ~383.7 hot side 219.7 cold side.
I used to know a Steve Fitzpatrick. From Pearland, TX. But seems to me someone told me he’s dead, so probably not the same guy?
Or could alter it, by putting block with not insulated side up on lunar surface at the equator and other not insulated side rests on lunar surface.
The block would warm up to about 120 C during lunar noon, and would cool down during lunar night. And evenually it would warm the lunar surface around it, as that surface is almost a perfect insulation.
And block is getting less than 1/2 solar energy {on average roughly* } than a block in space.
* actually, quite a bit less than 1/2.
That would be a completely different problem. As Willis stated the problem, cold side radiates to a perfect (which I suppose I would have to state in my assumptions, some have assumed radiating to some temperature above absolute zero) black body, while the same block on the lunar surface is either radiating to the gray body lunar surface, or even conducting to the lunar surface. And either way we could assume a lunar surface with homogeneous properties for that m² but we can be virtually certain that it is not. Let’s stick to the problem that Willis laid out, it’s simpler.
Speaking of the problem that Willis laid out, rather than the insulation of imaginary Unobtainium we could have featured an equally mythical infinite sheet of 1 m thick concrete, receiving a uniform 1,360 W/m² incoming radiant energy and we are calculating the steady-state temperatures of the center (how does one measure the center of an infinite anything, because by the definition of infinite, I could move over a meter or two or ten or even a million and still have the identical infinite expanse on all sides… but I digress…) m², the calculation is the same, the point is we don’t have heat wandering off to the left or right or wherever, there’s only one direction it can go, which makes all the heat flux exactly parallel to any other heat flux.
But even more importantly, Willis you have specified “…steady state…” but if there is a flux, something is moving, so that’s not steady state, is it? Well, in Fluids we assume steady-state flow all the time, which typically just means that it has settled down to either laminar flow or turbulent, and even if it’s turbulent we still call it steady state, even though in turbulent flow the direction(s) of the fluid is(are) constantly changing. So I could support your answer. Now I really need to dig out my Heat Transfer book!
If then block is perfectly insulated on all sides (as the problem states) Then all radiation ingoing and outgoing is on the front face. There is no radiation loss on any of the other faces. That means the front and back side of the block are at the same temperature!
Jim, it’s insulated on four sides, with the front and back uninsulated. Sorry for the lack of clarity.
w.
excuse me, I’m not a scientist and I have never met Fourier or Stefan-Boltzmann or taken second course. But, if emissivity and absorptivity are equal, then hot and cold side temps are equal. After reaching whatever equilibrium temp is absorbed by 1360 w/m3, the back side emits the same. If the emissivity were less, then heat would reflect back out the hot side. If emissivity were greater, there would be a temperature difference, and a flow from high to low.
thot = tcold
Bonus points for extra credit, temps at the sides under the unobtanium are equal to thot and tcold
Nope.
You are leaving out what happens to the hot side when it heats up.
It emits radiation, as all objects do that are not at 0°K
I protest, it is far too difficult:-)
I start with two simplified models.
If the backside had also been insulated, it would have been easy:
Energy in = 1360 * 0.95 = 1292 W.
By Stefan Boltzman we get T = 4th root(1292*SBconstant*0.95) = 394 Kelvin
And without insulation on the backside, but if the concrete had been superconductive, it would also be quite easy. The temperature would then be equal on both sides, and the emitted energy on each side would have been 1292 /2 = 646 W.
i.e T = 4th root(646*SBconstant*0.95)= 331 Kelvin
You have a problem with a concrete block with final conductivity. That is mean. 🙂
But now we have the extremes, so we know that the answer must be somewhere between there
From the above we know that the hot side has to be colder than 394 Kelvin since some of the energy goes through the concrete. And it has to be hotter than 331 Kelvin since the finite conductivity in the block now hold some energy back.
We also know that the cold side has to be cooler than 331 Kelvin, since it will receive less energy than from a superconductive cube.
I leave the rest to others of my fellow nerds out there. 🙂
/Jan
Some quick thoughts:
Let Tw be the temperature of the warm side, Tc that of the cold side. For the warm side, heat flux in from the Sun is e * Qs, where e=0.95 is the absorptance, same as emissivity in this case. Radiated flux out is e*sigma*Tw^4. In addition, there is a conducted heat flux of k(Tw-Tc)/L, where L is the depth of the cube (1 meter). Equilibrium at the warm side demands:
e*(Qs-sigma*Tw^4) – k(Tw-Tc)/L = 0.
For cold side, the radiative heat flux in is e*sigma*TCMB^4, where TCMB is the temperature of the cosmic microwave background. The flux is ~3*10^-6 W/m^2 and could be disregarded. Equilibrium at the cold side demands:
e*(QCMB-sigma*Tc^4) + k(Tw-Tc)/L = 0.
This is a pair of 4th order polynomials, and a numerical solution is easiest. Using e.g. Mathematica it is easy to see that there is one real solution with both Tw > 0 and Tc > 0. With numerical values substituted, we get Tw ~ 383 K and Tc ~ 221 K, assuming I didn’t typo anything. The temperatures seem high, but not altogether unreasonable. Increasing k yields Tw ~ Tc ~331 K as it should, since now the two sides must be at equal temperatures. Decreasing it gives asymptotically Tw ~ 393 K, Tc ~ 2.7 K. In this limit, both sides are completely uncoupled, and at least the cold side temperature is reasonable, since it is just the CMB temperature we put in originally.
Here is my solution:
In the state of equilibrium, the heat flow through the cold side is the same as through the warm side. The temperature Tspace of the “outer space” is assumed to be 0 Kelvin. The calculation of the equilibrium temperature of the cold side of the cube is then as follows:
HeatFlux = epsilon * sigman * Area * (Tcold ^ 4 – Tspace ^ 4)
1360 = 0.95 * 5.67E-8 * 1 * (T_cold ^ 4 – 0)
T_cold = (1360 / (0.95 * 5.67E-8)) ^ (1/4)
T_cold = 398.62 K
The temperature difference from T_cold to T_hot:
HeatFlux = k * Area * (Thot – Tcold) / d
1360 = 0.8 * 1 * (Thot -Tcold) / 1
1360 = 0.8 * (Thot -Tcold)
Tdelta = Thot -Tcold = 1360 / 0.8 = 1700K
Thot = T_cold + Tdelta
Thot = 398.62 + 1700
Thot = 2098.62K
But there is a problem:
The heat flow of 1360W requires a certain temperature on the warm side. The warm side should be in radiation equilibrium with the sun. If the surface temperature of the warm side deviates from the equilibrium temperature, the heat flow changes, which is necessary for the radiation balance.
For the solution to be correct, the temperature, the size or the distance of the sun would have to adapt to the problem!
You can’t just set a certain heat flow. The heat flow results from the interaction:
Sun heat radiation Thot heat conduction Tcold heat radiation Tspace
I forgot the outgoing radiation from the warm side.
I thought the 1360W is the heat flow into warm side.
Using a solver and the Stefan – Boltzmann constant = 5.67037E-8
I get 383.2797K for Thot and
Tcold = 221.4225K
The way the problem is framed shows 1361W/sq.m entering the hot face. Hence your first answer is correct.
To arrive at any other answer requires many assumptions or more information. For example, the surface temperature of the heat source; the emissivity of the heat source; the orientation of the block relative to the heat source; the size of the heat source; the distance of the heat source to the block of concrete.
The concrete cube has a radiating surface area of 2 square meters.
One square meter at front and back.
The cube will reach thermodynamic equilibrium eventually, with 680 watts per square meter IR being emitted from both front and back.
The cube could be reduced in thickness with same result, so for simplicity you could say there is a thin slab of concrete with same face areas but with thickness resulting in a mass of 1 kilogram
Using https://www.omnicalculator.com/physics/stefan-boltzmann-law
and the concrete emissivity of 0.91 the two square meters of concrete will have a temp of 338.7 Kelvins
(That’s 65.7 degrees celsius)
If the slab starts at 100 Kelvins, with 1000 J/kg-k specific heat capacity, it would take about 238700 joules to heat the slab to equilibrium temperature. 238700 divided by 1360 J/sec equals 175 seconds.
Roughly 3 minutes.
There are about 2400 kg slabs in the cubic meter, so the energy needed to heat the cube from 100K to 338.7K is about 2400 time 3 or 7200 minutes.
A real world heating time is much longer, due to IR loss from the front face almost immediately after heating begins.
bwegher:
Your error here is that you assume infinite thermal conductivity (zero conductive resistance in the cube, where the problem states a finite (and rather low) conductivity value.
Thermal conductivity is not involved.
The entire mass of concrete is at thermal equilibrium with the surrounding space.
Both sides have the same temperature.
There is no “cold” side, the IR radiates isotropically, 680 watts from both front and back.
Go to the SB calculator and enter 2 square meters, concrete (emiss 0.91) and 1360 watts
Calculated temperature is 338.8 Kelvins.
If you calculate each side at 680 watts per square meter, you get the same result.
For example, with the concrete reduced to 0.1 millimeters thickness (paper thin)
the volume of the concrete is now 0.0001 cubic meters, with a mass of 240 grams.
To raise the temperature of concrete from 100 K to 338.8 K with 8.80 Joules per gram per kelvin.
240 grams times 8.8 is 2100 Joules.
2100 divided by 1360 Joules per second equals 1.5 seconds.
The face of the concrete will take 1.5 seconds to reach equilibrium temp.
The next slice will take longer since only 680 Watts are available in that direction, the face of the first slice is emitting 680 watts of IR back to the source.
So 3 seconds times 9999 takes total of 29997 seconds to raise the 2400 kilograms from 100 K to 338.8 K. Rounding off to 30000 seconds that converts to 8.33 hours.
That’s just a first approximation
After at least 8.33 hours the cube will be emitting 680 Watts of infrared energy from both faces.
In a day, the full cube will have a steady temperature of nearly 66 degrees celsius.
Of course thermal conductivity is involved!!!
Like so many others, you confuse static equilibrium (no power transfers) with dynamic steady-state conditions (constant power transfer). This problem is clearly of the second type.
In this dynamic steady-state condition, the cube is gaining energy from one end, and losing energy from the other end. Of course these ends will be at different temperatures, and there will be conduction between the ends.
If you were asked about the steady-state conditions of a long steel bar with one end in boiling water (100C) and the other end in ice water (0C), would you conclude that the bar would be isothermal in the steady state? That is in effect what you are arguing!
This is a perfect example of what I just said about translating text and using logic to set up what must be solved for.
Clearly Willis stated the conductivity of the block.
Clearly this is a limiting factor…a bottleneck.
And this has everyday real world analogous situations.
If one is in a metal box, one will roast to death in the Sun.
But inside a concrete walled box a meter thick, it will be no hotter in the day than at night, and at a certain thickness, the temp will not even vary much over a year…like being in a cave.
Stone castles are a more even temp than metal sweatboxes.
No wonder the world is in trouble.
Erratum. Concrete specific heat capacity is 0.88 Joules per gram K
.88 times 240 grams is 211 Joules K
339-100=239 Kelvins times 211 is 50429 Joules
50429 divided by 1360 J/s equals 37 seconds to reach equilibrium
74 seconds for the next 9999 slices is about 740000 seconds.
That’s 205 hours or 8.6 days for the full 2.4 tonnes concrete to reach equilibrium
(By the time I was ready to post this, Willis and Roy had done basically the same thing. But hopefully my step-by-step explanation will still be useful.)
Ahhh, a classic introductory thermal sciences problem! Let’s go through it step by step.
In steady-state conditions, Qin = Qout.
Qin = 0.95 * 1360 = 1292 W
Qout only comes from radiation from the sun-facing surface (Q1) and the back surface (Q2).
Qout = A * e * sigma * T1^4 + A * e * sigma * T2^4 = A * e * sigma * (T1^4 +T2^4)
A = 1 m^2, e = 0.95, sigma = 5.67E-8 W/m^2/K^4
(I’m using T1 for Willis’ T_hot, and T2 for T_cold.)
Qout = Qin = 1292 W = 1 * 0.95 * 5.67E-8 * (T1^4 + T2^4)
Now for the conduction. In steady-state conditions, the Q2 output must be exactly balanced by the Qcond coming through the cube.
Qcond = A * k * (deltaT/deltaX) = 1 m^2 * 0.8 W/m/K * (T1 – T2) K / 1 m
So Qcond = Q2, and 0.8 * (T1 – T2) = 0.95 * 5.67E-8 * T2^4
I’m a bit rusty on solving general 4th-order equations in closed form, so I iterated and got:
T1 (T_hot) = 383.29 K (= 110.14C)
T2 (T_cold) = 221.42 K (= -51.73C)
To check, I get:
Q1 = 1162.5 W
Q2 = 129.5 W
Qcond = 129.5W
So Q1 + Q2 = 1292 W, matching the incoming solar power.
And Qcond = Q2, balancing the cold-side power.
Using T of deep space = 3K and solving heat transfer for the two faces per Dr. Spencer:
T(hot): 383.6K
T(cold): 221.5K
First, define some terms:
ε = emissivity = 0.95 (constant for all frequencies)
I = incoming absorbed power = 0.95×1360 W = 1292 W
T = temperature (all temperatures in Kelvin)
E = radiative emissions (in Watts)
T_hot = temperature of front (hot) surface
E_hot = emissions from front
T_cold = temperature of back (cold) surface
E_cold = emissions from back
Note: due to the unobtanium sides, there’ll be no net lateral flow of heat across the front or back surface, so the front and back surfaces will both be at uniform temperatures, though not at the same uniform temperature.
Stefan-Boltzman relation:
E = ε × σ × T⁴
σ = Stefan-Boltzman constant = 5.670374419E−8 W/m²T⁴
Simplifying:
s = ε × σ = 0.95 × 5.670374419E−8 = 5.38685570E−8 W/m²K⁴ for our grey-body.
Simplified Stefan-Boltzman relation for our grey-body:
E = s × T⁴
That’s true at both the front and the back surfaces, so:
E_hot = s × T_hot⁴
E_cold = s × T_cold⁴
At equilibrium:
I = E_hot + E_cold = 1292 W
Thermal conductivity “k” of the concrete is equal to 0.8 W / m / degree K, so energy flow from front (hot side) to back (cold side) is:
0.8 × (T_hot – T_cold) W
But at equilbrium, that must be the same as the radiant energy leaving at the rear, so we don’t need a new variable for it, it’s just E_cold:
E_cold = 0.8 × (T_hot – T_cold) W
At equilibrium the power entering and leaving the front are the same, and the total energy radiated and absorbed by the cube are the same, so:
E_hot + E_cold = 1292 W
E_hot = s × T_hot⁴ = 5.38685570E−8 × T_hot⁴
E_cold = s × T_cold⁴ = 5.38685570E−8 × T_cold⁴
We now have a set of four simultaneous equations to solve, for four variables:
E_hot + E_cold = 1292
E_cold = 0.8 × (T_hot – T_cold)
E_hot = 5.38685570E−8 × T_hot⁴
E_cold = 5.38685570E−8 × T_cold⁴
Renaming, for convenience of an online simultaneous equation solver:
u = E_hot
v = E_cold
x = T_hot
y = T_cold
The equations are:
u + v = 1292
v = 0.8 × (x – y)
u = 5.38685570E−8 × (x^4)
v = 5.38685570E−8 × (y^4)
Most online equation solvers like their equations separated by commas, so:
u + v = 1292, v = 0.8 × (x – y), u = 5.38685570E−8 × (x^4), v = 5.38685570E−8 × (y^4)
But this stumped:
https://www.emathhelp.net/calculators/algebra-2/simultaneous-equations-solver/
https://www.symbolab.com/solver/system-of-equations-calculator/
https://quickmath.com/webMathematica3/quickmath/equations/solve/advanced.jsp
Rats. Okay, before I write code to solve this numerically, perhaps someone here knows of a web site which can do it.
You can do it in Excel using the solver. Just tell it to minimise the sum of the squares of the two surface flux imbalances by varying the two surface temperatures. It’s probably also good to add the constraints that the absolute temperatures must be non-negative.
I’ve never learned to use Solver in Excel, but I put the equations into Excel, and defined two error terms, and then manually tweaked the two temperatures until the error terms were very small.
Here’s the spreadsheet, exported as a web page:
https://sealevel.info/hot_and_cold_of_space.html
That should load directly into Excel, but in case it doesn’t, here’s the .xlsx file:
https://sealevel.info/hot_and_cold_of_space.xlsx
Call this the result of a “manual heuristic search”:
T_hot ≈ 383.28 K = 110.13 C
T_cold ≈ 221.42 K = -51.73 C
…and I see that Ed Bo & Rick C PE both beat me to it!
Willis got slightly higher numbers, perhaps because he assumed that all 1360 W were absorbed, instead of 5% being reflected away.
Those are the same numbers that I got, Dave.
Regarding Solver, definitely worth learning. First you have to go to “Add-ins” on the “Tools” menu. Add “Solver” to your menus.
Then you need to set a cell to measure the error, usually the sum of the squares of the distance between the desired and the calculated answers. For more than one variable, you can use the Excel function =SUMXMY2().
Then select your error cell, and choose “Solver” from the Tools menu. Tell it the variables to change, and that you want to minimize the error term. Tell it to calculate, and you’re off. Hang on … OK, I just set up a demo spreadsheet, it’s available here. The variables to change are in yellow, and the error term is in green. Start by changing the yellow cells (which currently have the correct answer) and then run Solver to solve the problem.
Best regards,
w.
Oops, that was Roy, not you. (BTW, both of you should consider my confusion a compliment!)
Hey, thank you very much for the quick Solver lesson! That’s very slick, and very useful, and your instructions were perfectly clear and concise.
Dave Burton February 28, 2020 at 2:42 pm
You’re more than welcome. I see part of what I’m here to do on this planet is to give away whatever knowledge I have … here’s a post on that subject from my own blog, where there’s lots of interesting lunacy, including the story of a most curious oceanic voyage …
w.
If today weren’t a day of mourning, I’d have written that I’m having fun with this. But the news from Princeton puts a damper on it.
Even so, I played with Solver in my antique MS Excel 2003 for a while, and it works very nicely, but I couldn’t figure out how to coax it into running a few more iterations to get maximum precision. Unsettlingly, using Solver on your spreadsheet yielded slightly different results than running it on mine.
So I tried LibreOffice 6.2 Calc, and I’m quite impressed. You have to go into options and select the “LibreOffice Swarm Non-Linear Solver (experimental)” solver engine, but after you do that it apparently runs until it has squeezed out the last drop of precision. It is noticeably slower than Microsoft’s Solver, but its answer is much more exact.
Here’s your spreadsheet, optimized by LibreOffice Calc’s Non-Linear Solver (and I added some more digits of precision to the S-B constant):
https://sealevel.info/WUWT_Puzzle2.html
https://sealevel.info/WUWT_Puzzle2.ods
https://sealevel.info/WUWT_Puzzle2.xlsx
Here’s mine:
https://sealevel.info/hot_and_cold_of_space4.html
https://sealevel.info/hot_and_cold_of_space4.ods
https://sealevel.info/hot_and_cold_of_space4.xlsx
Reassuringly, using LibreOffice Calc’s Solver, the two spreadsheets now yield results (temperatures) which are identical out to a ridiculous nine digits of precision:
T_hot = 383.279628 K
T_cold = 221.422450 K
Try Wolfram Alpha. It will solve your problem!
x = 383.28
y = 221.42
Sure ‘nuf:
https://www.wolframalpha.com/input/?i=u+%2B+v+%3D+1292%2C+v+%3D+0.8+%C3%97+%28x+%E2%80%93+y%29%2C+u+%3D+5.38685570E%E2%88%928+%C3%97+%28x%5E4%29%2C+v+%3D+5.38685570E%E2%88%928+%C3%97+%28y%5E4%29
Or, adding the “>0” constraints:
https://www.wolframalpha.com/input/?i=u+%2B+v+%3D+1292%2C+v+%3D+0.8+%C3%97+%28x+%E2%80%93+y%29%2C+u+%3D+5.38685570E%E2%88%928+%C3%97+%28x%5E4%29%2C+v+%3D+5.38685570E%E2%88%928+%C3%97+%28y%5E4%29%2C+u%3E0%2C+v%3E0%2C+x%3E0%2C+y%3E0
Thanks!
In the past I’ve given thought to similar problems, along the lines of what would a mercury thermometer read in such a situation? And would it be different if I painted the bulb with a different color paint?
But that’s why I refuse to get involved in some of the discussions/arguments about the global warming thing here at WUWT and elsewhere. I can see that the climate models simply don’t work. That is the best starting point of attack. I think even Mosher said something similar a few years ago.
Willis ignores all arguments and just reasserts his faith that CHF = CSR.
His HSR is fake and only used as a meaningless variable to derive his CHF = CSR.
Come one Willis, Surely HSR MUST = CHF too.
WHY NOT???
If you can’t answer this, then concede, if you have integrity.
Zoe, as I said before, please STOP YOUR ASININE PERSONAL ATTACKS. I tell the truth as best I know it. I say I’m wrong when I’m shown to be wrong. Claiming I have no integrity if I don’t do exactly what you want is just you being ugly.
I do say that the heat flow THROUGH the concrete from the hot side to the cold side has to equal the heat flow OUT of the cold side. It’s like a garden hose. The flow rate out of the end of the hose has to be exactly the same as the flow rate through the inside of the hose. This is not contested by anyone I know of … except you.
My mom used to say:
Here’s the rude truth. The fact that I or anyone else disagrees with you doesn’t mean that we’re operating on faith or that we lack integrity. If you look through this thread, you’ll find that not one person agrees with you. Do they all lack integrity?
Nope. THEY JUST DISAGREE. It’s what people do, it’s what scientists do. We disagree. Doesn’t mean we’re bad people. Please stop trying to make it personal.
w.
Stop playing the victim, Willis.
“I do say that the heat flow THROUGH the concrete from the hot side to the cold side has to equal the heat flow OUT of the cold side.”
Yes, I see that you set flow thru = flow out
And what about the flow in = flow thru
“It’s like a garden hose. The flow rate out of the end of the hose has to be exactly the same as the flow rate through the inside of the hose. ”
And what about the flow INTO the hose being equal to what’s flowing through?
You’re not going to escape this and you’re not going to play the victim.
“Play the victim”? STOP WITH THE AD HOMINEMS! Man, you got a nasty tongue on you.
Next, the flow into the hose is NOT the same as the flow through the hose, and the same is true about the concrete block.
Why? Because some is energy is radiated from the hot end of the block, and thus it doesn’t pass through the block. 1,292 W/m2 is absorbed by the hot end. 1,162 W/m2 is radiated from the hot end. The remainder, 130 W/m2, passes through the block and is radiated out the cold end. Steady-state.
To continue the hose metaphor, it’s as though the hose is not screwed tight to the faucet, so some leaks out. So flow in minus leakage at the faucet is indeed equal to flow through the hose.
As to me going to “escape this” … get real. We’re not playing that game. It’s a discussion. Stop the personal attacks. You’re not doing your reputation any good.
w.
Willis,
“Why? Because some is energy is radiated from the hot end of the block, and thus it doesn’t pass through the block. 1,292 W/m2 is absorbed by the hot end. 1,162 W/m2 is radiated from the hot end. The remainder, 130 W/m2, passes through the block and is radiated out the cold end. Steady-state.”
Yup, just as I predicted.
Your hot side is fake hot side that just constitutes a tiny sliver. That tiny sliver for some can’t transfer more heat to the colder end, but is required to send most of its heat back to the source. Thus making the source even hotter. Right, Willis?
A real object at 383K can transfer 383K worth of energy all round, but not your stupid tiny sliver.
“the flow into the hose is NOT the same as the flow through the hose”
Cool self-serving assertion. Why not? Are you saying the water will flow back to its source. Funny, then you only ever had the NET of water coming in. Cool, then you don’t really have 383K, you only have the NET 130 W/m2 = 221K.
Zoe, does it even occur to you to consider the actual properties of a block of stone?
We all have seen, touched, and experienced stone and it’s various properties.
If what you think, and propound in your blog example that started all of this, the inside of a stone castle sitting in the Sun could be used as an oven.
Two watts propagating through the thick stone walls would lead to a vast outpouring of energy inside the walls.
Stone exists in real life…it does not behave as you insist it does in your made up nonsense world.
Zoe Phin wrote, “Willis ignores all arguments and just reasserts his faith that CHF = CSR.”
Zoe, you are as confused as you are rude.
At equilibrium, hot-to-Cold side Heat Flux CHF (that is, heat conducted to the cold side from the hot side) must equal Cold Side Radiation CSR, because if it didn’t then the cold side temperature would be changing.
Zoe Phin wrote, “His HSR is fake…”
No, it is the radiation emitted from the hot side of the tiny Borg Cube (not counting the 5% of incoming radiation which is reflected).
You do understand that a hot grey-body in space will emit radiation, don’t you? And you understand that the hotter it is the faster it’ll emit radiation, right?
Zoe Phin wrote, “Surely HSR MUST = CHF too. WHY NOT???”
No, they are not the same.
The Hot-Side Radiation HSR is governed entirely by the hot side temperature (given that the emissivity is stipulated to be a fixed 0.95 across the entire ER spectrum).
The hot-to-Cold side Heat Flux CHF is governed by the thermal conductivity (a property of the substance from which the cube is made, stipulated to be 0.8 W / m / degree K), and by the temperature difference between the two sides of the Cube.
The only way that CHF could be as high as HSR is if the thermal conductivity of the mini Borg Cube were infinite, instead of 0.8 W / m / degree K.
Dave,
Willis already admitted:
“Why? Because some is energy is radiated from the hot end of the block, and thus it doesn’t pass through the block. 1,292 W/m2 is absorbed by the hot end. 1,162 W/m2 is radiated from the hot end. The remainder, 130 W/m2, passes through the block and is radiated out the cold end. Steady-state.”
An object that rejects 1162 of 1292 W/m2 is not an object with an absorptivity of 0.95. But one with 0.1.
Willis wants to eat his cake and have it too.
Zoe, you are conflating reflection and radiation.
A solid object with an absorptivity of 0.95 REFLECTS 5% of the incoming energy. However, other than that they both involve emissivity/absorptivity, that has nothing to do with how much it radiates. That’s determined solely by
sigma epsilon T^4
w.
Willis,
I thought you’d play this game.
An object that absorbs 1,292 W/m^2 is an object that has molecules moving at 383K equivalent. These molecules WILL bump into neighboring molecules, and so on. But you reject this, and force evacuation of 1,162 W/m2. Your molecules can only bump their neighbors at 130 W/m2 / 221K. Your Hot Side Tempetature is a fake and fraud, and is NO DIFFERENT than had it rejected 90% of incoming energy.
I went overboard and gave you credit for having a 1-molecule thick sliver at 383K, but the truth is that even they don’t exist. They can’t bump their neighbors at 383K, because they must shed 90% of their energy back to the source.
They only have 221K of bumping power, so are they are 383K or 221K ? Come on, Willis, engage !
I need to go buy popcorn before reading any more of Zoe’s dumb azz insulting full-retard nonsense.
Her words seem to suck information out of the Universe, like a text version of a CNN broadcast.
I suspect “Zoe” is some kind of AI robot, pulling technical sounding terms from WikiPedia and constructing sentences using them in a semi-random way. A bit like the way they write fake scientific papers to spook publishers.
That may explain why every reply she give is totally unrelated to the previous question and just starts another unrelated discussion.
Zoe, why are stone houses more comfortable on a hot sunny day that inside a metal walled shed?
What do you feel if you stand in front of a stone or concrete block wall that has been in the Sun all day?
This is not some impossible hypothetical. In fact it is closely analogous to what happens all ove rthe planet and the solar system alll the time, wit the exception of the insulated sides, but there is nothing technically challenging about that. Roughly the same results could be had if instead space shuttle thermal tiles were glued to four sides of the stone cube.
You know those tiles? The ones that get heated to thousands of degrees from the friction of re-entry, and conduct so slowly that the bottom side of the times is not even very hot.
All of the Apollo missions used a capsule with a heat shield as well, but one shaped like a saucer.
Those heat shields get hot and radiate away the heat as they are heating, and then until they have cooled to ambient temp.
You are so focused on believing that photons cannot radiate towards a source of heat has your mind in loopy hallucinations of cognitive dissonance.
I can tell you it is something to behold.
A person incapable of basic logic and reasoning because one preconceived notion must be protected at the expense of rationality if that is what it takes.
” … because they must shed 90% of their energy back to the source.”
—
Oh my goodness, you keep saying stuff like that! No, the energy does not go “back to the source”, it radiates in all available directions, no matter the re-emission temperature. Plus at the shaded end of this block you act like emission doesn’t or can’t occur into space alone, in all available directions, in thermal IR. No, it does not need a colder target object to emit toward, just like the illuminated side does not send radiation back toward its illumination source.
Sunlight striking the surface rocks of the moon and reflecting as ‘Moonlight’ toward the earth obviously did not go, “back to its emitter”. Nor if the sunlight was absorbed by surface rocks and re-emitted later, as IR, for the Moon has an IR output, detectable on earth or in orbit, which IR re-emission did not go, “back to its emitter”.
So drop this nonsense about:
” … must shed 90% of their energy back to the source.”
That does not happen.
Note how Zoe ignored all my questions related to a stone wall sitting in the direct sunshine.
The outside of the wall towards to the Sun gets hot.
The side of a 1 meter thick stone wall that is in the Sun all day will never get hot, even if it is in the Arctic in Summer and the Sun is shining on it all damn day long fro six months in a row.
I feel like going to the Arctic, or Antarctic, and building a one meter think stone wall just to shut her up!
The side of the stone that is one meter from the sunny side will never get hot because heat conducts very slowly through stone.
And the sunny side does not keep getting hotter until it melts because it radiates heat away far too fast for 1200-1300 watts to ever get it to it’s melting point.
In fact, anyone can do this on a bench top using a source of thermal radiation and a block of stone with insulation on four sides.
While the source of radiation is still turned on, anyone can place their hand a few inches from the heated side and feel the heat radiating off the surface.
A FLIR camera can see it.
Nine million FLIR cameras at ever possible angle can see it.
An infrared thermometer can measure it.
And after a year the back side will still only be as warm as thermal conductivity allows for energy to travel through the block of stone.
Notice how Zoe ignores all of my questions except the one in which she could point out she wears expensive undergarments.
Prove it, Zoe.
Zoe, the cube does not “reject” (whatever that means) 1162 of 1292 W incoming radiation.
1360 W of incoming sunlight are incident upon the cube. Of that 1360 W, 1292 are absorbed, and the remaining 68 W are reflected.
Once equilibrium is reached, the block also radiates 129.48574 W from the cold side, and 1162.51426 W from the hot side. But that does not represent incoming radiation “rejected” by the cube. The two sides of the cube have different emission spectra, and both of their emission spectra are very different from the spectrum of the incoming solar radiation.
Dave,
That’s not what Willis said AND
Now you have 1162 – 129 =
1033W flowing “through”
1291W flowing “in”
128 W flowing “out” @ CSR
1162W flowing “out” @ HSR
But Willis said that what flows through must be what flows out.
1033W != 129W
His water analogy is crap?
Zoe, what Willis meant is that 129.48574 W is thermally conducted from the hot side to the cold side, and at equilibrium the same amount of power, 129.48574 W, is radiated from the cold side to space.
The remaining 1162.51426 W (of the 1292 absorbed W) is radiated from the hot side to space.
That’s because at equilibrium:
1. the rate at which energy arrives at the cold face (thermally conducted through the Cube from the hot face) MUST be exactly equal to the rate at which energy leaves the cold face (radiated to space). And,
2. the rate at which energy leaves the entire cube (from the cold face + from the hot face) MUST be exactly equal to the rate at which energy is absorbed by the cube (1292 W). And,
3. the rate at which energy is absorbed at the hot side (1292 W) MUST be equal to the sum of the rates of the two ways in which energy leaves the hot side: 1162.51426 W radiated to space from the hot side + 129.48574 W thermally conducted away from the hot side.
Do you understand, now?
Dave,
“Zoe, what Willis meant is that 129.48574 W is thermally conducted from the hot side to the cold side, and at equilibrium the same amount of power, 129.48574 W, is radiated from the cold side to space.”
But what is conducted to the middle of the block?
I just want acknowledgement that his water analogy is crap.
Zoe asked, “what is conducted to the middle of the block?”
129.48574 W.
129.48574 W is also conducted from the middle of the block.
(It doesn’t matter where along a pipe you measure the flow, the answer is always the same.)
Also, BTW, though you didn’t ask, the temperature at the center of the block will be 302.351039 K = 29.201039 C.
As I pointed out on your discussion thread, that is simply the conservation of energy. Willis has “faith” in the conservation of energy. That seems a pretty good basis for his “belief”.
You waffled about “subtypes” but where not able to say what other energy was being ignored if the conducted flux is not equal to radiated flux as you try to insist.
I think I asked you that 4 times. You just keep ignoring the question.
In Willis’ world thermal equilibrium is impossible. Seriously!
Can he suppose one problem where thermal equilibrium is achievable outside of 0 kelvin?
No he can not.
I feel like I’m in an episode of Black Mirror.
Zoe Phin February 28, 2020 at 12:23 pm
Zoe, I have no idea why you’d think that. This whole puzzle is about what happens at steady-state, after the temperature of the block has completly equilibrated with its surroundings.
So far you’ve seen more than a dozen folks agree that the answer is that steady-state occurs when the hot side is around 383K and the cold side is around 221K, depending on your exact assumptions about emissivity.
w.
Willis,
You’ve redefined thermal equilibrium to mean preserved heat flow, rather than delta T accross an object = 0.
Congratulations! Maybe you also think there are 70 genders? Why not? Redefining words and noun phrases is OK, right?
Actually, Zoe, I never used the term “thermal equilibrium”, so I couldn’t possibly have “redefined” it.
I was very clear that what I was describing is called a “steady-state” condition. This is a condition where after some period of equilibration with surroundings, temperatures no longer change.
Finally, accusing me of thinking there are 70 genders? IT’S A DISCUSSION ABOUT PHYSICS! Stop with the personal accusations.
w.
“after the temperature of the block has completly equilibrated with its surroundings.”
The cold end is “surrounded” by the hot end, but it refuses to accept energy from it because it has to send HEAT to space, a substance that doesn’t accept HEAT in order to cool the hot end.
Remember Newton, Willis?
He said an object in motion stays in motion unless acted upon by an external force.
Can you name the scientist that first invalidated this and confirmed that molecules in motion reduces their motion by exerting their own force (EM radiation)?
Zoe Phin February 28, 2020 at 2:08 pm
I can’t. My head explodes. I just can’t. Do I remember Newton? Can’t be Isaac, wrong field of physics. Huey Newton? Fig Newton? Gravitational Constant of 6.67430×10−11 Newton⋅m2/kg2?
Pass … I can’t.
w.
Zoe what the hell are you talking about?
Every comment you make is incoherent.
Maybe if you stopped making stuff up that no one said and inserting those words into other people’s mouths, you could focus on the logic and the scientific principles involved in the discussion.
Why are the people who act like you always the ones who do not seem to have any idea what they are talking about, but act like they are the only ones who know anything?
I should just ignore you like most others have…but I am not able…you are incredible rude and irritatingly obtuse.
Just give up and ignore her Willis you can’t win this, it is because classical physics breaks down there is no actual classical physics that heat should flow. It’s an observed effect from a physics outside classical physics. Temperature is a made up observational statistic there isn’t anything fundemental about it.
It’s the same problem with trying to argue with someone that there can’t be a point on earth that gravity doesn’t exist. All the classical gravitational laws are observational there is no way to actually prove it.
Any physics uni student who knows the basic background can troll indefinitely because they know there is no actual proof you can use, they just have to keep shifting the background which is what she is doing.
Initially I thought she was just being dense but you look when I asked her about instantly exploding points on the block. She realizes she is in trouble via observation because the law of averages says if things aren’t forbidden they will occur and so we should have instances of instantly exploding pieces of the block where heat decided to pool. She would then be in the situation of actually having to explain why something doesn’t happen rather than just deflect it.
She is a troll it is best to just ignore her and there is no “proof” you can use it’s just classical physics at it’s finest.
LdB February 28, 2020 at 11:33 pm
LdB, I’m not writing to try to convince or educate her. I write for the lurkers. For every commenter I figure there are ten or more lurkers who read but rarely write. They are not set in their ways as she is, and they are not immune to facts as she is. I write to dissuade them from believing her nonsense.
Regards,
w.
I fear all you are doing is giving her the limelight which is all she did it for in the first place. You get this a bit with uni students when they work out just how broken classical physics is and they can play games with those less educated.
She is a young student because she fell into 2 traps
1.) With the cold end she made the mistake of wanting to have matter that sets up the problem that the cold end had to know matter existed. She has not dared touch that anymore because it ends the game.
2.) As per above if you don’t have a gradient then the energy can clump anyway it likes so there is nothing to stop it melting pieces off. Her only answer she could give was she wanted to see data.
Personally my advice ask Anthony to delete the article and deny her the two minutes of fame.
Willis:
Like many over at PSI, Zoe confuses the case of static equilibrium (no power transfers) with dynamic steady state conditions (constant power transfers). This means that she, like the others, goes off the rails before she even gets going.
To anyone familiar with basic thermodynamics and heat transfer, it should be obvious that this problem is the second case of dynamic steady state.
I’ve said it many times: reading crackpot sites like PSI (or Alex Jones, Sott, Slanderin’ Sou from Bundangawoolarangeera, David “Avocado” Wolfe, etc.) actually seems to kill brain cells.
(However, I might be confusing cause and effect.)
It’s a resistor
Sorry but I haven’t read all comments, so I don’t know if anyone has already said this. And Willis, you have triggered a lot of comments with this one.
The outward radiation on the sunny side. plus the transmission rate through the block, must equal incoming radiation. We’re assuming no reflection, presumably.
The rate of transmission through the block is proportional to the temperature gradient.
The sunny-side temperature and the temperature gradient give you the temperature at the dark side, and the outward radiation there must equal the transmission rate.
Three linear equations for three variables. All you have to do is put in the numbers.
One interesting thing is that – depending on the parameters – the dark side doesn’t have to be very cold. The higher the conductivity of the material, the cooler the sunny side is and the warmer the dark side is.
My solution:
– Cold face: – 53.05°C (219.95 K)
– Hot face: + 108.16°C (381.16 K)
Checking:
– Heat radiated cold face: 0.95*5.8E-8*(219.95^4) = 128.96 W
– Heat radiated hot face: 0.95*5.8E-8*(381.16^4) = 1163.05 W
– Heat tranmitted by conduction: (108.16 + 53.05)*0.8 = 128.97 W (= heat cold face)
– Total heat radiated: 128.96 + 1163.05 = 1292 W = 0.95 * 1360 W (= heat received from the sun)
Is it cheating to assume that any initial difference in T at either end will cause the cube to spin and this the whole thing can be simplified to a single face of 2 square metres?
Well, of course it is. But it makes my sums easier.
Before doing the numbers, I’d like to take this as a learning opportunity for myself, to talk about it in words first. Again, I’m attempting to learn, so tell me where I go wrong:
* The concrete block has one side (hot side) with a constant maximum energy input, and one side with a constant maximum rate of cooling.
* The power of energy going in cannot ever equal the power of energy radiating out, because there is ALWAYS energy coming in on one end (no cooling at the surface area), and ALWAYS energy going away from the other end (constant cooling at THAT surface area).
* The physical structure of concrete allows it to transfer energy from hot side to cold side ONLY at a given rate — this is the conductive heat transfer, and it has a particular value for concrete.
* Conductive heat transfer is the rate at which the hot end can supply energy THROUGH the block, .. TO the surface area at the cold end.
* If the cold end is ALWAYS cooling, then the cold end can never achieve the temperature of the hot end. Rather, it will achieve the highest temperature possible with the given amount of conductive heat transfer offset by the constant cooling rate. This will always be a lower temperature at equilibrium.
* Now the cold end, at the lower temperature will now be radiating energy at a lower rate than the hot end. This cold-end radiation, though, is NOT the conductive heat transfer. Rather, the conductive heat transfer is the CONSTANT quantity of energy delivered to the cold end to MAINTAIN its radiating temperature to the cold ambient space around it.
* Input flux is greater than conductive heat transfer … is less than output flux … is also greater than conductive heat transfer. The hot-end higher-energy area transfers some of its value constantly to the cold-end lower-energy area to MAINTAIN that lower energy, which enables the value of the cold end’s temperature at equilibrium.
* A watt is a joule per second. Joules-per-second constantly occurring for a number of seconds delivers a number of joules to the cold end, while the cold end constantly looses joules per second cooling. The conductive heat transfer overcomes the cold end’s cooling enough to maintain the cold end’s temperature, which temperature itself is higher than the conductive heat transfer maintaining it.
* The cold end, now at its lower-than-hot-end temperature COULD now act as a source of heating for, say another concrete block, with its own conductive heat transfer, which would be a smaller value than the first block’s conductive heat transfer.
* The conductive heat transfer of the first block is what maintains the cold-end temperature. THAT cold end temperature has its own flux, but this cold-end flux is NOT a conductive heat transfer or a source of conductive heat transfer, until we know what is next to it.
Okay, where did I go wrong? Thanks.
Yours truly,
Either-making-a-fool-of myself-or-providing-useful-insight
(you can call me “foolsight” for short)
Thank you Robert.
Since you ask, I was wondering about this:
“The power of energy going in cannot ever equal the power of energy radiating out, because there is ALWAYS energy coming in on one end (no cooling at the surface area)…”
The hot side absorbs 95% of the incident radiation, and heats up.
Thermal energy begins to conduct through the block, and conducts faster as the heated side gets hotter.
But as soon as the front side absorbs any energy, it begins to emit radiation at 95% emissivity.
And this also increases as it gets hotter.
So the front surface is cooling by conduction through the block, and by radiation from the surface.
No?
Nicholoas M, regarding your February 28, 2020 at 3:26 pm comment,
that sounds logical to me.
The temperature will vary at every point on and in the block. On the surface of the block facing the sun, it will be warmer in the centre of the square and cooler at the edges. So the formulation of the question, with its assumption of constant surface temperatures over a single surface, is incorrect.
You could assume that each surface of the block was composed of perfect thermally conductive material, and totally thermally insulated at the edges from the other surfaces. Then you would have to know the thermal resistance between each of the surfaces. Hard to do without a computer.
Why do you say it is warmer in the center?
The side pointing to the sun will be hotter. The sides pointing away will be coldest. Therefore the edges of the sun-pointing side will be nearest to the coldest sides, so the temperature there will be colder, due to the shorter thermal pathway. If the temperature at the edges of the sun-pointing side is /are colder, the temperature in the middle of the sunpointing side must be warmer.
If the material of which the cube was constructed had infinite thermal conductivity, then all would be the same temperature.
” Therefore the edges of the sun-pointing side will be nearest to the coldest sides, so the temperature there will be colder, due to the shorter thermal pathway.”
No…the surface is flat and the sides are insulated. The center of the front is the same distance from the center of the back as the edges of the front are from the edges of the back side.
The entire front surface is one meter from the entire back surface.
If the sides were not perfectly insulated then yes.
Part of exercises like this is to pay attention to every part of the problem as described.
https://www.youtube.com/watch?v=AiZWFrb76pM
Here we see a video of a radiator being heated.
The source seems to be about 60C,
so we’ll say it is 333K
The room temperature is, say, 25C or 298K
Now remember according to ideological mathematics:
CHF = CSR
CSR = 5.67e-8*(333^4-298^4) = 250 W/m2
Well, what do we see in the video?
The output temperature = input temperature, despite
the huge size difference of the output.
Tcold = Thot !
CHF == ZERO !!!
But not according to ideological mathematicians
Because CSR MUST EQUAL CHF
Set k to 1 ( we don’t know or care what it is )
CHF = k (333 – Tcold) = 333 – Tcold = 250
Tcold = 63K
No wait, we can’t do that (I did it on purpose). Let’s drop
the room remperature:
333 – Tcold = 5.67e-8 * Tcold^4
Tcold = 214K or -59C
Is that what we see? NO!
Why do people think Boltzmann and Planck were idiots who couldn’t notice CSR=CHF?
Just kidding, k is important to know.
Steel radiator has k between 12 and 30
The result is 296K to 314K
But we see the temp go to virtually 60C, or 333K.
“So the question is: at steady-state, what will be the temperature T_hot of the hot side and the temperature T_cold of the opposite cold side?”
Well, according to the Apollo 13 fraud, freezing cold throughout.
Please show the solution when the hot and cool sides of the block are just thin layers and have vacuum in between them, and do not forget to include back radiation from the cool layer.
Also show the solution when a VIS-transparent & IR-absorptive glass layer is put in front of the warm surface with a small vacuum gap in between, again including the effect of back radiation on the sun-ward layer from the new glass layer. In this case, it is a concrete block again.
Please show the solution.
Hey Johnny,
I loved that stunt where you rode down an escalator in a shopping cart full of bowling balls and glass bottles!
In the final stationary state T2=T1=T.
Then, the irradiated surface of size A, S1, receives G = alpha * 1360*A Watt and loses by radiative loss L1=2*pi*eps*sigma*A*T^4 Watt and – by conductive loss to the interior – the same amount, which in turn is radiated from surface S2, L2=L1. The thermal conductivity (together with the specific heat) just determines how long it takes until the stationary state is reached.
Hence, with alpha = eps (= 0.95 T) = (alpha *G / (4*pi*eps*sigma))^0.25 = 212 K.
R. F.
People have a hard time understanding what conduction is.
The conduction formula = KA(Th-Tc)/L
If A is in the (x,y) dimension
L is in the (z) dimension
K is in units W/(m*K), and m is also in the (z) dimension
In the conduction formula: K/L ~ 1/m /m = 1/m^2
The conductive m^2 is (z) dimension divided by (z) dimension = still (z) dimension
Conductive m^2 is different from Radiative m^2.
Do you not see that A is not in the same dimensions as K/L ???
Willis et al believe they can compare flux through the (x,y) dimension to the (z) dimension.
This is silly.
Boltzmann and Planck found that
CSR = εσ(Th-CHF*L/K)⁴
And CHF is free to approach ZERO. When it does,
CSR = εσ(Th)⁴
Thermal equilibrium! SB Law!
https://en.wikipedia.org/wiki/Black_body
“A black body in thermal equilibrium (that is, at a constant temperature) emits electromagnetic radiation called black-body radiation. The radiation is emitted according to Planck’s law, meaning that it has a spectrum that is determined by the temperature alone (see figure at right), not by the body’s shape or composition.”
But hey why do real physics, when self-deceptive rhetoric and ideological mathematics will do?
Willis never specified that the concrete block was equivalent to a black body in equilibrium. In fact, by stating that there was conduction through the block and an exposed, non-insulated rear surface away from the radiation input side, he gave fair warning that IN NO WAY could the concrete block be modeled as a blackbody!
Gordon,
He models a 0.95 * Blackbody. Basic laws don’t change. Sheesh, if wikipedia doesn’t mention a 0.95 * Blackbody. I must be wrong, right? How pedantic.
You posted: “He models a 0.95 * Blackbody.” No, he does NOT.
It is you who is attempting to argue a blackbody has relevance to Willis’ postulated problem. It simply does not.
And there is no purpose of me trying further to gently convince you of that. Good luck.
A blackbody will emit: sT^4
A greybody will emit: esT^4
where e = [0..1]
Are there any others laws evoked?
Are you arguing that he doesn’t use a grey body therefore the emission law is completely different, say sT^3-4T^12+PI or sT^2-4ln(T), or whatever?
What are you saying?
where does Boltzmann and Planck refer to CHF ? That is just you own BS mixed up derivation from assuming YOUR block was cooled by SB radiation when there is nothing to say that is the case.
You are still unable to address what I’ve pointed out at least six times now. You made a false assumption.
Do actual blocks of concrete in space do real physics Zoe…or do they whine about units and pretend they know what they are talking about?
It’s the same A. The magnitude of the flux is inversely proportional to the length, since the longer the bar, the greater the thermal resistance.
Wrong again. m is the dimension of A divided by the dimension of z. L^2/L in dimensional analysis.
The more you come out with the more you show your ignorance and basic lack of understanding of any of this. But don’t let me stop you, this is fun.
Greg,
“Wrong again. m is the dimension of A divided by the dimension of z. L^2/L in dimensional analysis.”
Why would you divide a face in the (x,y) by z. What does that yield? And you forgot K.
You’re forgetting that the flux equivalent is:
Q/(A*dt) = K(Th – Tc) / L
Now both sides are W per m^2
But the two m^2 are in completely different dimensions.
“Why would you divide a face in the (x,y) by z.”
You are not dividing a face by z. You are dividing the power by area to get the flux and multiplying by the length, to derive a property of the material which is not a function of an object’s shape or size.
You could say Wm/K/m^2 which is what it really is but the m on top and bottom cancel, so it becomes W/K/m. The ‘per metre’ is not a linear length it is the result of dividing an area by a length. Dimensionally it is L-1 i the units.
They’re not, since K ( usually k ) includes “per unit area” in its full form: Wm/K/m^2 . That is why you divide by length and multiply by temperature. What is left is power / area.
“You are dividing the power by area to get the flux and multiplying by the length, to derive a property of the material which is not a function of an object’s shape or size.”
(Q/(A*t)*L) = K (Th-Tc)
Now you LHS is meaningless as it divides z-dimension by (x,y).
So you’re right (LOL), you derive a meaningless nonsensible property.
“They’re not, since K ( usually k ) includes “per unit area” in its full form: Wm/K/m^2 . ”
No it doesn’t. k or K is in W/(m*K).
“In the International System of Units (SI), thermal conductivity is measured in watts per meter-kelvin (W/(m⋅K)).” — Wikipedia and every place else.
You have no idea what you’re talking about.
I’m not saying anyone every writes it that way ! I’m trying to explain the derivation of the unit and what it means, to explain to you that it is division by a length at all.
Energy flux of 1361W/sq.m through hot face. Energy is conserved so, at steady state, 1361W/sq.m must exit the cold side. That requires a cold side temperature of 399K ([1361/.95/5.67E-8]^.25). The hot side temperature must be at 2100K (1361/0.8/1*1+399) to achieve the steady state heat flow through the block of concrete.
The hot side also radiates, no?
Yes of course it radiates. But the problem shows 1361W/sq.m being absorbed by the hot face. Radiation is, by definition, a result of THE electro-magnetic field. It is singular and unidirectional at any point in space and time. A proper solution requires solving Maxwell’s equations for the electrical-magnetic field that the object exists in. That requires details on the heat source and more detail on the orientation of the concrete block. Approximate solutions can be achieved by applying view factors and using radiant heat transfer equations; again requires details on the heat source and the orientation of the block relative to the heat source..
Nonsense and gibberish.
With all due respect.
To properly account for conservation of energy (actually, in this case, power) you need to account for radiation off the front surface of the block at its equilibrium temperature.
Come on, Nicholas and Gordon! Everyone knows that a black asphalt parking lot in the tropical noon sun, receiving 1360 W/m2 of solar input doesn’t radiate away any power at all…
(Need I say /sarc?)
If the asphalt is receiving 1360W/sq.m then that is the end of the story. The radiation energy is singular. It occurs by virtue of THE electro magnetic field – singular. There is only ONE EM field no plethora of fields that are adding or subtracting.
Are you seriously arguing that a black parking lot in the hot sun does not radiate away energy while it is in the sun??? You don’t need any sophisticated sensors to know that it does — you can feel it yourself!
Of course you can add the effects of multiple sources together. If I shine two lights on you, the EM field that reaches you will be stronger than if I only shine one light on you.
This is basic, basic stuff!
I think it was sarc
Yes, and I guess all those infrared cameras actual image unicorn dust instead of RADIATED thermal energy, particularly in the absence of any incident radiation.
/sarc
The limiting cases for this problem are:
When the thermal resistance of the concrete is zero (high conductivity concrete) then both faces have the same temperature which energy balance reveals to be 330.9K.
When the thermal resistance is infinite then and
and 
I will choose an intermediate value for the thermal conductivity of concrete which is well within the various values for concrete.
which is well within the various values for concrete.
Now I have to iterate between two equations, which are energy balance at the two faces:
1.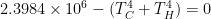
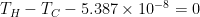
and
2.
I started with and iterated between the two equations six times until I was bored….
and iterated between the two equations six times until I was bored….
Oops….
1.

and
2.
I started with and iterated between the two equations six times until I was bored….
and iterated between the two equations six times until I was bored….
For some reason I cannot correct a typo in eq. 2….It is missing a factor of in the second term.
in the second term.
For some reason this software won’t let me correct the error in equation 2.
1.
and
2.
I started with and iterated between the two equations six times until I was bored….
and iterated between the two equations six times until I was bored….
Well, I read through a bunch of comments and did not see one person follow the instructions and give an answer as one would on a test and also show work or, where I went to school anyhow, you get a zero in this type of class.
Some people said things that were probably true but unhelpful.
Some told how to set it up but gave not one detail that would instruct anyone who does not already know how to do it.
And some said stuff that was either mystifyingly abstruse, perplexingly opaque and also likely wrong, and just plain made no sense.
Others pretended that it is impossible to have such a situation, which in the case of a perfect insulator on the sides is likely true, but there are very good insulators and anyone who knows this stuff knows how to calculate a limit or so I would think, so it should be easy to say what would happen as the insulator approached being perfect from a state of being pretty good.
We can put block of concrete with the described characteristics in orbit and measure it…using some ultralow density stuff like space shuttle tiles or aerogel or some crap like that.
It seems that is the simplest way, despite the assembled wit and wisdom of the commenters/comments I was able to read, before becoming bored to tears, anyone not already an eggspurt on this topic could arrive at an answer, given the condition that putting some concrete on the very first Virgin Galactic flight would be easier than getting the required edumacation in thermodynamics.
It is not impossible to show your work here.
Write it down, take a picture, post it on photobucket or twitter and post a link.
I have done stuff like that a thousand times.
Nicholas:
Several of us posted step-by-step analyses of the problem just as we would for a technical class, all reaching the same answer. I have taught technical subjects at the university level, and I would give full credit to the others whose analyses matched mine.
Thank you Bo…Yeah, I wrote this after reading a few dozen of so at the very beginning.
Maybe less.
I did see that several people has already done the exercise using what appears to me to be the proper logic.
I often do not read all through a thread before commenting.
Especially when the site is acting up.
(For a while there, maybe a week or more, comments were posting immediately and the page would return to the part of the thread one posted their comment in.
Now suddenly comments are posting after a delay off variable length again, and after clicking the blue button the page returns to the top and stays there.
Had to find one’s way back sometimes…)
Solving word problems, as they used to call them in grade school or high school math or algebra classes, is very difficult for a lot of people, even in advanced University science and math classes.
Willis,
You really hooked me today. I found an online solver Wolfram Alpha. Using t1 for the hot face deg K and t2 for the cold face deg K, and expressing the two simultaneous equations to solve using only the two temperatures, here is the result as a link.
https://www.wolframalpha.com/input/?i=solve%7Bt1%5E4*0.95*5.67E-8%2Bt2%5E4*0.95*5.67E-8%3D%3D1360*0.95%2C%28t1-t2%29*0.8%3D%3Dt2%5E4*0.95*5.67E-8%7D
Just like the Mathematica solver you used, it gives the desired answer at the end of the list.
I hope this works as a link, as I suppose it should.
Nice, Dave, the link worked perfectly. I have standalone Mathematic on my computer, same answer, but it doesn’t give the cool graphic showing the two real solutions.
w.
wow, I did not realise that T^4+T^4 was a closed loop though I probably should have recognised the similarity to x^2+y^2=r^2 , a circle. With the forth powers I suppose that’s like squaring the circle and it ends up making a … squared circle.
When I did my graphical solution below, just plotted the intersections and did not scan out to a scale where I would have seen the full form. Nice.
Th = temperature of hot side (uniform across the face)
Tc = temperature of cold side (uniform across the face)
Qh = heat radiated by hot side = ε σ Th^4
Qc = heat radiated by cold side = ε σ Tc^4
Qs = heat from sun = 1370
Qi = heat input to cube = ε Qs
Qt = heat conducted thru the block = k(Th-Tc)/t
t = thickness (from hot to cold side)
(All values for Q are W/m^2)
(the front side and the back side will be at constant temperatures across each surface)
There are two constraints relating these two variables.
1) the heat conducted through the block must equal the heat radiated by the cold side
Qt = Qc
k(Th-Tc)/t = ε σ Tc^4
2) the total heat in = total heat out
Qi = Qh+Qc
εQs = ε σ Th^4 +ε σ Tc^4
Note that emissivity drops out here, so
Qs = σ Th^4 +σ Tc^4
Two equations, two unknowns — so just solve.
OK, OK, that is much easier said than done! Solving either equation for Th and substituting back into the other equation gives nasty combinations of Tc, Tc^4 and/or Tc^0.25. I am not sure there is an analytic solution, nad right now I don’t have time to do a numerical approximation. >/i>
Partial credit for anyone who first defines their terms.
No more than partial credit for anyone who does not.
Both are regardless of correct answer.
(Getting the logic part correct is 75% of the possible points.)
I think the “right answers” are
383.6 °K for the lit side, and
183.7 °K for the dark side. Why?
Assuming Stefan-Boltzmann constant of 5.67×10⁻⁸, then the lit side will be reflecting 5% of 1360, leaving 1292 W absorbed. It will come to asymptotic balance emitting 95% of that, or 1227 W. This corresponds to 383.6°K or 110.4°C.
Likewise, the net transmitted thru also asymptotically approaches (absorbed – reemitted), or 64.6 W. Using σ (SB constant), this corresponds to an emission temperature of 183.7°K or –91.8°C.
The reason one doesn’t have to figure the thermodynamic conduction part is because of the unobtanium wrap. Over long enough time, thermal balance is in steady state.
⋅-⋅-⋅ Just saying, ⋅-⋅-⋅
⋅-=≡ GoatGuy ✓ ≡=-⋅
GoatGuy, your solution doesn’t check out. You have the hot side radiating 941 W/m2 and the cold side radiating 61 W/m2. This only adds up to 1,002 W/m2, but the block is absorbing 1,292 W/m2.
No bueno.
Sorry,
w.
Graphical solution:
gnuplot> Th2(x)=(1360/sigma-x**4)**0.25;
gnuplot> Th1(x)=(sigma*epsilon*x**4)/k+x;
gnuplot> plot Th1(x) tit “(sigma*epsilon*x**4)/k+x”,Th2(x) tit “(1360/sigma-x**4)**0.25”
take intersection where both values are +ve : 221.4 , 383.34
There are 3 scenarios under which Tcold will not equal Thot, i.e steady-state gradient condition.
1) k increases thru the block
2) A increases thru the block
3) There’s radiative/conductive/convective leakage through the 4 walls.
Willis defines exclusion of all these things, and therefore his solution is invalid. He never researched what would create a gradient.
Of course his other comments reveal there is no gradient, only a small sliver at 383K, while the rest is 221K.
It’s fantastic pseudophysics. I’m impressed how he keeps this game going without any reference to literature or experiments.
Plug in given Th into CSR = CHF assumption to get Tc, but ignore HSR = CHF, then 90% of energy from HSR goes back to the source (making it hotter?) while you pretend aborptivity was still 0.95 and albedo is 0.05. LOL
You need to read all of Willis’ comments to understand this.
You have it exactly backwards:
“This” (meaning whatever what you wrote is purported to mean) can never be understood, at least not in this century.
Perhaps in several millennia your particular brand of delusional thinking will be understood.
But it will take at least one or two rounds of Twenty Questions.
Zoe says “There are 3 scenarios under which Tcold will not equal Thot”
Actually, there is 1 scenario under which Tcold WILL equal Thot” — the thermal conductivity, k, is infinite.
Or unobtainium is covering 5 sides
I forgot a 4th: mass variation.
To Zoe,
I posted fhis comment on your site, please please stop trying to re-invent physics and math.
READ “Transport Phenomena” by Bird Stewart and Lightfoot
If you don’t like second order differential equations don’t bother.
It’s rude to send people on wild goose chases. If that book had the solution to what we’re discussing you’d be more specific with the section, pages, etc.
Haha!
The rudest person all day gets her knickers in a twist over being asked to do some research!
touche`
Nicholas, my expensive lingerie doesn’t get in a twist.
I’m not going to read a giant textbook just because someone says so. If they find something useful and applicable I will gladly read that chapter.
You not feeling like doing something does not make it rude.
You could have just asked for a summary or a page number.
What is it called when one takes the less than polite alternative?
Nicholas,
You know nothing of our previous conversation, but you butt in with your opinion on etiquette.
Do you have any self-awareness of your own rudeness?
Yes, plenty.
I am rude to people who deserve it.
“Treat me nice, I’ll treat you better.
Treat me bad, I’ll treat you worse.”
I extend “me” to include anyone for whom I have respect from prior interactions.
If you treat them badly, I’ll treat you worse.
And BTW…you know nothing of what I do or do not know regarding previous interactions.
And that is irrelevant in any case to calling out hypocrisy.
This is a science site.
Getting things correct is a virtue unto itself, so it is not a rudeness to say that someone who is wrong, is wrong.
It is simply the truth.
* To quote the great D.B. Stealey, wherever he is.
I wish he was here right now.
“Of course his other comments reveal there is no gradient, only a small sliver at 383K, while the rest is 221K.”
Zoe, how do you think the pressure in a hose varies along its length while water is flowing. Is it all at tap pressure with just a “slither” atmospheric pressure just before the outlet, or is there a pressure drop ( a gradient ) along the hose?
Willis said that which flows thru the hose must leave the hose. But when it comes to entering the hose (HSR), for that we can just reject 90%. But it already entered!
As you do EVERY SINGLE TIME you avoid answering the question and reply with some diversion.
All the solutions here are based on flux in = flux conducted = flux out. What is your 90% “we” can reject ?
Greg, pay attention,
The flux in is spread out to both sides to get flux out.
Flux in = HSR Out + CSR Out
Does that happended with water?
First Wilis stipulates that all water entering must leave. Then it turns out that only a small fraction of it leaves, and the rest can’t go through and spills out where it should come in.
He has 1000 “W”+ of water going through but only ~130 “W” (I forget the numbers) leaving.
In fact he’s got more water coming out the way it should be going in then goes through it.
If you can’t make sense of this, it’s because Willis’ analogy is crap and he contradicts his core assumption, but I guess it’s all OK because radiation is “different” now then his water analogy.
Zoe, the water hose was an ANALOGY, and like all analogies it is not perfect. My main point was simple.
The water flowing THROUGH the body of the hose must equal the water flowing OUT of the far end of the hose. That was the only reason I brought it up. So forget anklebiting the damn analogy and let’s get back to the actual question
You keep claiming that the energy flowing THROUGH the block is NOT equal to the energy flowing OUT of the far side of the block. Nobody here, including myself, has a clue how that would be physically possible.
If more energy is flowing THROUGH the block than is radiating OUT of the far side of the block, where is the extra energy going? And if more is radiating OUT of the far side than is flowing THROUGH the block, then where is the extra energy coming from?
You make the same bizarre claim on your blog with respect to geothermal heat. You claim that somehow a geothermal energy flux of a few tenths of a W/m2 is sufficient to heat the earth surface to 15°C, which is losing energy by radiation at about 380 W/m2 or so.
Madness. I’d hoped that if I weren’t the only person pointing out this facially incorrect claim, you might notice … seems I was wrong.
Now, please don’t take this and start off in another direction. Just answer the questions. Here they are again:
If more energy is flowing THROUGH the block than is leaving OUT the far side, where is the extra energy going? And if more is coming OUT of the far side than is flowing THROUGH the block, then where is the extra energy coming from?
Answer, please. No handwaving. No misdirection. No personal attacks on me. No invective.
Answer, please.
w.
“Nobody here, including myself, has a clue how that would be physically possible.”
Of course they don’t. They distegarded all my arguments and spouted their ideological math.
The CHF in the sun’s photosphere is TINY compared to its CSR.
It doesn’t matter to me that ideological mathematicians disregard observations and claim its not possible within their ideology.
I’m interested in science, not ideology.
My solution differs slightly from papijo’s.
I used 5.670367 *10^-8 for the Stefan-Boltzmann constant.
Hot side: 383.28 K
Cold side: 221.43 K
So the flux to the cold side is 129.48 W
After some iterations the radiation of the hot side was set to 1162.5, that leaves for the cold side 129.5 W, that agrees well with its flux.
Wow!! All this is way over my head.
What I take from this exercise is the following:
This problem relates to a stationary cube.
The cube is composed of a uniform and constant substance.
The properties of this substance is known
The cube has perfectly flat and uniform surfaces.
Only two surfaces of this cube are interacting with the environment.
A constant source of external energy is applied one suface.
Sounds simple right?
I am left wondering how the same problem can be applied to a spinning globe with an uneven surface composed of wildly differing materials. This globe is then regularly subjected to variations in orbit around a variable energy source. The orbit has variations both in distance from the energy source and variations in the angle of incidence to the energy source. All the while, the globe is shrouded by a complex mix of gaseous fluids which interact both with the surface of the globe and the incoming heat source.
If I don’t believe Al Gore I am labelled a denier.
Elementary my Dear Watson.
I didn’t even try to kid myself that I might be able to solve this one – IQ limitations and all that jazz.
As an aside, the solution is the same no matter how the 1,292 W/m2 is added at one side.
It could be a star far away applying 1370 W/m^2.
It could be a 394 K blackbody surrounding one face applying 1370 W/m^2.
It could be an IR laser spread uniformly over the surface 1370 W/m^2.
It could be a 1292 W electric heater on the surface.
This realization may help squash a few red-herrings like ‘heat back to the source’. In every case, we are assuming a fixed input such that 1292 W/m^2 is absorbed. The exact nature of the source is immaterial to the problem at hand.
“As an aside, the solution is the same no matter how the 1,292 W/m2 is added at one side.”
Actually, no. One has to consider the view factor for the radiation emitted off the front face. If the view factor is ESSENTIALLY that of a hemisphere of deep space, then front face radiation is to an “environment” that is essentially at 3 K over 2*pi steradians.
If the view factor is that presented for the front face of the concrete block at, say, 100 km altitude orbiting a brown dwarf having a diameter 200,000 km and looking onto a “surface” temperature of 700 K, the front face radiation “environment” is essentially 700 K over ~2*pi steradians.
W/m² K °C
1.360,00 398,62 125,46
0,95*P 1.292,00 393,54 120,38
1.292,00
T2*Ϭ4 129,49 221,43 -51,73 = T2
T1*Ϭ4 1.162,51 383,29 110,13 = T1
(0,8) * (T1-T2) 136,30 161,86
0,95 * Pi = 1.292 W/m²
Tcold = -51,73 °C = 221,43 K
Thot = 110.13 °C = 383,29 K
I posted a video to a youtube video that debunks my critics. Not surprisingly no one responded. No references to any literature.
I very much enjoyed the insults. I do not feel that my best criticisms got an intellgient response.
The best part is that I have all the references I need from the scientific literature to back up what I said. I will unroll it all on my blog over the year, and I will continue enjoy being attacked by ideological mathematicians posing as physicists.
Youtube is the most inefficient way to communicate that sort of thing. Why not just write it down.
Oh hang on, it’s going to take you a year to do that ?
You should be able to do it in a couple of paragraphs. I know, why not make it ten years. Then you can hope that everyone will have forgotten by then and you will never have the embarrassment of having to eat crow pie in public after having insulted everyone in sight.
Watching a thermally imaged radiator in real time action debunk the theories espoused here is a bad way to communicate?
Great. Thanks for confirming your ideological math trumps experiments. Not surprised denial runs deep.
Yes, it’s a bad way to communicate because it is totally different to what is discussed here and what is in your own version. The heat loss in the radiator is predominantly due to lateral losses which were expressly set to zero in both exercises.
You can waffle for 20min on a video and not say what can be put down in a few lines written and understood in two min. Yes, it’s a bad way to communicate.
I did not mention a single bit of maths in that last comment, “ideological” or otherwise.
Can you explain what “ideological math” is ? It sounds like a new and interesting field of study that I seem to have missed.
Greg,
“The heat loss in the radiator is predominantly due to lateral losses which were expressly set to zero in both exercises.”
Exactly, the radiator had more disadvantages than this block, and it still reached thermal equilbrium (CHF=0).
You lack critical thinking skills.
Zoe, among your many wrongnesses, you seem to have a very poor understanding of language, and what certain words mean.
To name a single example, you have no idea what the word “debunk” means.
“I posted a video to a youtube video that debunks my critics. Not surprisingly no one responded. No references to any literature.”
Psst a video can not do this.
Psst references to literature cannot do this.
Simple question:
Why do my critics convert all of conduction to radiation on the cold side, but on the hot side they reject most radiation becoming conduction?
Why the hypocrisy?
Why does the cold end decide to reject most of the energy and cause the hot side to return most of the energy that could’ve went into heating it?
That energy returning from the hot side back to the source must make it hotter, no?
Not only does the source have its own energy but its getting backenergy from the block! That must make it hotter. And hotter objects emit more! Does the block ever get to see this amplified source?
How could my critics remove energy from the block back to the source and then forget about it?
I don’t get it. I really don’t understand your math outside of the ASSUMPTIONS you put in it.
I completely understand what you guys did, and it’s still smells funny. But I guess you can’t smell it, because winning an argument is more important. It all actually makes perfect sense within the framework of your idealogical mathematics, I’ll give you that.
“Why do my critics convert all of conduction to radiation on the cold side, but on the hot side they reject most radiation becoming conduction?”
Heat will move by any and all means available from warmer areas to cooler areas.
* On the hot side, heat can conduct toward the cool side and can radiate toward cool space, so both happen. Some conduction and some radiation.
* On the cool side, heat can radiate toward cooler space, but cannot conduct anywhere. So only radiation.
So the hot side can send photons right through the photons coming in. Cool.
Or the hot side can emit EM waves right through the EM waves coming in.
Brilliant!
If Planck thought that there was two-way photon travel in his cavity, his radiation formula would have an extra 2x factor, but there isn’t because Planck knew there was only one standing waving per frequency between all two opposing molecules of opposing walls.
Where is it written that Planck described his standing waves as being between two opposing molecules?
light , EM and sound waves can all flow in both directions at the same time. The fields superimpose. When you look in a mirror, does the light coming from you face block the reflection?
You should really think before you post, you are making yourself more ridiculous at each step.
Have you ever heard the expression: when in a hole stop digging?
Excellent example, Greg. When you look right at your eyes in a mirror, the light goes from some light source to your eyes, thence to the mirror and right back to your eyes.
And that obviously is what Zoe ridicules as “two way photon travel”, which despite her ridicule happens all the time.
w.
Willis,
We’re talking about normal radiation. Obviously photons can pass through angles.
What you failed to show is that radiation from your eyes comes back to your eyes [thus making it warmer due to the mirror.]
“heat can radiate toward cooler space”
Tim , what makes you limit that statement? Radiation happens equally in all directions, it does not poll what is happening on the other side of the universe before setting out.
This is a common misinterpretation of the second law of thermodynamics. In Willis’ example the sun will radiate more heat towards the object than the other way round, thus 2nd is obeyed. That is NET flow of energy, it does not forbid radiation to be emitted from the block back to the sun.
Greg,
“Radiation happens equally in all directions, it does not poll what is happening on the other side of the universe before setting out.”
Tell me the scientist that originated this idea and quote him.
Greg, I was trying to emphasize that heat = Q (the net flow of thermal energy between two regions) is always from hot to cold. Ie
* Heat always goes from hot to cold
* Radiation is one form of heat
* Therefore radiant heat is always from hot to cold.
I was trying to say the same thing you were saying. Radiation itself goes both ways; the net radiant heat is always from hot to cold.
“Why do my critics convert all of conduction to radiation on the cold side”
… because that is all there is happening on the cold side.
Why the offensive language. The fact that you can not understand the difference is your problem, it does not impinge on the integrity of others.
Pretending your own short comings are someone else’s fault is hypocrisy.
It does not “reject” it ( apart from the 5% reflected ) , it absorbs it, gets hotter and re-emits it. It is not “returned” to the sun, it is radiated over a full hemisphere. You could calculate what minute proportion of that is in the sun’s direction and how many milliwatts that will be but you can be sure it’s not something the sun is going to notice against its 63 MW/m2 output. So technically, yes, it will heat the sun but you’d need a whole lot of sig figs to write down the difference.
Because anyone with half a brain can see it’s insignificant.
Oops, projections. Hypocrisy.
Greg,
You could take an example from Tim Folkerts. He understood my point 100%.
In my original post that inspired Willis, my block was supposed to demonstrate geothermal heating the hot end. No radiation input.
Willis’ example was not supposed to be clone of what you did, it was in fact considerably harder to solve but it does show conservation of energy and constant fluxes through out the system.
So now you are dropping the whole discussion here and trying to divert back your original post on wordpress.
You do realise this is looking a lot like retreating to where you came from. Did you feel you were doing better in that discussion?
“You could take an example from Tim Folkerts. He understood my point 100%.”
No, I didn’t. Your understanding of the basic physics of this problem is wrong.
“So the hot side can send photons right through the photons coming in. Cool.”
Of course it can. Just like I can shine a flashlight at you and you can simultaneously shine a flashlight at me. Photons easily pass through each other.
“If Planck thought that there was two-way photon travel … there was only one standing waving ”
A standing wave IS two-way travel! A standing wave is two equal waves heading in opposite directions.
Thanks Tim, I was going to point out that she did not understand what a standing wave was but you have to limit the number of issues you raise at one time. It seems that a single question is already information overload for this self proclaimed successor to Einstein.
“A standing wave IS two-way travel! A standing wave is two equal waves heading in opposite directions.”
Great! You have 1361 W/m^2 of waves going from sun to block, right at the beginning. So what is this 1162 W/m^2 now going back?
Is that in addition or is it overlaid with what came in? And if it is overlaid then how is it a loss? 1361 covered it in the beginning – i.e. two-way 1361 as you say.
“i.e. two-way 1361 as you say.”
Sigh. I never said any such thing. YOU brought up standing waves in a different context (cavity radiation). I pointed out your misunderstanding in that context — that within a cavity, the standing wave is the superposition of 2 equal waves in opposite directions.
Nothing in the concrete cube scenario is radiation within a cavity. The 1360 W/m^2 of solar radiation is definitely not a 2-way standing wave.
Tim,
Planck had ONE standing wave, not 2 waves superimposed creating the illusion of one.
If what you said was true, Planck’s formula would have a 2x factor for this increased photon density you proposed.
Sigh, sophists will sophize.
“ … That energy returning from the hot side back to the source must make it hotter, no? Not only does the source have its own energy but its getting backenergy from the block! That must make it hotter. And hotter objects emit more! Does the block ever get to see this amplified source? …”
—
Incredible, you’re actually serious. The illuminated side of the block does NOT redirect its (absurdly characterized “rejected”) energy back towards its original “source”. You actually think the 0.95% of the 1,361 w/m2 light must go back to the emitter? You don’t even seem to realize that re-emission (and even scattering off a surface) can go in all available directions, into open space. At this point I’m amazed how misguided you are, and how you got such basic observational things so discombobulated. But your ongoing attempts to avoid all direct questions and requests for answers, and ignoring of all calls for a reasonable explanation, or citations. And you claim people respond with “ideological mathematics”, when you can’t even make output balance input!? However, you want people to take you ‘seriously’ and if they don’t you act as though they were wrong to reject your many false assertions?
I’m left wondering what your motive is because it sure isn’t to get the details of EM radiation and thermodynamics hammered out straight and true.
Now imagine a john Q public who doesnt have Willis’ skill.
They will happen on this discussion and have no clue who is correct; willis or Zoe?
( psst Willis is )
But imagine our John Q public. How will they judge? they can’t work the math themselves
How will they judge?
by vote?
by credentials?
by identity? willis is a nice guy, Zoe is a piece of work
or will they say ‘hey this isn’t settled?”
what would be a rational approach for someone who cannot check? who doesn’t get it?
The rational approach for people who DO GET IT is clear. watch. observe how they reason together.
what about Joe bag of donuts? how does he rationaly decide who is right
Wow, I hate to be the one who points out the *elephant in the room*, but everyone knows there are tiny bubbles of CO2 gas trapped inside all Unobtainium, and in the concrete itself. CO2 gas, as every climate scientist knows, changes the amount of heat trapped inside the concrete by a catastrophic order of magnitude. One would have to model the concrete and level of CO2 in order to determine the amount of heat as no physical process exists to explain it.
Sorry for those of you trying to take this seriously, but there you go!
Ah, that’s it !
That is how Zoe manages to get 557W or for 2.5W in, it’s CO2 amplification.
Now my critics are suggesting backradiation is OK, but backconduction is not. How silly.
“silly ” is not a scientific argument, what is you point?
Again, it’s not “backradiation” it’s radiation. All surfaces do that.
I love how Willis turned my conduction to radiation problem which acted like a euphamism of geothermal to space into a point-source radiation to conduction to radiation problem in order to show me wrong. Assuming your premises true: great job Willis! but there is no backradiation into Earth. The surface will get what it gets from geothermal, and there won’t be magical backradiation from Th in order to deprive Tc.
Or are you now going to claim backconduction from Th?
Well why not backconduction from Tc in this example?
“acted like a euphamism of geothermal to space”
That word euphamism, I don’t think it means what you think it means.
” … I love how Willis turned my conduction to radiation problem which acted like a euphamism of geothermal to space into a point-source radiation to conduction to radiation problem in order to show me wrong. …”
—
Actually your assertions of such a geothermal flux were already debunked due to being inconsistent with observations and implications for the near surface terrestrial geotherm profile, plus ocean basin thermal profiles. If you were correct about such a high geothermal flux deep mining would not be possible as we couldn’t provide viable cooling to survive a kilometer underground. And long-chain hydrocarbons would have already been thermally eliminated before humans came along. So you wouldn’t be wearing plastic knickers. And if earth’s interior were so hot how do earthquakes in a rigid solid mantle still occur down to 680 km? We know its rigid rock that’s fracturing there because measured seismic wave velocities show it is. If it was liquid there would be no quakes at all, no faults, no fracturing, no mineral bonding within liquid for storing convective cell motions as elastic energy with. No mechanism for creating the observed epic acoustic shockwaves so far down that we see occur every day.
WXCycles,
“If you were correct about such a high geothermal flux deep mining would not be possible”
You obviously lack reading comprehension.
You lack the ability to make a coherent point when challenging a factual statement made by someone else.
WX made a reasoned argument with facts and numbers and the best you can do is a vague “lack reading comprehension”. Do really you think he or anyone else will go : gee you are right, why didn’t I see how flawed his position was ?
Was that comment even meant to say he was wrong? Once again you fail to make any meaningful comment.
Greg,
“WX made a reasoned argument with facts and numbers.”
You also lack reading comprehension.
The geothermal heat flux (CHF) is 92 mW/m^2.
The geothermal emission (CSR) OUT of the ground is like 335 W/m^2.
All references to mining should use CHF, and not CSR.
WXCycles purposefully confused the two to score some cheap points.
“If you were correct about such a high geothermal flux”
OUT OF THE SURFACE, NOT IN THE GROUND.
“… OUT OF THE SURFACE, NOT IN THE GROUND.”
—
Is the wall of a mine shaft not a “surface” emitting into the same gas mixture as above? Please explain why we still need active cooling with giant fans pushing air down vents to survive the thermal emission from the walls? Specifically, why do some IR photons emit from the “surface” of a mine’s walls, but not the whole 335 W/m^2 enchilada?
Please explain in plain English Z so we can all understand your reasoning.
The sun emits 63MW/m^2, and the conductive heat flux in the photosphere is TINY.
Why don’t you check your BS with observations?
What EXACTLY are you claiming is “BS” ?
What is the relevance of conductive heat flux in the photosphere? TINY compared to what ?
You are just spouting gibberish again. Try to make a coherent point for once and explain what you mean.
According to religion on this forum, CSR = CHF
The photosphere is what emits 63MW/m^2 (CSR), but the CHF in the photosphere is TINY compared to this.
I understand that the religious fanatics on this forum will never understand or acknowledge this. How could they? Their ideological mathematics is inoculated from observations.
Their only plan is to not understand what I’m saying. That’s your plan, isn’t it?
Oh no, what if Zoe is right?
Nah, Zoe is so stupid, she doesn’t understand how ideological math is real science.
At equilibrium, the flux is 1360W in, through, and out.
So working backwards, and using engineering toolbox calculator (it’s not cheating, it’s working smart):
https://www.engineeringtoolbox.com/radiation-heat-transfer-d_431.html
(I had to iterate the inputs to get the required output)
Tc = 125.6 C
Again, using the calculator for conductivity:
https://www.engineeringtoolbox.com/conductive-heat-transfer-d_428.html
Th = 1825 C
According to Stefan – Boltzmann you would be emitting 1043586.8538327338 Watts from the front face. I find that hard to believe.
I have learned some things today:
If I strap my wifi dongle to the wireless router I won’t get a signal because the distance to the aerial is shorter than the wavelength.
If I shine a torch into space I will get no reading on the ammeter and voltmeter on the battery. There will be a reading if I place my hand in front of the torch.
Zoe is very entertaining and passionate in her beliefs.
I don’t want Zoe on my side. If she were to agree with me I would have doubts in my own conclusions.
If she were to agree with me, I would doubt my own sanity !
She is displaying exactly the same kind of refusal to deal with logical scientific arguments as you get from flat-earthers. It is more important to them to cling on to their little brain-fart “discoveries” which make them a special person than it is to work from a common basis of understanding to get to the truth.
This is a sociological phenomenon, not a science question.
This whole exercise illustrates with poignant clarity why discussing science with a climate alarmist is like peeing into the wind.
If someone who considers themselves skeptical of AGW is this far disconnected from logical argument, what kind of hope do you have with someone whose entire worldview, political self-identity and peer-group social standing is grounded on screaming “DENIERZ” instead of seeking the truth.
We really have reached the end of the age of enlightenment. We will probably descend rather rapidly into a new dark age now. The next glaciation will take care of what remains.
You have to decide at what point to stop beating your head against a brick wall.
Alex,
There’s more than one wavelength in thermal interaction.
If your wifi was based on 2m signal, it would work at 2m, 4m, 6m, etc., but not 1m,3m,5m, etc. It would be terrible technology.
“If I shine a torch into space I will get no reading on the ammeter and voltmeter on the battery. ”
When WMAP satellite at L2 shined its 4K reference load at the stars, only 2.275K came out. And we called this Microwave Background Radiation.
According to theology on this forum, all 4K should have come out.
Great thought exercise by Willis.
Now for extra credit: What if we were to apply a gravitational field/attraction to the hot side of the cube. Would the cold side warm to a higher temperature? (gravitational field considered to be produced by non-emitting invisible force).
I approached it as an energy balance equation
Energy in = 1360 x 0.95 x area = 1292 W
At equilibrium, Energy In = Energy lost from the front face + Energy lost from the rear face.
So 1292 = δ x (Tfront x 0.95)^4 + δ x (Trear x 0.95)^4
Energy conducted from the front to the rear is a function of the temperature of the two faces, (and a unit distance). Energy conducted = 0.8 x (Tfront – Trear)
Simultaneous equations will get the answer, or you can load the two equations into excel and play around with the two temperatures to get an answer close to Tfront = 379K and Trear = 217K
Given your equations, yes, you could load the equations into Excel and play around . . . but you would never get the correct answers for the front and rear face temperatures . . . and your’s are not correct.
You see, in the S-B equation for radiation power exchange, the emissivity factor (0.95) is not brought together with the absolute temperature term (T) into a term that is then raised to the fourth power: as your expressed it, (Tfront x 0.95)^4 and (Trear x 0.95)^4.
It is only the absolute temperatures that are raised to the fourth power.
The cold side will emit Th-Tc 0.8 W/m2 (as L is 1m) = qc.
Th ^4 = (1360 W/m2 -qc)/0.95σ
Tc ^4 = qc/0.95σ
qc = ((1360 W/m2 -qc)/0.95σ -qc/0.95σ) 0.8 W/m2
qc = (1360 W/m2 -2qc) 0.8W/m2/0.95σ
I’ll have to call that coefficient C
1/C = 1360W/(m2.qc) -2
Soccer is on but the arithmetic from here shouldn’t be difficult like typing this on a phone.
I
The cold side will emit (Th-Tc) 0.8 W/m2 (as L is 1m) = qc.
Should have figured it out on a piece of paper first.
exchange heat with void?
i just don’t understand…
why the heck add unaobtainium?
unaobtainium , is that a material which you can only get one bit of?
“Unobtanium” is the material used to fabricate replacement aircraft parts, the originals of which were fabricated from “Breakseasium”
I hope that clears things up.
I can’t believe how many ****ing wrong answers there are here.
(unit system all mks)
Th=389K
Tc=223K
Yeah, I ran to 3 sigfigs since I don’t think you’re interested in just one
Th= hot side temperature (kelvin)
Tc = cold side temp
k = thermal conductivity = .8
Conductance through the cube
1) q=k*(Th-Tc)/l
Power radiated from cold face
2) Pc = Ec*Bk*Tc^4*A
A = area of a face = 1m
Bk=Stefan-Boltzmann constant = 5.67 x 10 -8
Ec = emissivity of concrete face = .95
Steady state, power radiated from cold side equals q through cube
3) q=Pc
Power radiated from col and hot must equal power incident on hot side
4) Pi=Pc+Ph
Pi = power incident = 1360
Need to substitute Tc for Th:
q = Pc
k(Th-Tc) = Ec*Bk*Tc^4
Th=Tc + (Ec*Bk/k)*Tc^4
Subbing in for Th:
Pi = Ec*Bk*Tc^4 + (Tc + (Ec*Bk/k)*Tc^4)^4
If you want to solver for Tc, be my guest. I used a matlab program to settle on the values and verify eq 4)
Matlab
—————————–
Pi=1360; %incident power, W/m2
k=.8; %conductance W/mK
Ec=.95; %emissivity of the concrete
Cc=.75; %specific heat of concrete kJ/kG*K
Bk=5.67e-8; %boltzmann constant
Th=0; %temperature of the hot side
Tc=0; %temperature of the cold side
minErr=1e-4;
cmpVal=Pi/(Ec*Bk);
estimateArr=zeros(1,1000);
tst=0;
while (tst<=1000)
tst=tst+1;
tstVal=(Tc^4)+(Tc+(1/k)*Ec*Bk*Tc^4)^4;
diffVal=nthroot(cmpVal,4)-nthroot(tstVal,4);
Tc=Tc+.1*diffVal;
estimateArr(tst)=diffVal;
if (abs(diffVal)<minErr)
break
end
end
estimateArr(tst+1:end)=[];
plot(estimateArr);
Th=Tc+(1/k)*Ec*Bk*Tc^4;
Ec*Bk*(Th^4 + Tc^4)
title(['Th= ' num2str(Th) ', Tc= ' num2str(Tc)]);
PseudoNhymm says:
“Power radiated from col and hot must equal power incident on hot side
4) Pi=Pc+Ph
Pi = power incident = 1360”
No. Power radiated from cold and hot must equal power ABSORBED on hot side.
Pa = Ec Pi = 1292
Since you used the full Pi, your numbers are both a little high.
I can’t believe that after saying that you added a wrong ****ing answer. LOL.
4) Pi=Pc+Ph
Pi = power incident = 1360 *0.95
If the cube is perpendicular to the radiation from the Sun, then there are 5 cold faces of 1meter squared, each radiating. So, the power radiated from the |FIVE cold faces is 5*Pc.
In the steady state, Pc=1348/5=5.67 x 10 -8*0.95*Tc^^4
Thus Tc = 262 Kelvin
The exercise stipulates that four of the faces are covered with a perfect insulator and are not radiating, and the insulation absorbs no energy from the cube either.
Correct, which is why this is mathematically equivalent to an infinite sheet problem. There is heat flux only through the top and bottom of the sheet. There is none out the edges, which are at infinity. It’s this sort of trick that allows one to convert sheet, cylinder and sphere 3-d diffusion problems into 1-d problems. In this case, we have steady state and the time derivative is zero. The solution for T to the 1-d differential equation is a linear function of position from a surface of the sheet. So the problem boils down to figuring out the values of T at the boundaries, subject to flux (proportional to dT/dx) values at the surfaces, which have to account for incoming energy and blackbody radiation out. The cool thing is that heat capacity is not important here. Different heat capacities merely affect the time it takes to reach steady state, not the final temperature profile.
I remember solving this sort of problem decades ago to show that continuous lasers could be used to uniformly heat small silicate mineral grains. Good time, good times.
Willis: This is the epitome of a “well-posed problem.” If I may, I’d like to add one more problem to the mix, one that’s equally well-posed, yet whose solution may elude everyone – or at least astonish everyone.
First, let’s establish sign conventions:
If energy enters the system, its sign is positive.
If energy leaves the system, its sign is negative.
If work is done on the system, its sign is positive.
If work is done by the system, its sign is negative.
Now, given these sign conventions, what is the time rate of change of entropy of the system you have described? There are three choices: zero, some number greater than zero, or some number less than zero. Other than zero, a numerical answer is not required…
If you are including the energy source ( sun ) that is losing energy which is being dispersed in all directions, positive. The bit of energy in beam hitting the concrete is then also dispersed in all directions at each end. Both those processes are an increase in entropy.
Thermal energy at the hot end is flowing to the cold end. Since this is descibed as steady state the temperatures are not coming closer together but you need to consider the loss of energy at the cold end flowing out into emtpy space in all directions. Entropy ++ .
You’ve taken a well-posed problem and turned it into a complete mess. Who said I was “including the energy source (sun)”? Just look at the system Willis has defined.
Willis, scrolling through the comments this morning, I see why you did this. Good for you.
For others reading this now or in the future, please consider that Willis gets this right, because the fundamentals from which he constructs his problem and answer are plain for all to learn and verify for themselves.
When I first saw the head post of the problem posed, I thought, “I got this.” But it took me a while to knock off the rust and get the problem framed into the necessary equations. So I learned again that humility and persistence pay off. And I learned again there may be alternate paths to the same correct answer.
David,
I imagine many of us can relate to your comment.
Now Zoe is hilarious
https://phzoe.wordpress.com/2020/02/12/average-moon-temperature/
I do give her credit for sharing her code. it lets folks find her error immediately
The error is not in the code. It is in the false idea that you can average temperatures !
The false idea is that you should convert temperatures to radiation, average radiation, and then convert back to temperatures. That is so insane.
Let’s say you have 2 objects with equal mass and heat capacity. One at 0C and the other 50C.
You bring them together. What will their equilbrium temperature be?
Will it be 25C?
or 27.94C? (The Willis Method)
If the two objects have the same heat capacity, it’s legit since temp is then ( and only then ) proportional to the energy content. But that’s a big if. That is exactly the reason that you should average energy content not temperature. ( see intensive vs extensive properties ) You can impose special conditions under which temp is a valid proxy for heat content. In that case it works.
If you are trying to calculate the instantaneous SB thermal emissions you want to be using the “Willis method”.
As you can see in the graph in this article land temps change about twice as quick as SST. That is because land ( wet rock ) has about half the specific heat capacity as sea water.
https://judithcurry.com/2016/02/10/are-land-sea-temperature-averages-meaningful/
Averaging the two ( where land represents only about 30% of the earth’s surface ) biases the result high. That in essence is why it is done.
In that article I suggested a weighting of land temps by SHC and area. The traditional 70/30 weighting becomes 85/15.
Of course, all that assumes that you have not spent the last 40 years rigging the various datasets to support you agenda in the first place !
As you see if you take the time to read it, he was not trying to average the thermal energy of the surface by averages T^4, he was trying to get the emitted thermal energy to do energy budget calculations. That seems perfectly sensible to me.
He is also very open about the limitations and the context of what he is doing, in fact he does both with the aim of comparing. So saying “Willis’ method” as though he only sees one way of doing it and applies to all is disingenuous.
“he was not trying to average the thermal energy of the surface by averages T^4”
In your quote that’s exactly what he says he did.
read the quote again.
That why I never average temperatures. Only Tony heller does that
HadCRUT, GISS LOTI, BEST all average temperatures. The whole climate discussion is based around average temperatures. Worse they are land + sea averages. Different media.
400 comments in barely 24 hours. Nice! 🙂
I think half of them were mine 😀
Having trouble getting through, though.
The solution is straightforward. Two equations and two unknown. 1) T1^4 + T2^4 = 1360/sigma, 2) T1-T2 = sigma • T2^4/k, T1 is the front side temp and T2 is the back side temp
Unfortunately, the equations are non linear so a graphical solution is the simplest way. We know that T1 > T2 for equilibrium heat flow, we know that T1max = 393.5 K thermal conductivity = 0, T2 < 330 K (the point that radiation from both sides is equal to the input flux. In equilibrium the radiated heat from the back = the heat flow through the concrete.
We need only plot radiation from back side sigma T2^4 and heat flow Q = k (T1-T2) delta T (T1-T2) over the range of T1 = 330 K and 393.5 K and look where the curves cross T1 = 383.727 K and T2 = 218.99 K.
Albedo 1 above. The 0.95 albedo only is a 1.3% correction and only shows up in eq. 2. T1= 383.28 K, T2 = 221.32 K
I posted that yesterday and again about 4 comments above. Essentially you use the same method.
Zooming in a little closer to gain accuracy I get :
221.42 and 383.28 K
Why do you think your cold end is significantly different. ?
Greg,
I’m actually impressed with your gnuplot solution. I learned something new.
Thanks for the positive comment. I’m glad you found it useful.
Which scientist first made the claim that hot and cold will send photons to each other? That there will be two-way heat flow, but only one NET heat flow?
Who started it?
Well, the idea of photons was developed by Einstein. So he would be the first to make such claims.
But sure, feel free to correct Einstein.
So obviously finding such a claim by Einstein should be easy.
I don’t see how calling waves photons and returning back to the corpuscular theory of light was progress, when dozens of scientists explained photoelectric effect using waves.
I’ve mentioned this several time but it seems convenient for you to ignore it. The current concept is NOT a return to Newton’s corpuscular light, it is wave-particle duality. A recognition that light is neither billiard ball particles nor purely wavelike but displays both kinds of properties. It depends on the situation as to which model is most useful in describing observations.
Remember, all the “laws of physics” are not discoveries, they are human inventions. Attempts to capture the key aspects of what happens and model them in a way our brain can deal with in an attempt to have enough understanding to predict future behaviour.
Neither does a wave have to a continuous stream or two way standing wave. Consider the concentric rings spreading out when you throw a stone into a pond. Now imagine the cross-section of the wave, there’s a main peak with smaller ripples ahead and behind which is propagating forwards. This is similar to the idea of a photon as a wave packet travelling through space.
Einstein in his 1917 paper has this to say about the heating of a gas by photons:
http://inspirehep.net/record/858448/files/eng.pdf
During absorption and emission of radiation there is also present a transfer of momentum to the molecules. This means that just the interaction of radiation and molecules leads to a velocity distribution of the latter. This must surely be the same as the velocity distribution which molecules acquire as the result of their mutual interaction by collisions, that is, it must coincide with the Maxwell distribution. We must require that the mean kinetic energy which a molecule per degree of freedom acquires in a Plank radiation field of temperature T be
kT / 2
this must be valid regardless of the nature of the molecules and independent of frequencies which the molecules absorb and emit.
Einstein understood like no other before or since the unity of mass, light and time. The equation e=mc^2 encapsulates this. Here he also sees that heating of matter by photons isn’t that different from heating of matter by matter. And the whole IR absorption blanket idea is false.
From your various comments, I think the bottom line question you are seeking is: what is the justification for a body emitting into a void , without another body, another “receiving ” molecule or a standing wave between two bodies?
Off the top of my head , I can’t tell you who is responsible for that crazy idea. 😉
You seem to refuse the concept of a photon entirely and are not happy with the concept of wave particle duality.
Just as an aside, as an undergrad, I recall being given the exercise to calculate the diffraction of a 20 tonne truck, driving at 60mph between two narrowly spaced buildings, as one would do for a photon going through a narrow slit.
You can do it, but the answer is some insanely small deflection that would never be measurable. The point being that you can even model a truck as wave.
https://en.wikipedia.org/wiki/Photon#Historical_development
Waving a rope can be used to create a whipping force.
If EM waves travel through aether they too can have a momentum just like the rope.
Science works by flasification, not advocacy of one group’s POV.
Why don’t you mention those that debunked the corpuscular view of light and unified everything under wave theory, rather than those who failed to do so and left an unnecessary duality.
You should watch some of the PBS space-time YouTube videos by Matt O’Dowd. This one explains that matter and radiation aren’t that different from each other (e.g. the photon box thought experiment at 1 min 29 sec:
https://youtu.be/gSKzgpt4HBU
So much effort to solve a “thought exercise” that bears no relation to the real physical world. The gray surface model stipulated by Willis reduces the exercise to a simple mathematical problem that is irrelevant to the real world heat transfer problem.The student should rather read the engineering literature concerning topics such as spacecraft thermal control ,solar collectors etc.,to understand the critical effect of non gray behavior on heat transfer involving solar radiation.
Until you are able to deal with such a simple idealised exercise you are unlikely to get too far with a more complex , realistic analysis.
AM, you missed the important revelations of this “thought exercise” completely.
They are:
(1) the percentage of presumably-educated individuals that make logic mistakes in considering the solutions to the postulated problem, which in reality is not all that hard to comprehend and solve.
(2) the obstinate, condescending attitude some people take when they are shown to be poorly informed or flat out wrong, and
(3) how easy it is for humans to make mistakes in performing what are really simple algebraic calculations.
Now with this as a basis, how do you think humans will fare in the nearly intractable issue of “fighting climate change” . . . however you want to interpret that phrase. Feel comfortable spending $50-100 TRILLION trying to do so, given the results seen here of Willis’ “thought exercise”?
Yes I have, it’s my job. Remember this is an exercise intended to provoke thought and interest. The most critical aspect oversimplified is the absorptivity and emissivity are different at different wavelengths, henc the almost ubiquitous use of white paint.
Exactly. A waste of time with no insight how the world works. I am still confused be Willis statemt that the Tao buoy readings show back radiation. I’m not saying they don’t, bit it’s far from clear to me. For the life of me I can’t understand the focus on radiation when convective forces dominate in the troposphere. It’s a little like asking the drunk who lost his keys why he’s lóoking for them under the street light.
“when convective forces dominate in the troposphere.”
Yes. But radiation dominates from the top of the troposphere to space. Neither one by itself gives the whole picture. Both are needed to understand climate and temperatures in the atmosphere.
Nelson February 29, 2020 at 3:24 pm
What’s not clear? As I said, downwelling longwave radiation from the atmosphere has been measured all over the planet for decades, both by scientists and by automatic recording stations like the TAO buoys and the SURFRAD stations. That’s just a fact. Google it if you doubt me. Heck, go here, you can plot the SURFRAD downwelling infrared (longwave) for yourself from any of the SURFRAD stations.
As a global 24/7 average there’s about 160 W/m2 of shortwave solar energy absorbed by the surface, and about 340 W/m2 of downwelling longwave absorbed by the surface. So longwave radiation is a huge part of the surface energy budget, more than twice that of solar energy, and as such it deservedly gets lots of focus.
Regards,
w.
The solution tom 2 decimal places is:
T1= 383.28 K
T2= 221.43 K
These assume sigma is 5.67E-8
Part of the exercise was to “show your work”. So, apart from copying the consensus result from several earlier contributors an asserting it like you are a sage to whom this is abundantly obvious, what is your method for arriving at that result?
In the postulated problem:
— the concrete block dimensions are given to one significant figure,
— the block’s emissivity is given to two significant figures,
— the block’s thermal conductivity is given to one significant figure.
Given the great importance of the block’s frontal area and emissivity in deriving the correct face temperatures, it is improper to give temperature solutions to five significant figures.
“It is the mark of an educated mind to rest satisfied with the degree of precision which the nature of the subject admits and not to seek exactness where only an approximation is possible.” — attributed to Aristotle
This Cambridge University research reported at Science Daily, analysed the atmosphere on a planet 125 light years away with a mass twice that of earth. It’s in the habitable zone with the possibility of water at the surface.
https://www.sciencedaily.com/releases/2020/02/200226212008.htm
Here’s the approach they used to analyse the atmosphere:
Given the large size of K2-18b, it has been suggested that it would be more like a smaller version of Neptune than a larger version of Earth. A ‘mini-Neptune’ is expected to have a significant hydrogen ‘envelope’ surrounding a layer of high-pressure water, with an inner core of rock and iron. If the hydrogen envelope is too thick, the temperature and pressure at the surface of the water layer beneath would be far too great to support life.
This conveys the suspicious impression that knowing the mass density and (gravitational) pressure only, together with distance to the star, tells you the temperature.
Oddly enough, I missed any mention of CO2. Shouldn’t atmospheres freeze to absolute zero without at least one molecule of CO2 present?
This rings a bell – didn’t two scientists propose a similar idea recently – that mass, pressure and distance to the sun is sufficient to know ground level atmospheric temperature? With composition irrelevant? Nikolov and Zeller I believe – it was that Volokin and Rellez?
N&Z got their causality backwards.
Turn off geothermal and solar, and the entire atmosphere solidifies and falls to the ground. This solid “gas” mass still exerts a pressure, but there is no atmosphere. Obviously pressure doesn’t make it hotter.
There is only one sound theory (mine):
https://phzoe.wordpress.com/2020/02/13/measuring-geothermal-a-revolutionary-hypothesis/
https://phzoe.wordpress.com/2020/02/25/deducing-geothermal/
“(mine)”
Now that was funny!
After all of this you finally said something amusing.
I have always believed that you can get a measure of a person by how often they something intelligent, interesting, or funny.
One word out of a few tens of thousands does not cut it.
Still, at least for a moment, you said something that was hysterically funny.
I actually did LOL.
Most satellites in Earth orbit run at roughly 250-350K. The big problem on telescopes is keeping the sensors cool and the structure at a constant temperature so there is no thermally induced distortion of the optics.
And did I mention electronics?
Another interesting thought experiment is the “photon box” that Matt O’Dowd discusses starting about 1 min 20 seconds into this:
https://youtu.be/gSKzgpt4HBU
It turns out that matter and radiation are not fundamentally different from each other.
Check out photonic matter. We’ve been able to convert invariant mass matter to energy for quite a long time, but to convert energy to invariant mass matter is a relatively new discovery. I consider it the first tiny step toward a Star Trek-type replicator… a long ways off still, to be sure, but we’ve at least taken that first step.
The photon, being massless and circularly polarized when considered singularly, doesn’t carry its energy in its linear momentum, it carries it in its angular momentum.
It is the interaction of the electronic and magnetic fields, oscillating in quadrature, geometrically transformed into a spiral (because a sinusoid is a circular function and a circular function spread axially over space-time is a spiral)
which is where the energy of a photon is carried.
http://staff.washington.edu/bradleyb/spiralsynth/fig3.1.gif
This is why, when traveling through transparent mediums of differing refractive indexes, the photon energy does not change, while the apparent photon speed does.
E^2 = p^2 c^2 + m^2 c^4
pc is the magnitude of the momentum vector. Since c is fixed in vacuum, p must change for the photon’s energy to change.
p=ħk
where k is the wave vector (where the wave number k = |k| = 2π/λ), ω = 2πν is the angular frequency, and ħ = h/2π is the reduced Planck constant.
The energy and momentum of a photon depend only on its frequency (ν) or inversely, its wavelength (λ):
E= ħν = hc/λ
This is why Planck’s constant has units of angular momentum (J-s), and the reduced Planck constant represents the quantum of angular momentum.
Apparently, converting photon angular momentum into rest mass is the key.
If anyone is still reading, a better problem is what is the relative mean temperatures of a sphere that is illuminated from a point source and such extremely high thermal conductivity that the whole surface has a constant temperature, compared with a sphere of insulating material so that each section of the surface behaves like an isolated black body.
I don’t have it on me but I did a back of envelope (still required a few sheets of paper) and got about a factor of 2.5. The main simplification was to have linear relation of T with angle of incidence on the illuminated side from 0 to Tmax in the latter.
1360 W * 0.95 = 1292 W
783.28 W + 508.72 W = 1292 W
T_hot 70 C (783.28 W)
T_cold 35 C (508.72 W)
70 C = 343.2 K
343.2 K * 0.8 W/K = 274.56 W
783.28 W – 274,56 W = 508.72 W
(Relationship between degrees Celsius and Blackbody temperature according to Wolfram Alpha.)
A simple problem:
Place a frying pan on a gas stove. Turn it on. We know the bottom will get 400F. After a long while, what will the top of the frying pan emit?
Assume emissivity = 1.
Next question: what will the conductive heat flux be?
Zoe Phin February 29, 2020 at 4:41 pm
said
How on earth do you know that? You really really do not know that. The temperature that the pan reaches depends on the size of the pan and the power(wattage) of the flame.
You are completely out of your depth.
This is not a difficult problem.
https://youtu.be/Var3o_eko9U
Anyone doubt that the CHF is nearly zero?
Anyone still doubt that CSR != CHF?
Come on sophists, do your sophistry …
Everyone who thinks this problem is unrealistic or that the two sides are the same temp: Go outside and find a large flat rock. Shaped like a lens would be best, but not required.
Hold the rock up to the Sun.
Wait.
Feel the side in the Sun after a nice long while.
Feel the other side.
Are they the same?
Why not?
Now the Sun goes down.
What happens?
How does the hot side feel after a few hours, compared to when it was in the Sun for several hours straight?
How about the other side? How does it feel, compared to when the Sun was shining?
How long does it take the hot side to cool down?
What happens to the energy that had made it warm?
On a cold clear night with low humidity, place a thermometer under your car sitting in the driveway.
Place another above the car, but not lying on it, maybe on a wooden post right next to the car. Make sure it is not pointing at the sky…put a piece of cardboard overhead of it. As an experiment, place another one face up on top of the cardboard. Cheap alcohol in glass thermometers can be had for a small price at any home store of big box market or on Amazon.
In the middle of the night, place your hand on the metal of the roof of the car…or the top of the hood or the top of the trunk if your car has a fabric roof.
Now place your hand on the metal of the bottom of the car.
The thermometers will tell you that the air temp is about the same under the car as above it, although very close to a concrete surface that was in the Sun all day, it will stay quite a bit warmer.
The thermometer on top of the cardboard facing the sky will show a much lower reading that the one facing sideways under the cardboard.
The car roof will be very cold to the touch, under the car far warmer.
Try it with a car on grass another time.
Another way to understand radiation and heat conduction is to spend a lot of time outside all night when the temp is cold enough and the sky clear enough, winds light enough, and air dry enough, for frost to form.
Frost will form on car roofs first, at 38°F…far above the melting point of ice.
Next will be grassy surfaces and blades of grass themselves.
If the day time temp is warm, you will never get frost on concrete.
You will never get frost under a tree with leaves on it.
Here in Florida, there are a lot of nights in the central part of the state when it is warm in the day, and chilly or very cold at night. In agricultural areas, it can be nearly a hundred nights a year or more, between October and April.
How can frost form when the air temp is above the melting point of ice?
How can frost form on grass when the ground two inches under the surface is tens of degrees higher than freezing?
Why does concrete protect plants from frost, while a few feet away on grass, the same type of plant will be damaged from ice crystals?
How does spraying water on plants protect them on cold nights, when if a person gets sprayed with water on the same night in the same place and the same time, you will be suddenly freezing cold…far colder than if you do not get wet?
Materials all have their own capacity for storing and transmitting thermal energy, both from the surfaces and through the material.
Dirt has one, stone and concrete have their own, sheet metal, especially thin sheet metal, has another.
Why is it as much as ten degrees cooler under a live oak tree during the day, but that night when it is cold, under the same tree is five or ten degrees warmer than a foot away from the tree canopy?
The sky is very cold when the air is dry at all levels.
Dry air has a far lower heat content than air of the same temp that is moist.
Radiation from a surface is far faster at dissipating energy than the rate of heat conduction through many materials, such as stone, dirt, or concrete.
But heat conducts very rapidly through metals, and so a layer of sheet metal can get very cold if exposed to a cold sky.
Tree leaves are the same as the air temp at night, or maybe ever a little colder, but still far warmer than the sky, so they are radiating down to the air and ground under the tree, preventing frost and keeping it warmer than where the ground and air is exposed to cold sky.
Now for a surprise, and this I have verified by many nights outside protecting a plant nursery full or tropical plants from cold weather:
On a night with a cold clear sky, dry air, and temperature falling rapidly, it happens occasionally that a thin layer of cirrus streaks overhead from the southwest.
What happens then is immediate and dramatic.
Within minutes the air temperature at eyeball height will not just stop dropping, it will jump up, by several degrees.
Nothing else has changed…the dew point is the same, still no wind, no mixing of air layers…
Just a thin layer of ice crystals many miles up in the air.
Raises.
The.
Temperature.
Of.
The.
Air.
Near.
The.
Ground.
And.
Keeps.
Plants.
Alive.
I do not care who does or does not believe me, and I have seen this not once or a few times but dozens and dozens of times.
Entire orange and strawberry crops have been saved from killing frost or hard freeze by a wisp of ice crystal clouds many miles up where the temperature is far far below freezing.
Anyone who knows me knows I am no warmista, and am not a member of any sect withing the skeptical community.
I made these observations over many years, decades in fact, while I was building and running a tropical plant nursery in Central Florida, and for the first five or more years of such experience, there was no such thing as global warming alarmism…it was the early and mid eighties.
And at the beginning of that time I was in college, studying physical geography, chemistry, geology, math, physics, astronomy, biology, meteorology, climatology, botany, and every other topic in science offered by Florida Universities.
I have chronic insomnia…I only sleep a few hours a night.
I used to sit outside all night back in those days, sky watching, observing the weather and the wildlife (our farm is in the middle of nowhere if ever such a place exists)…and I still do, although not as much as back when there was no internet, no weather channel, no social media…nothing much to do but read and make observations.
I have no idea why anyone thinks photons will not leave a material in the direction of a warmer object, let alone why anyone thinks they will not leave a surface of any object unless they can see some matter for them to impinge upon.
Do stars radiate in all directions all the time or not?
Do stars in orbit around each other radiate differently than if they were in isolation?
Does a slightly cooler star in orbit around a slightly warmer star, close enough that the warmer star occupies a large part of the “sky” of the cooler star…stop radiating on that part of that side?
Our Sun sends out photons in all directions, even though it is a mathematical certainty at least some of those photons will never strike anything but will keep going forever.
Anyone who proves different has a big fat Nobel Prize and lifelong fame and an immortalized name waiting for them
Interestingly and coincidently, tonight is a cold and clear and dry air night here in Florida, and there may be some cirrus clouds coming in from the Pacific ocean across Central America, if not tonight then perhaps tomorrow night.
Dew point where I live is 30°F at the moment.
Forecast low is 41° although it may be a little colder and maybe we will get frost.
I am gonna see if the proper conditions exist to demonstrate when I was just talking about.
I have some old smartphones and a tripod and one of those phones will take time lapse video in HD.
I think one problem we have these days, and I know it is only one of many, is that there are a lot of people who have never made a single scientific observation in their life, never done a lab experiment, never spent whole days and nights outside in remote locations, never sat alone and just observed things for any length of time at all.
The little girl from Sweden (or wherever she s from…it hardly matter) just travelled around the world and took photos of herself in hundreds of places and posted them on social media.
Every one of them was some scenic locale of big city, and in most it was remarkable for the scenic splendor and the places.
But after months of such travel…she told us all again about how the planet is dying and in a crisis.
She has never showed one place with any crisis, or any place that is “dying”.
We can learn a lot from books, but not everything.
We can learn a lot from school, but the most important thing to learn is HOW TO THINK CRITICALLY AND FOR OURSELVES.
When what one thinks or believes does not match up with what can be seen, that person needs to change their mind, or they will just be wrong for the rest of their life.
Greetings Willis,
I did this last night on a napkin and got 383 Kelvin and 221 Kelvin.
But you asked us to show our work, so this had to wait until this morning. So here it is, including the units analysis. Thot = Temperature hot (front), and Tcold = Temperature cold (rear)
At equilibrium, energy in = energy out. So, Insolation Front = Radiation Front + Radiation Rear
At equilibrium, Heat Flow through the block = Radiation Rear
Professor Lehman taught us in Thermodynamics that if you can cross out all of the matching units on both sides of the equation and you have none left over, then you will not get the wrong answer. So, crossing out all of the units, striking the terms that appear on both sides of the equations, we can reduce the above two equations to just…
Two equations with the same two unknowns, Thot and Tcold.
So, we solve the second equation for the hot temperature (Thot) …
Then insert the Thot result back into the first equation to find the cold temperature (Tcold), and we have …
I cannot factor 4th power terms to even attempt to isolate Tcold. But I can guess, repeatedly, starting with say 200 Kelvin, and work my way up to find an answer that results in 1360 watts.
We use the Define Names part of the Formulas tab in Excel to name the cells that contain our constants and our results so that we can use them in our equations …
sigma 5.670374419E-08
epsilon 0.95
k 0.8
Answer 1360.0000000000
Tc 221.4224502148
Th 383.2796281619
The formula for the Answer cell (showing 1360.0000…) is “=sigma*power((Tc+(sigma*epsilon*power(Tc,4))/k ),4)+power(Tc,4))”
The formula for the Th cell is “=Tc+(sigma*epsilon*power(Tc,4))/k”
The Tc cell contains plugged values and is changed repeatedly until 1360 pops out as the answer.
Reporting the results with the same unreasonable precision that we see in orthodox climate science as practiced by the high priests of the Church of Anthropomorphic Global Warming, we have …
Temperature of the front of the block (hot): 383.2796281619 Kelvin
Temperature of the rear of the block (cold): 221.4224502148 Kelvin
I actually solved a similar real-world problem from INSIDE the block some years back.
I had a Digital Equipment Corporation VAX Cluster that we used to monitor the temperatures of IV bags during sterilization in a set of autoclaves at the pharmaceutical company I work for. The VAXen were bullet proof and ran continuously for decades without complaint or failure, dutifully recording data from dozens of temperature probes that were placed amongst the IV bags to endure that the product achieved lethal temperatures for the required time, and was therefore safe to inject directly into patient – which was me once during a hospital stay, so I was very happy to see our logo on the saline bag.
One day the electricians came to me and said they had to shut down the Liebert CRAC units (Computer Room Air Conditioners) for four hours to complete required maintenance to the feeder circuits, and they wanted me to turn off the VAXen. I didn’t want to do it because I owned the campus-wide continuous running computer uptime record, exceeding 7 years, and I wasn’t going to let that record go easily – it’s a geek thing – my computer is better than yours, etc. So, we clamped an amp meter onto the power cables and determined that the VAXen were soaking up just shy of four thousand watts. A tape measure gave us the dimensions of the room. We looked up the R values of the box’s materials, which were concrete floors, insulated steel panel walls, glass windows, and a foam tile ceiling, so we could calculate your equivalent “k” values. We wheeled in an industrial fan to keep the air moving, so conduction and convection ruled, not radiation. The problem was more complicated than your steady state problem, as the (Th-Tc) quantity that drives heat into the walls was changing over time as the room warmed up.
The math said the room would be 85F at the end of four hours.
When the electricians were done at the end of four hours, the room was 85F.
I enjoyed the problem.
Thanks Willis.
My pleasure, Thomas, and thanks for walking through the exercise including the units. Cancelling out the units is something I do on a regular basis in my research, my thanks to Mrs. Henniger, my high school Chemistry teacher for insisting that we do that.
w.
Willis,
To be fair, you should reask this problem, but with a conductive heat source, and a radiative output – just like my original.
Zoe, assuming you’re talking about this problem, I fear your problem is ill-posed. Why? Because it’s not at steady-state.
If the hot end is fixed at 75°C as you show, then it would need to have energy being constantly added to it … but you haven’t included that energy in your problem
You also haven’t included the radiation coming off of the hot end at 75°C. I understand that you don’t think that something can both absorb energy and radiate it at the same time, but if you think about a hot rock in the sun you’ll have to admit that yes, it’s not only possible, it happens all the time. And assuming an emissivity of say 0.95, that end is radiating 791 W/m2 … which doesn’t appear anywhere in your problem.
Without the incoming energy necessary to keep the hot end at 75°C, then the block is not at steady state, and the problem cannot be solved.
Which is why I put up the problem in the head post, which actually is well-posed and solvable, as proven by the large numbers of people who got the same answer that I got, 383K for the hot end and 221K from the cold end.
Until you understand that and can do the calculations for it yourself and get that correct answer, I fear you won’t understand why your problem is ill-posed.
Best regards,
w.
Willis,
I presented a very simple problem you can find in a high school textbook. In fact it’s usually the first problem your presented with when you learn about heat.
Have you used this calculator?
https://www.engineeringtoolbox.com/amp/conductive-heat-transfer-d_428.html
I didn’t specify steady state.
A steady state wouldn’t bring the cold end down from my 50C anyway.
My point was to show how CHF and CSR are related, and no, CSR != CHF at steady state.
I’m glad your guests were able to solve the problem AS IF the sun was a POINT source, rather than the actual Sun square shining on your block.
Do you even understand view factor?
All your guests and you solved the problem wrong. But it makes for good ideological math.
Zoe Phin March 1, 2020 at 12:56 am
Me, I’ve NEVER seen a high school textbook that said one word about thermodynamics, but it sounds like your high school was different.
Perhaps so … so you can either give us a link to said mythical “high school textbook”, or admit you’re just making things up with no evidence.
Your choice.
w.
Willis you are correct about her making that claim about text books ( high school or otherwise ), I challenged her to provide such an example and, guess what, she ignored it.
There is no reason to have the hot end radiate, the temperature and heat flux are given quantities. Nothing is specified about how that outcome is arranged.
The problem is the cold end.
You may find some such idealised thermal conduction example a text book but it will NOT have the little “E=?” tagged on the end. That is her false claim. That does not come from a text book , she added that bit in. SHE MADE IT UP.
That is the fundamental error which leads to her “magic” energy creating block of concrete.
In spuriously assuming that the given heat flow and temperatures are consistent with the cold end evacuating all that flux by thermal emission, she has created a contradictory set of conditions. This leads to the violation of conservation of energy at the boundary and the magical creation of energy.
If she’d been a little more low key about this until she checked it out , she could have just said : oops I made a mistake.
Sadly, after bad mouthing everyone in sight ( and even those she can’t see ! ) and becoming more and more belligerent, she’s painted herself into a corner where she’d need to eat enough humble pie to feed the 3rd world for several years to come.
I’d erroneously assumed she was about 18, since this is the kind of age when people are often this foolish. They have the excuse of youthful inexperience of life. Sadly, she claims to be 34, an age where one would generally say : she should know better.
Oh well, it’s never too late to learn.
Have you? It clearly shows the heat flux going into , through and out of the block. I guess they must be using that damned “ideological maths” too.
BTW , nowhere on that page does it show the cold end being cooled by SB radiation. I wonder why not ? Standard text book stuff apparently. They must have missed school that day.
Thomas Edwardson,
Answers for temperatures carried out to 13 significant figures? Really?
Gordon, it was a joke. He said:
w.
Sorry, I get it . . . but even the Church of AGW and its offshoot, the Church of CAGW, do not provide values—when they provide values at all—to that extreme. 😉
With that result the average of both temperatures is 302 K which would correlate with 471 W/m2 radiated away on average.
This is nowhere close to what is received, which underlines that averaging temperatures in order to determine radiation power is a wrong approach.
If one was to calculate the average temperature needed to radiate away all incoming solar power, the answer would be 335K.
335K is not 302K. Interestingly it is exactly 33 K which seems to be the GHE.
Seems the GHE is just an issue of wrong physics.
Well, we’re rounding up on 500 comments on this thread, far more than I thought it would garner. What have I learned?
• I’ve learned that there are a lot of folks out there who understand this stuff backwards and forwards, in some cases because this is what they do for a living.
• I’ve reaffirmed that there are a number of people out there who don’t understand this stuff at all and are totally immune to facts. For example, despite having likely seen two people with flashlights shine their lights at each other at the same time, they cannot be convinced you can have two opposing streams of photons occupying the same space …
• I’ve seen that there are still folks out there who think that a colder object cannot leave a warmer object even warmer than it would be if the colder object wasn’t there. For them, I can only recommend my post entitled “Can A Cold Object Warm A Hot Object“.
• I’ve been sadly unsurprised by Zoe’s comments. Ah, well, as the old saying goes, “You can lead a horse to water, but teaching it to do the backstroke might be difficult” … or something like that. However, my intention was not to convince her to change her beliefs. It was to dissuade others from adopting her beliefs … and her angry, aggro, evasive comments have certainly been helpful in that regard.
• I’ve been amused by the number of wildly different answers to the question from folks who are totally convinced that they are 100% right.
• And finally, I’ve been happy to see that my original answers to the question and my interpretation of how to solve it agree with the answers of those who know this stuff the best.
Let me say that I don’t expect this summation to end the commenting on the post, nor is it intended to. I just wanted to nail this up as a précis of where we have gotten to so far.
My thanks to everyone who has commented, including Zoe. Even though she’s been disagreed with by those who know this subject the best, she has not turned and run. And while I don’t like that she appears to be totally immune to facts, at least she’s willing to attempt to defend her misunderstandings. Can’t ask for more than that. Well, I guess I could ask for more than that, but it doesn’t appear I’d get it, and I’m a man who tends to focus on wanting what I have and to not worry too much about having what I want.
Regards to all, lurkers, commenters, professionals, amateurs, and casual readers alike, and as always, my thanks to Anthony Watts, to Charles The Moderator, and to the many volunteer moderators for providing us with this marvelous space for public peer review.
w.
Willis,
You’re a hoot. I agree that I’m wrong from the point of view of ideological mathematicians.
If you noticed, I posted videos debunking the claims made here and all I got were crickets.
There are other points I had that went ignored, misunderstood, or ridiculed.
There is ZERO reference to any experiments or scientific literature.
What am I to make of this? Let’s give it another shot.
To be fair, you should re-ask this problem, but with a conductive heat source, and a radiative output – just like my original.
I’m still waiting for an introductory link this amazing field of study which no one seems to have heard of yet apparently we are all practicing without knowing it.
Google drew a blank on that one , can you help?
Maybe it’s a branch of post-modernist philosophy which I need to know about.
In fact , your won refusal to engage in reasoned logical discussion seems decidedly most-modernist. Maybe that’s where we are all going wrong.
“her angry, aggro, evasive comments have certainly been helpful in that regard”
You have to be angry and aggro when dealing with people in fantasyland telling you you’re wrong because their ideological math tells them so.
I didn’t get ahead on walls street by being quiet and listening to bad suggestions.
https://youtu.be/Var3o_eko9U
Anyone doubt that the CHF thru the pan is nearly zero?
Anyone still doubt that CSR != CHF?
Interested mathematicians greatly desire to know what IS the factorial of CSR (CSR!). Please expand on this.
!= mean not equal to 😉
OK, if you say so. But in my mathematical universe the common symbol meaning “does not equal” is “≠”.
Gordon, you are right about the symbol ≠. However, computer programming is done with the regular keyboard. As a result, the “!” has been chosen to stand for negation.
So for example, in the computer language called R, we have a function called “is.finite()”, which tests to see if a given value is finite or is some kind of error.
The negation of that, which tests if a given value is NOT finite, is written as:
!is.finite().
Regards,
w.
Pass. I’ve made my arguments, posed my puzzle, got the same answer as a bunch of actually sane people practicing that evil “ideological math” that’s taught in every thermodynamics class in the world, and made my point.
You have studiously ignored it all, been ugly, abusive, and abrasive, and generally done everything possible to further my goal of convincing people not to listen to your nonsense.
As a result, I fear I have nothing left to say to you. You’ve proven my case that you’re not worth listening to very neatly, my thanks for that, but my work is done here.
So hele on! I wish you well, but I’m not going to continue the discussion. As a number of people have pointed out, you never answer questions, you just take off on some other unrelated track. That’s fine, but you’ll have to go down that track without me.
My best to you,
w.
Willis,
“Pass. I’ve made my arguments, posed my puzzle, got the same answer as a bunch of actually sane people”
That’s nice, and the empirical evidence proves you and them wrong. Sorry. Empiricism wins over consensus.
“You have studiously ignored it all, been ugly, abusive, and abrasive, and generally done everything possible to further my goal of convincing people not to listen to your nonsense.”
I implored people to look at empirical evidence. If their fine with armchair philosophy, then clearly I’m wrong.
But hey, you know your tribe. If a video demonstrating that CSR != CHF can’t convince them of my arguments, then you deserve them. Please take your ideologues with you, and I’ll keep the empiricists. Deal?
But you know what? Maybe I was wrong the whole time, and you have some empirical evidence to show me that CSR = CHF….
I would love to be shown this, and I graciously await your return so I can formally apologize.
Pass, Zoe. I’m taking the advice of the man who said, “Never wrestle with a pig. They enjoy it, and you just get dirty”.
w.
I pointed out straight away your false assumption in your own concrete bar puzzle: you spuriously assumed that the arbitrary 2W flux was consistent with the cold end being cooled by SB radiation. There is not reason for that to be the case and with the values you chose, it is not the case. End of.
You have studiously avoided even commenting on that and realising that your amazing energy multiplying gadget is a simple conceptual error. ( Sadly we are still going to have to pay for every watt we need ).
Clearly you have no intention of admitting you are wrong or even responding to explanations of where you went wrong. That is not the behaviour of someone who is likely to formally apologise about anything.
It is the behavious of someone who is a little too sure of themselves and their level of knowledge. Like a freshman undergrad who has learnt a few basics and imagines he knows it all.
Questioning accepted ideas is good attitude but you have to follow through and back it up. Just coming up with a crazy statement and ignoring counter arguments will go down fine at a flat earther meeting but not with trained scientists and engineers who require answers.
I gained a degree in applied physics , so I’m fine with empirical evidence.
All I see so far is your faked text book example and spurious assumptions. Now where is your empirical evidence?
Are you actually heating your home using one of these concrete blocks with a duracell battery providing the 2W input energy?
Zoe, That cooking video is supposed to be your empirical evidence? Looking at the cold side of the veggie burger?
1) The video shows radiation via a FLIR camera, but it shows nothing about conduction. How are you ‘measuring CHF’ to know how it compares to ‘CSR’ when the only data you have is ‘CSR’ & ‘HSR’ ?
2) The veggie burger never comes to a steadystate condition, so no one would expect ‘CSR’ = ‘CHF’ .
3) There is air in that kitchen. EVEN IF the burger came to a steadystate condition, there would be conductive and convective loses from the top of the burger, meaning
CHF = CSR + CSConvection + CSConduction
Again, no one would expect CHF = CSR.
You don’t seem to even understand what your own evidence is showing.
OMG , that is what she was calling empirical evidence?
I refuse to get into U-tube videos as part of a scientific discussion but thanks for watching it.
So much for the idea of designing a controlled scientific experiment. What a joke.
Tim,
You appear to be not quite right in the head. Did the stove appear to make the top and bottom of the pan the same temperature? Then CHF = 0, and CSR is the hot yellow you see.
The burger was not mentioned. Can’t you read?
“Anyone doubt that the CHF thru the pan is nearly zero?”
Zoe, that is no better!
1) Anyone with a shred of scientific knowledge would indeed doubt that “the CHF thru the pan is nearly zero”. If that were the case, pans could not cook food or boil water. Pans work precisely because they conduct heat very well.
2) The fact that the FLIR sees intense IR radiation is proof that there is large “cold side radiation” — lots of energy leaving the from the top of the pan. This means there must also be lots of energy being supplied to the top of the pan = large “CHF” ( conductive heat flow) resupplying the energy being lost via radiation.
Tim,
You appear to be not quite right in the head. Did the stove appear to make the top and bottom of the pan the same temperature? Then CHF = 0, and CSR is the hot yellow you see.
The burger was not mention. Can’t you read?
“Anyone doubt that the CHF thru the pan is nearly zero?”
What is the resolution of the measurement that allows you to state that the top and bottom temps are the same?
Pans of different materials have completely different thermal characteristics.
The amount of heat conducted through and aluminum pan is an order of magnitude higher than that through a stainless steel pan under the same conditions.
Can you give a coherent explanation of how heat arrives on the top surface of the pan if it is not being conducted through the pan?
I seriously doubt it.
Prove me wrong.
All you ever do is say stuff.
Wrong stuff.
You remind me of the guy who used to come around arguing that there was no such thing as convection, and that meteorology was a fake science, water vapor was heavier than dry air, everything was caused by “vortexes” that only he know anything about.
He was similarly immune to facts, insulting, and by all indications 100% uneducated in anything resembling any actual science.
BTW…here is the source for aluminum vs stainless conduction rate, at bottom of the page:
“Conductive Heat Transfer through an Aluminum Pot Wall with thickness 2 mm – temperature difference 80oC
Thermal conductivity for aluminum is 215 W/(m K) (from the table above). Conductive heat transfer per unit area can be calculated as
q / A = [(215 W/(m K)) / (2 10-3 m)] (80 oC)
= 8600000 (W/m2)
= 8600 (kW/m2)”
“Conductive Heat Transfer through a Stainless Steel Pot Wall with thickness 2 mm – temperature difference 80oC
Thermal conductivity for stainless steel is 17 W/(m K) (from the table above). Conductive heat transfer per unit area can be calculated as
q / A = [(17 W/(m K)) / (2 10-3 m)] (80 oC)
= 680000 (W/m2)
= 680 (kW/m2)”
https://www.engineeringtoolbox.com/thermal-conductivity-d_429.html
Any explanation yet, why the theoretical average temperature and its associated Emission Power is 33 k apart from the real physical value?
I’d like to propose a different experiment.
You have a one meter cube box which is completely covered with unobtainium. (is that name trademarked by the Pandorans?)
On the inside wall of the is covered with gold leaf, which is very reflective. 1 centimeter in is another cube with a layer of vantablack on the outside, and a layer of gold leaf on the inside. Inside that box is another box of the same specification with vantablack on the outside and gold on the inside continuing until you have a small 2 centimeter box in the middle.
The space between each of the cubes is filled with air at a temperature of 100 C at sea level pressure. The walls with vantablack and gold also start at 100 C.
What will happen as vantablack absorbs any emissions and re-emits those emissions equally inward and outward. Given that gold is highly reflective, and vantablack is highly absorbing, would the energy of this system tend to rise as you get to the center and lower at the outsides until some steady state occurs?
The question my be ill formed by my ignorance of blackbody radiation and laws of thermodynamics, but the question has bugged me for decades. Thanks.
Jeff, IF I understand your experiment, eventually the entire apparatus would be at ambient temperature. Neither gold leaf nor vantablack is a perfect relflector or absorber, so the end result would be that they would all trend to the same temperature.
At least that’s my answer, but I’ve been wrong a few times. Well, more than a few times if you insist on brutal honesty … but that’s my answer.
I say that temperatures will equalize in part because if it were not true, if there were a persistent temperature differential, then we could use it to run a heat engine forever … perpetual motion. No can do that.
w.
I think the air rather negates the though experiment since conduction and convection would equalise temps. If you evacuate the the whole thing, I think that is maybe more like what Jeff wanted to imagine.
If we also replace imperfect materials with perfect ones we remove the get-out clause and get back to basics.
In that case I would say that the black surface would emit given total power, the ungoldium would prefectly reflect it and it would be perfectly absorbed by the ideal black surface. Each cavity would remain in just the same state as if started.
Even if there was a temperature difference between the outside and the innermost cavity this would remain unaltered since there is no mechanism : radiative , conductive nor convective to transmit heat between them.
Any other set up short of this ideal would eventually lead to equal temperatures throughout: 2nd law.
Zoe, I woke up in the middle of the night and I thought “Dang! How did I miss that?”
What I realized was that in the midst of all of your dodging, and redirecting the conversation, and plain and fancy tap dancing, and talking about “ideological mathematics” and such, I’d totally overlooked a simple fact:
You never answered the question in the head post.
So, since you hold yourself out as an expert in these matters, much more knowledgeable than us mere mortals, could you please use your “non-ideological mathematics” and tell us how hot you think the two sides of the block will end up?
As requested in the head post … please show your work.
Many thanks,
w.
My thoughts went like this. Starting at ambient temperature 100 C of everything, the gold would emit at some frequency of IR. The vantablack would absorb 99.99 percent o that. Half the energy would go to the gold on its other side, the other half would be re-emitted back at the gold that emitted it first. Gold would reflect most of it at around 95% iirc. The decrease in energy of the outermost cube would be replenished by contact with the air with the air decreasing ever so slightly. The radiation doesn’t affect the air temperature and gets emitted to the black surface. In mean time the energy transmitted to the next layer of gold gets emitted to the next black layer. The air slowly gets cooler. And so forth.
In this particular scenario with the perfect insulator on the outside. The total energy of the system stays the same. The energy moves about depending on the properties of the stuff it comes in contact with. Were the box just full of air, the individual molecules would be transferring energy with each collision with some locations occasionally warmer than others. The energy moves about, but the whole system stays the same. I was applying stuff we know such as if you paint your house black, you’ll be hotter than if it is covered in mirrors because the radiation behaves differently depending on what it comes in contact with. If you take the universe as a whole, the total energy of the system isn’t changing, but in our little part of it, we make heavy uses of the energy potential differences to extract work from it. So I came up with this closed system idea to see if one could create an energy difference in one part of an area to be converted to work, and then back to heat and then start over again.
So when you say “if there were a persistent temperature differential, then we could use it to run a heat engine forever … perpetual motion. No can do that.” I was simply adding the word “yet.”
This is a thought experiment because I don’t have the resources to perform the actual experiment, but there are people who do. I’d like to see the actual experiment performed. Like you, I’m highly sceptical of the idea, but can’t seem to shake the idea. Either way, we’d learn something and have something to throw at all the perpetual motion proponents who like to scam people with their ideas. Like you, I’ve been wrong enough times to not take thought experiments too seriously. The real world has a nasty habit of popping bubbles.
“This is a thought experiment because I don’t have the resources to perform the actual experiment, but there are people who do.”
There are people that can make a set of nested boxes that do not touch each other?
Who are those people?
Besides, vantablack only has the properties you are thinking of for some set of wavelengths and perpendicular rays.
And it does not absorb 99.99%, it is 99.96, and that is for visible light with perpendicular rays.
The numbers are less for other wavelengths and angles.
It would not matter anyway.
There is no magic box with nested cubes that never touch.
And it would have to have a perfect vacuum, also impossible.
And gold does not reflect perfectly, just very well, and again it does so best at certain wavelengths.
No one has made a perpetual motion machine YET because never is a long time.
It is impossible, because nothing is perfect.
No process is perfect, and if you can invent a better version of Maxwell’s Demon, it will not work any better than his version, so why go to all the trouble of nested boxes and exotic materials?
Just have a little guy that only let’s slow molecules (or the fast ones…either will do the trick) past a door he opens and closes real fast.
As you have laid this out, there is no temperature difference, thus there is no (net) heat transfer, the system is already steady state and will remain exactly as you have described it.
Zoe, I woke up in the middle of the night and I thought “Dang! How did I miss that?”
What I realized was that in the midst of all of your dodging, and redirecting the conversation, and plain and fancy tap dancing, and talking about “ideological mathematics” and such, I’d totally overlooked a simple fact:
You never answered the question in the head post.
So, since you hold yourself out as an expert in these matters, much more knowledgeable than us mere mortals, could you please use your “non-ideological mathematics” and tell us how hot you think the two sides of the block will end up?
As requested in the head post … please show your work.
Many thanks,
w.
Hi, Willis.
I answered the question with the very first post I made:
“Zoe Phin February 28, 2020 at 9:55 am
Assuming there is no matter past the cold end, the final temperature of both sides will be equal.”
Both sides will be ~120C.
Now answer my questions:
https://youtu.be/Var3o_eko9U
Does the top and bottom of the pan not get to the same temperature?
Doesn’t that make CHF = ZERO?
And CSR = whatever bright yellow is?
And don’t tell me there’s cond/conv/ective losses all over the place. There’s also sideways radiative losses. All those things are not advantages, but disadvantages to reaching equilbrium.
Sorry, Zoe, but you didn’t answer it because you didn’t show your work.
As to your video, you ask “Does the top and bottom of the pan not get to the same temperature?”
No, they don’t.
w.
toolbox :
k=70 , A=0.1 , T1=502, T2=500 , s=0.01 Calculate ! Heat transfer = 1400W ( right ballpark for the cooker ).
Do you really think your thermal imager will detect that kind of temperature difference on the scale you are using?!
No, the top and bottom of the pan does NOT get to the same temperature but your pathetic “experiment” is not able to resolve that fact because you did not design an experiment capable of doing so.
The only empirical evidence I see is that you have no idea what you are doing.
Greg,
Yeah, I saw this coming. Deny that the temperature is the same and fabricate your own numbers.
Hey, poophead, why don’t you model this with Willis’ method. Go ahead. No excuses!
Hey poophead, why are insults such an essential part of science for you? Go over the CAWG camp, that is one of their qualifications.
Oh dear, you used the D word , see what a I mean?
I did not “fabricate” the numbers any more than you fabricated those in your example. I used what are reasonable figures to illustrate what will be happening with your frying pan. If you had half a brain you would have done that before designing your experiment and would have ensured that it was capable of proving something instead of shouting from the rooftops that you had “empirical evidence ” when you have nothing.
The calculation shows that, if there was a finite heat flow consistent with the accepted view of thermal conduciton, your pathetically incompetently designed “experiment” would be incapable of detecting it.
As such it is not “empirical evidence ” of your claim but clear empirical evidence that you do not understand squat about physics or how to design an experiment to test a hypothesis.
Zoe Phin March 1, 2020 at 9:56 am
Wonderful. More tap dancing. ANSWER THE DAMN QUESTION!!! How does the cold end know that there is no matter past the cold end?
w.
Well, it is light, so it must be able to see, eh?
How else could it know where it was going?
And a distant star has to not only be able to see, before it dares to shine any light on the Earth, but it must be able to anticipate what will be where, in tens, to hundreds, to billions of years. Obviously.
Hubbell Deep Field has objects that shone their light at the telescope over ten billion years ago, before the solar system formed, and before the large stars that went supernova to seed the cloud that became out solar system and us with heavy elements, had themselves formed and exploded.
And it was way before we decided to build that telescope and put it there…of course, before the Earth was even a glimmer in the solar proto-disc’s eye. Yeah, before the elements that went into the make up of the disc were even created in those supernovas.
That kind of makes a mockery of the speed of causality…but what the hey…light cannot shine at nothing…
So says Zoe!
Pharaoh had nothing on this gal.
Zoe, one other comment. You asked if I’d seen the online calculator for this. When I looked at it, I understood why you make the claim that you do.
If we set up the online calculator with the setup you use in your problem, with one wall at 70°C and the other wall at 50°C and all the rest, we indeed get a flux of 2 watts just as you say.
However, you then make the claim that there are only two watts flowing through the wall, but the cool side of the wall is radiating at a Stefan Boltzmann level around 590 W/m2. You use this as evidence for your theory that the conductive heat flus is different from the cold side radiation.
What you are not taking into account is, why is the cold side at 50°C and why is the hot side at 70°C?
The answer, of course, is that they are held at those temperatures by whatever is heating the rooms on either side of the wall. That energy, the additional energy needed to maintain those temperatures, is NOT taken into account in your formulation, and it leads to your incorrect conclusion.
I’d hoped to make this obvious by restating your problem in outer space. There, we can see that there is nothing heating the cold end of the block. And as a result, all of the energy being radiated by the cold end has perforce must, come from within the block itself.
And this is the reason why the conductive heat flux through the block has to equal the amount radiated out the far end. This requirement forms one of the two simultaneous equations that everyone solved to get the correct answer.
But heck … give us your answer, using your “non-ideological mathematics”, and we can all see how you would calculate the temperatures of the two sides of the block in the head post.
Regards, I’m off to burn some fallen tree limbs in the back yard, I’ll check back in a bit.
w
Willis,
Answer my question:
https://youtu.be/Var3o_eko9U
Time: 01:57
Does the top and bottom of the pan not get to the same temperature?
Doesn’t that make CHF = ZERO?
And CSR = whatever bright yellow is?
Your ideological math:
The first thing you would’ve done is compare the surface area of the pan to that of the planar fire head touching the pan.
Bam! You’re already on the path to the WRONG answer.
Bam, you ignore everything that was said go back to your totally uncontrolled frying pan again.
Willis has just restated what I pointed out to you about 3 days ago when I initially commented on your blog. You are spuriously thinking the cold end is cooled by SB radiation and there is NO REASON to suppose that. Indeed it is incompatible with the rest of the conditions you laid down.
You confused yourself an made a simple error based on a spurious assumption. It’s that simple.
The arbitrary values you chose do indeed give Tc as 50 deg C , as I said you have that much correct. Sadly, the good bit it ends there.
The engineers toolbox is the closest you have come to supplying an example of this kind of “text book” calculation and the conspicuous difference is that they say nothing about SB radiation at all. So your claim about your original exercise remains a false claim.
Your refusal to address this by this stage makes it pretty clear you realise this, that you have no answer or counter argument and so your last line of defense is to divert and hope it works.
500+ comments indicates that is a failed strategy.
One definition of insanity is repeating the same behaviour and expecting a different outcome. You are doing a lot of repetition here.
“You are spuriously thinking the cold end is cooled by SB radiation and there is NO REASON to suppose that.”
LOL. Projection. Actually you and Willis believe that, and I don’t. If there is no matter past the cold end, there no heat transfer. The 557 W/m^2 is a radiating potential.
Why is that sophists ignore empirical evidence in the video? Oh that’s right, they’re low life sophists – it’s all they can do.
Zoe Phin March 1, 2020 at 9:12 am
Um … I hesitate to ask, knowing that I’m just gonna end up going down a Zoelian rabbit hole but … how does the cold end know that there is no matter past the cold end? Radar? ESP?
And is it an “all or nothing” kind of deal? I mean, if there is one molecule of matter out there, does the cold end suddenly begin to radiate full bore? Or is the amount of radiation proportional to the amount of matter?
Next, does the matter have to be in a perfectly straight line perpendicular to the flat end, or anywhere within the field of view?
Next, if you pick an arbitrary line and follow it far enough out into space, sooner or later you’ll hit matter … does that count? (This is particularly true if the answer to the previous question was “anywhere within the field of view …).
Finally, could you point us to one, just one, text of any kind that says that solid objects above 0K only radiate when there is other matter around?
Regards,
w.
“radiating potential” another made up term of your new physics world.
No radiation to space ? Another invention of your new physics.
More insults.
I’ll repost what I just posted about you “empirical evidence”.
toolbox :
k=70 , A=0.1 , T1=502, T2=500 , s=0.01 Calculate ! Heat transfer = 1400W ( right ballpark for the cooker ).
Do you really think your thermal imager will detect that kind of temperature difference on the scale you are using?!
No, the top and bottom of the pan does NOT get to the same temperature but your pathetic “experiment” is not able to resolve that fact because you did not design an experiment capable of doing so.
The only empirical evidence I see is that you have no idea what you are doing.
” If there is no matter past the cold end, there no heat transfer. ”
So what happens to the 2W arriving at the cold end, why does it not get hotter with all that thermal energy arriving with nowhere to go ?
That’s the problem with rewriting one of the laws of physics you end up needing the rewrite the rest and still come up with a self-consistent , coherent theory.
Good luck with that.
So now your problem is where is happening to the 2W ?
Willis,
“how does the cold end know that there is no matter past the cold end? Radar? ESP?”
Yes Willis, “ESP”. You never read how Planck derived his law?
Waves only form between matter.
If the separation distance between objects is 1 meter, no 2m wavelength will form.
Why do you think not all photons are possible? What do you think is the basis for Quantum Mechanics.
According to you, photons are emitted willy nilly, so ALL photons should be possible.
Supposing there is some matter about 13 billion light years away, how long will it take you “radiation potential ” to resonate with that and start emitting?
Just wondering because we don’t know the rest of your new physics yet.
Willis,
‘”As to your video, you ask “Does the top and bottom of the pan not get to the same temperature?”
No, they don’t.’
My pixel analysis shows you to be a liar. You didn’t analyze anything before you spouted that convenient self-serving lie.
There’s dozens and dozens of real-time thermal videos on youtube showing you that you’re wrong. You ignore it all and call yourself honest. Hilarious!
But please don’t kill yourself. That would be a very awful thing.
The bottom side of the pan is in contact with an open flame with a temperature of ~3560°F, and the top of the pan is in contact with a cold slab of some cold food-like substance and room air that is probably in the range of 68-78°F.
It takes time for heat to flow through any material, whether you believe in that or not.
And you somehow have the idiotic notion that the two surfaces are the same temperature?
It is obvious at a glance that you make zero sense, but it takes some thought to understand the true extent of your ignorance and belief in an unphysical reality.
“Um … I hesitate to ask, knowing that I’m just gonna end up going down a Zoelian rabbit hole but … how does the cold end know that there is no matter past the cold end? Radar? ESP?”
How about field radiation pressure? You know, that which you denigrate, while not explicating a satisfactory replacement mechanism for absorption / emission regulation in accord with 2LoT, therefore you tacitly must claim all matter emits as though its in a 0 K ambient, and absorbs as though its in an ∞ K ambient?
You only deny radiation pressure, 2LoT, the Work-Energy Theorem, Stefan’s Law and due to that, pretty much everything else. LOL
You wrote: “Heat can’t flow from cold to hot, but radiated energy absolutely can.” That violates 2LoT, and is self-contradictory. Heat is definitionally an energy flux, so you’ve essentially just written “energy can’t flow from cold to hot, but radiated energy absolutely can”… as though radiated energy is a ‘speshul’ kind of energy that can violate 2LoT. Of course, in this context, “heat” is “radiated energy”… so you’ve self-contradicted in a particularly ironic way. LOL
Energy is a measure of the ability to do work… just how, exactly, can a photon of lower potential do work upon an object of higher potential? Or are you going to follow the climate catastrophist guidebook and claim radiative energy transfer “has nothing to with the photon’s ability to do work”… you’ve already tacitly done so in your denial of the Work-Energy Theorem in your claim that radiated energy can violate 2LoT.
Bet you didn’t know that Rice University measured the temperature of a single molecule by having a deep understanding of 2LoT as relates to radiative energy transfer, huh. That, if you care to look it up, proves you wrong on your blather about “Heat can’t flow from cold to hot, but radiated energy absolutely can.”
You’re yet another of those ‘colleeg edumacated idjits’ with equations you use like they’re black-boxes (plug in the numbers and out pops an answer… is it correct? Who knows. It’s an answer, though!), sans any deep understanding of the concepts behind those equations, and you’ve bought into a wide swath of the climate catastrophist weltanschauung.
I’d politely encourage you to educate yourself, but “you are far too arrogant and self-important to follow my polite request” (your words), so instead, I’ll raucously laugh at your perpetual and self-imposed confusion. LOL
You do no one any good deed in leading them astray while purporting to educate them… educate yourself first… you don’t even have a grasp on the basics.
It will equalize between the two sides!
Smart man
Robert,
Walk outside on a sunny day.
Find a stone wall, or just a stone, that has the sun shining on one side only.
Now, feel the sunny side.
Then feel the other side.
Then wait several hours for the stone to warm up some more.
Feel both sides again.
The think about what you just said.
Come back and offer your opinion again based on the actual world and how things actually work.
Zoe Phin March 1, 2020 at 9:56 am
Wonderful. More tap dancing. ANSWER THE DAMN QUESTION!!! How does the cold end know that there is no matter past the cold end?
w.
Willis,
The answer is: mu.
That’s the proper answer to a question that already presupposes something.
Have you stopped beating your Wife?
Your question presupposes the Corpuscular Theory of Light as valid. It’s not.
Come on, Willis, why are not all photons possible (the premise of Quantum Mechanics)?
Think.
Zoe, it is YOUR theory that the cold end will not radiate (despite being above zero K) if there is no matter beyond the cold end. Not mine. Yours.
I’ve simply asked, how does the cold end know that there is or isn’t matter there? Simple question about YOUR theory.
I “presuppose” NOTHING. It’s your theory, so if there are presuppositions, they are yours.
So once again I ask that you stop the ridiculous tap-dancing and ANSWER THE SIMPLE QUESTION ABOUT YOUR OWN THEORY.
w.
Willis,
Why are you such a liar?
Of course you presuppose that matter MUST emit to nothing.
What’s your scientific basis for that?
Zoe Phin March 1, 2020 at 2:06 pm
Listen, you vile little pissant. I don’t lie. You must be thinking of your friends, or perhaps you are looking in a mirror, but you’re not looking at me.
I am an honest man, and I tell the truth as best I know how. Obviously, you have little experience with my type of human.
I was brought up under what in my family was called the “Captain’s Code”. “The Captain” was my great-grandfather, a Mississippi riverboat captain. One part of his Code goes as follows:
Now, this isn’t the 1870’s when he was alive, so I don’t kill anyone for that. However, I’ve done my best to live by that maxim, and I don’t put up with your kind of ugly crap from anyone, man, woman, or child.
I’m done with you. Your endless insults have finally brought me to do something I rarely do, which is to flat give up on someone because they’ve proven that they have no honor. You’ll have to peddle your garbage to someone else. You’ve cancelled your vote with me. All I’m going to do with your madness from here out is to point and laugh.
w.
Zoe,
You have a Nobel Prize waiting for you, so why not claim it.
And you need to go and rewrite a whole bunch of Wikipedia article as well.
I am sure everyone will be grateful for your having corrected these erroneous statements, such as this one:
“Thermal radiation, also known as heat, is the emission of electromagnetic waves from all matter that has a temperature greater than absolute zero.[3] It represents the conversion of thermal energy into electromagnetic energy. Thermal energy consists of the kinetic energy of random movements of atoms and molecules in matter. All matter with a temperature by definition is composed of particles which have kinetic energy, and which interact with each other. These atoms and molecules are composed of charged particles, i.e., protons and electrons, and kinetic interactions among matter particles result in charge-acceleration and dipole-oscillation. This results in the electrodynamic generation of coupled electric and magnetic fields, resulting in the emission of photons, radiating energy away from the body through its surface boundary. Electromagnetic radiation, including visible light, does not require the presence of matter to propagate and travels in the vacuum of space infinitely far if unobstructed.”
https://en.wikipedia.org/wiki/Thermal_radiation
The fact is you have no idea what the hell you are talking about, and everyone here knows you are one of the stupidest people ever to show up.
People like you are why humanity was stuck believing nonsense for all of time up until logic replaced doubletalk.
Nicholas,
No, I think you deserve a Nobel Prize. Quoting Wikipedia uncritically is the height of genius.
Look up the sources.
^ K. Huang, Statistical Mechanics (2003), p.278
^ K. Huang, Statistical Mechanics (2003), p.280
^a b S. Blundell, K. Blundell (2006). Concepts in Thermal Physics. Oxford University Press. p. 247. ISBN 978-0-19-856769-1.
Zoe, you said:
“Quoting Wikipedia uncritically is the height of genius.”
But what I said was the opposite of what you claim.
I said:
“I am sure everyone will be grateful for your having corrected these erroneous statements, such as this one…”
Does that sentence sound uncritical to you?
As anyone can see, I said the opposite of what you claim.
So maybe that is what is the matter with your brain…you have the reading comprehension of my cat. And not the smart one…the dumb one.
Zoe Phin March 1, 2020 at 8:07 pm
“… Nicholas, No, I think you deserve a Nobel Prize. Quoting Wikipedia uncritically is the height of genius … .”
—
What Nicholas quoted was consistent with Richard Feynman’s Quantum Electrodynamics 1961 lectures–which you should read.
Nicholas,
You’re an immature child. Your argument is still: Wikipedia said so, so it’s true. You didn’t demonstrate that waves grow from nothing to something over long distances.
If you look up Wikipedia “photon”, then you get several alternative views. And since there are alternative views that which you quoted is not the final word.
This aint about me Zoe…it is about you.
You know, the one who has no idea what the hell she is talking about.
I take back the part about you having the same reading comprehension as my stupidest cat.
Obviously you have no comprehension at all.
And you hallucinate…wildly.
I know why you have to pretend that I said what you hallucinated.
Because if you did not, you would actually have to offer your rewrite, which is what I actually said.
And obviously you have as much ability to rewrite physics as the lizard my cat is playing with.
Face it Zoe…you are a complete failure.
You cannot even spin tall tales very well, let alone bullshit your way through a coherent explanation for any of the malarkey you have invented.
Greg,
You blab so much I need to start a new thread.
“k=70 , A=0.1 , T1=502, T2=500 , s=0.01”
How nice you fabricated your own numbers in order to justify your ideology.
I screen-captured the video @ 1:53, just as the pan was being removed. I compared the pixel color just below the burger to just above the fire.
They are exactly the same!
As you move up above the burger, it does decrease very slightly, and perfectly reasonably, as that part of the pan is much farther from the fire, and had time yo cool.
You claim the resolution is not good enough and that’s a complete self-serving lie.
I counted 53,915 unique colors in the image.
The resolution is just fine.
LOL, as if there was just one video on youtube showing top T matching bottom T in all sorts of mediums (metal, water, wood, hard plastic), instead of DOZENS and DOZENS.
“I compared the pixel color just below the burger to just above the fire.”
How nice you fabricated the zone you look at to justify your ideology.
“just above the fire” ? What the hell kind of sampling method is that?
What is the specified resolution of the device? Sure, you will have noise and can pick as many convenient pixels as you want. Find two that are the same and say “bingo!” I’ve proved my case.
How many different colours are there is those white blobs? The whole pan and the burner looks the same temp : white, you’ve just saturated the device. You have no resolution at all at that end of the scale, that’s why they look the same “temperature”.
You are pretending that both sides of the pan are the same temp but you have no shot of the underside of the pan, so you are not even measuring what you claim to want to know.
Go away and design an experiment which would be capable of detecting a difference of less than a degree at that temperature and start again.
Anyway you are so ridiculously disingenuous since your big mouth has got you into a position where you can’t back down. You have to just keep going whatever, so there is absolutely no point in continuing to converse with you.
It’s always interesting looking at new ideas but your attitude is beyond a joke. As Willis said you have made it pretty plain for everyone to see that can not be taken seriously and you fell for the bait.
Bye-bye “poophead”, it was fun playing with you.
The camera is designed to work -20C to 650C.
It is not saturated. The pixel color is #FDFCE7, not #FFFFFF
“How nice you fabricated the zone you look at to justify your ideology.”
Center of the fire and as close to the center of the pan as possible. By Willis’ theory there should actually be a radial gradient as well.
“Go away and design an experiment which would be capable of detecting a difference of less than a degree at that temperature and start again.”
The camera is capable of 0.05C degree discernment. The software that comes with the camera rounds to the nearest 0.1.
The camera man chose not to display temperatures.
Question: What experiment validated Willis?
Oh it’s in a textbook? Well, I guess we’re just supposed to presume it’s been validated, right? No textbook has ever had falsehoods in it?
Sure Greg, all those videos simply have noise and saturated the device. Yeah right.
Why do people like you exist?
No evidence will uproot your ideology that has no evidence, other than theoretics, to support it.
Are you a Maoist like Willis was? They’re just as convinced of their own B.S.
Zoe’s solution with both sides at a temperature of 120 °C is more or less possible in a universe in which the hemisphere visible from the backside of the concrete bar is empty. For the same reason the Sun would not radiate if it were alone in space and no other bodies could absorb its radiation (Tetrode’s star) the backside would not radiate. That does not agree very well with the fact that the frontside is heated by the Sun.
Teerhuis, you blithely repeat Zoe’s curious claim that if something is alone in space it doesn’t radiate. Neither of you have provided the slightest scrap of evidence that this is true, and it seems totally bizarre.
As I asked Zoe, how would some inanimate object know whether it is “alone in space” or there was some kind of matter around it? What if the “matter” is one other star 50,000 light years away? Would the sun radiate in that situation?
w.
Willis,
You are an ideological twit. You were asked to explain several times why some photons can form and not others. You don’t give a hoot about science, just pushing your ideology. Why don’t you just read how Planck derived his formula? He couldn’t have done it believing matter just sends photons to anything.
What we learn from Planck is if the separation distance between MATTER is 1 meter, no 2 meter wavelength photon will form, got that? There is NO 2 meter photon. That means the objects “know” the distance between each other and “know” not to send a 2m photon.
But in your theory, all photons are possible because objects don’t care what’s around them. They are dumb in your view, and just send whatever. Well this view does not yield EMPRICIAL results, so this view is wrong.
You got that?
Willis,
May be Zoe’s curious claim is from a comment of mine on her website. I read the argument about Tetrode’s star on Math Pages:
https://www.mathpages.com/home/kmath610/kmath610.htm
No, I did not take it from you.
I read science.
A smart person can understand that standing waves can only form with a “rope” that’s attached on the other end.
But Willis is not a smart person, and believes the rope simply grows in length and can wave with fixed amplitude anyway, and then attach itself to something. Yup, he’s a growing rope believer. How silly.
Zoe, you are expressing a belief in something called an luminiferous aether, which Michelson and Morley demonstrated was unlikely to exist, and by the 1920s it was considered prove that there was no aether.
Explain redshift and blue shift with your rope analogy.
You seem to fixate on analogies and transmute them into exact parallel situations inside your mind.
Read and learn.
Most people here probably knew all of this when they were teenagers.
You are not smarter then everyone else, you are dumber.
A veritable cement head.
https://en.wikipedia.org/wiki/Luminiferous_aether
Michaelson and Morley’s famous 1887 experiment did not get a null result as theologians of Corpuscular Light Theory will have you believe.
In fact the experiments were perfected and carried out again, also showing serious results.
But of course you don’t know that.
Zoe,
Planck assumed a cavity with perfectly reflecting walls, then there are only standing waves in the cavity. But later it was proposed that the ‘oscillators’ in the walls absorb and emit photons, so the photon gas is not restricted to standing waves.
How about a supernova, Teerhuis?
Does it only explode when every photon has a destination printed into it’s itinerary?
If a gamma ray burst shoots out to the whole universe when two black holes collide, can they only collide once every gamma ray photon has it all worked out where it will end up?
LOL@Klimate Katastrophe Kooks March 10, 2020 at 10:10 am
I keep asking you the same question and you keep ignoring it … so I’ll ask it again.
If your theory about “field radiation pressure” is correct, how can two flashlights shine directly at each other? Per your theory, the “field radiation pressure” from the stronger flashlight should prevent the weaker flashlight from shining at all.
But obviously, that’s not happening … why not?
First, heat is NOT radiated energy. Heat is the spontaneous flow of net energy from a warmer to a cooler object. Or to use the Encyclopedia Britannica definition:
Here’s another definition:
Note that NOT ALL ENERGY IS HEAT. Heat is a particular form of energy—the energy that flows spontaneously from warm to cold.
If we have two bodies within each others’ “view field”, each one is transmitting radiant energy to the other object. However, the flow of heat is the NET flow of energy, which always goes from the warmer object to the cooler object. So no, there is no violation of the Second Law of Thermodynamics. Radiant energy goes both ways, but in conformity with the 2LoT, the heat flow only goes one way, from a warm object to a cool object.
Regards,
w.
LOL@Klimate Katastrophe Kooks March 11, 2020 at 10:34 am Edit
Heat is indeed an energy flux. But all energy fluxes are NOT heat. This is Thermo 101 stuff. Get a textbook and stop making a public spectacle of your ignorance.
w.
Comment on Zoe and Willis:
“how does the cold end know that there is no matter past the cold end? Radar? ESP?”
The emission and absorption of a photon is one single event, despite that in our reference frame there can be billions of years between both events. Try to travel with a photon. There is no such thing as a ‘free photon’. The Sun radiates so its photons will be absorbed no matter how far the absorbing bodies.
If it is assumed that the Sun can radiate and can heat the front side of the bar then the backside can also radiate.
You are ignoring the case where there is nothing in the path of the photon, ever.
Look at the Hubbell Deep Field.
See all the places with nothing there?
You are taking the case of a photon that hits something and inventing the idea that there is no other case possible, with no evidence.
Nicolas,
In the reference frame of the photon there is no time and distance. You cannot know very accurately what is beyond the observable universe. But I admit that this is not a very practical way to describe spectroscopy. It is much more useful to describe the emission of a photon as the result of a coupling of a particle with a vacuum-state (see Feynman’s work).
Chicken and egg.
Whether you call it a speed of a distance, the photon cannot know where it will end up when it leaves.
Does it have a little homunculus riding along with it, peering out a porthole prior to embarking, and knowing that in billions of lightyears of distance, objects that do not even exist yet will bend it path through multiple lensing events, and eventually impact some atom of matter than has not even created yet?
The entire notion of knowing where it will end up before it leaves can be disproven by reduction ad absurdum.
”’speed or a distance…
Willis:
“Listen, you vile little pissant”
How Lovely.
This is what happens when you try to educate someone that doesn’t understand their ideological posession.
No experiment and no reasoning will suffice. And expect to be accused of the same thing.
Whatever. Like I said Willis, you can have your ideological nitwits that believe they understand science, and I’ll stick with Planck and empiricism.
He is frustrated with you, Zoe, because what you are writing is nonsense, and because you steadfastly refuse to learn.
You are only hurting yourself, but it is a common problem. I often encounter people who erect impenetrable barriers to their own learning, by refusing to ever admit, even to themselves, that they were confused or mistaken, about anything of consequence.
They apparently think that admitting an error would be admitting weakness, and that is something that they will never do. The truth is just the opposite. Everyone makes mistakes; it is how you handle them which reveals your strength, or weakness. A readiness to admit errors, correct them, and learn from them, is a sign of strength. It is evidence of intellectual integrity. It is the unwillingness to admit errors that proves weakness.
– Jack B. Sowards (writer), as the character Lt Cdr Data (Brent Spinner), TNG S2E2
https://archive.is/MlOiz#selection-3467.9-3469.1
https://youtu.be/8eDYVtPwWiM?t=43
Hilarious!
Even though there’a dozens of thermal imaging videos on youtube refuting your claims and supporting mine, you ask me to acknowledge my “error”.
Wow. What a pathetic sore loser … projecting his gross incompetence.
Zoe says: “If the separation distance between objects is 1 meter, no 2m wavelength will form.”
This is small point in larger discussions, but it goes to show once again Zoe is misinformed. A standing wave between two objects/surfaces/ends a distance D apart can have wavelengths of 2D/n.
So when the distance is 1 m, the allowed wavelengths are
2m, 1m, 2/3 m, 1/2 m, 2/5 m, ….
Yes, 2 m is indeed allowed.
Yes, Tim, I dumbed it down so that I don’t have to retype the same thing on my smartphone the first 8 times I tried to explain reality to Willis.
Willis would mock what you just said and call it ESP.
Nice try liebaby.
That one was truly laughable.
You ‘dumbed it down’ by giving wrong information? How is that supposed to strengthen your case?
Tim,
When Willis ignored my claim the first 2 times I told him this on my blog, he ignored it. Then I started writing it wrong, so he could have a chance to refute it. This would at least have shown he read something. He did not.
Thanks for agreeing that not all photons can form and therefore objects don’t emit photons willy nilly.
You have refuted Willis. That supports my case.
I have refuted one of your claims about cavity radiation (standing waves within a closed, reflective chamber) — correcting your incorrect claim about the allowed wavelengths. Since Willis is making no claims about cavity radiation, then you have refuted nothing he said.
Most of the world is not a cavity resonator, but there are still rules about the allowed wavelengths of light emitted by materials. These are related to the quantum mechanics of atoms & molecules & crystals (not the quantum mechanics of cavity resonators).
Here’s another video:
https://youtu.be/LckKhwJJgtE
According to Willis et al:
Since the incoming surface area is much smaller than the outgoing radiation surface area, and because of much convective surface area, we should expect to see a very steep “steady-state” gradient form where the top is at the very least twice (more like 4x) as cool as the bottom.
And yet reality shows different.
And remember, Willis is an “honest” guy.
Moderators:
I posted a longish comment here last night at around 8:00 EST that has not appeared.
Any sign of it in the moderation bin?
I did get a notice it went to moderation, although reading through it I could not see why.
Thanks,
Greg and Willis,
The Saints are jealous of your seemingly infinite patience.
But I am wondering if there is a such thing as being too patient?
At some point it becomes obvious one is dealing with a pathological mind, and it becomes unethical to humor them.
Such people live for the confrontation.
Consider the antivaxxer guy who insists there is no evidence whatsoever than any vaccine has ever done anyone any good, ever, at all.
This is the same thing.
Imagine the nerve of this brat who calls Willis a liar?
Who claims Greg does not know actual physics?
I personally know people like this, have my whole life, and I know that what really motivates them is awareness of their own ignorance, and crushing feelings of inferiority coupled with a huge ego.
The only thing they understand is being spoken to in the same language they use on others.
Trying to be logical with them is futile, because what they need psychologically is to engage with those that make them feel dumb on what feels like to them to be an equal footing.
So treating them as if they are smart and logical but misguided only feeds their pathology, in my experience.
Just sayin’.
And JMO.
Thanks for your consideration Nicholas. As I said in my last post I will not be conversing with this offensive idiot any longer.
Part of the problem is that she has mouthed off and insulted everyone, from Einstien downwards, for so long it is now impossible for her to backtrack and say, oops I was wrong. She is obliged to keep this going until everyone else gets bored and goes away.
Pathological , quite possibly. I remarked on a couple of occasions that she is displaying behaviour typical of a flat-earther. Such people are not amenable to reason. To think they are is to misunderstand their motivation for holding whacky ideas.
When someone start calling people things like “poophead” because they are not getting their own way in what is supposed to be a scientific discussion, you have to realise that you are dealing with someone with the mental maturity of a pre-school infant.
At that point I draw the line.
Quite clearly there is absolutely zero chance that she will accept anything anyone else presents to her, so to maintain the same desperate hope that logical discourse may arrive at an agreed understanding with this person would be foolish.
It was fun solving Willis’ problem and it is always useful having things you take for granted challenged from time to time, so the effort was not totally wasted. I’m unlikely to waste a similar amount of effort in the future as a result of this “learning experience” , so I’ll regard it as investment for the future.
Thanks for your thoughts.
LOL, quite a lot of projection.
“Quite clearly there is absolutely zero chance that she will accept anything anyone else presents to her.”
Not true. I will accept empirical evidence.
I presented empirical evidence, and the ideological mathematicians refuse to accept it. Hence your ranting is just projection.
I can’t believe the depths of denial of some people. It’s honestly quite disgusting to watch, and I hoped that a little bit of shaming would help. I guess I was wrong. It just cemented their beliefs even further.
As new comments rolled in, I saw the same pattern emerge:
Everyone avoids observational experiments I presented. They want to stay in fantasyland, where they can be right.
The only person who did not, Greg, had to fabricate his own numbers.
Imagine that: people who avoid reality and then want you to admit you’re wrong.
They dare not show any empirical evidence, attack you 50 to 1, and then call you rude as they do the same thing.
Whatever. Hopefully my fight with these fantasy dwellers helped somebody.
Let’s take a look at this video:
https://youtu.be/LckKhwJJgtE
Electrically Heated Water. Conduction based.
How would Willis fans solve this problem?
Energy In = Energy Out
Conduction from Bottom => Radiation(All 3D direction) + Conduction + Convection
It’s not easy to solve, and maybe I got it wrong (on purpose; I wanna see some nitpicking on purpose) but it’s easy to realize that just like his block, a steep gradient will form.
How would I solve it? Easy:
Conduction from Bottom => Radiation at the Top
Conductive Heat Flux => 0
Obviously our two approaches differ greatly. His predicts a gradient and mine: thermal equilibrium.
Now what does the video show?
Uhuh. So when are my attackers finally going to acknowledge reality? Never. Because you see … I’m just wrong. That’s the premise.
“Obviously our two approaches differ greatly. His predicts a gradient and mine: thermal equilibrium.”
The coffee pot shows a small but clear temperature gradient at the end of the video — warmer at the bottom and cooler at the top.
Well yeah, the video cuts off. I assumed my audience can think where it’s going.
CHF approches ZERO and clearly CSR != CHF.
The Willis method predicts a steep gradient, and CSR = CHF. We don’t see anything anywhere near that. Quite the opposite.
So your “empirical evidence’ is only wishful thinking of what you *hope* might happen in the future (but never does).
“easy to realize that just like his block, a steep gradient will form.”
No it is not easy to see. If it is so easy, I am sure you could show a few lines of calculations supporting your assertion .
PS. Remember that Willis was considering a system that ONLY had external thermal radiation as input, ONLY had conduction within the system, and ONLY had thermal radiation as output. This system has an internal electric heater as input, conduction, convection, and radiation within the system, and conduction, convection and radiation as outputs. Only a fool would think these are in any way analogous.
For this system, the “Willis method” (also known as the “Every Engineer and Physicist Everywhere method”) would include all the additional pathways for heat transfer available and would indeed predict a rather gradual gradient here — exactly as observed.
Tim
“and would indeed predict a rather gradual gradient here”
Don’t lie, Tim.
The Willis method would dilute the incoming surface area by the outgoing surface area, and create a very steep gradient.
In my video, the cooler top is the AIR above the water.
The top has a hole that allows convection. But even so, let’s say the small T difference you observed was 2C. (80C vs 82C)
k-value of air is ~0.025.
L=0.1, A=0.1
CHF = 0.05, CSR = sig(353.16)^4
Hmm, not even close to what the Willis method would predict.
“The Willis method would dilute the incoming surface area by the outgoing surface area, and create a very steep gradient.”
That doesn’t even make sense!
You haven’t even defined exactly what “problem” you are considering.
A) the gradient from the heated bottom to the unheated top?
B) the gradient from the heated interior to the unheated exterior?
C) other???
For (A), there is not just conduction through the ceramic walls of the pot transferring heat upward, there is also convection of the air within the pot, steam boiling away from the bottom and condensing at the top, and IR within the pot. These additional methods of heat transfer would make the interior nearly uniform in temperature (not a ‘steep gradient’ as you claim).
For (B), their apparently is a gradient across the walls. The interior is 100C (boiling water) but the exterior is ~ 80C based on the IR images. That gradient would be most equivalent to Willis’ scenario — heat applied on one side of a solid material (electric heating element in the interior), heat escaping form the other side of the solid material (IR and conduction to the air) and heat conducting thru a solid material. And here there is a definite ~ 20 C gradient in less than 1 cm.
Tim,
You won’t even do the math or show your setup because you know you’re lying.
“In my video, the cooler top is the AIR above the water.”
Are you comparing the solid concrete cube to the gaseous air above the water? Your calculation for CHF would seem to be
CHF = k A (Th-Tc) / L = 0.025 * 0.1 * 2 / 0.1 = 0.05
Where “L” would be the length from the hot side (the water) to the cold side (the lid); 10 cm is the right magnitude.
“A” would be the cross sectional area of the pot. Your estimate here is WAY off — the cross section is closer to 10 cm x 10 cm = 0.01 m^2. Consequently, the conductive heat flow would an order of magnitude smaller than you estimate.
That is all well and good — as far as it goes. But …
1) there is convection of the air within the pot (nothing to do with the ‘hole at the top’ that you mention). This will transfer orders of magnitude more heat than conduction through the air.
2) there is water boiling from the bottom, and then condensing on the sides. This also will transfer orders of magnitude more energy than conduction through the air.
3) there is radiation from the room back to the top of the pot. The net IR loss from the top of the pot is about 1/2 of what you calculate.
The fact that you don’t seem to even recognize any of these factors shows just how much you don’t know about heat flow in the very example you chose to present.
“The fact that you don’t seem to even recognize any of these factors shows just how much you don’t know about heat flow in the very example you chose to present.”
I do recognize them, and that you pointed out all the reasons there should be a steeper gradient than what we see according to your philosophy. And yet …
“Consequently, the conductive heat flow would an order of magnitude smaller than you estimate.”
You emphasized my point even further!
Thanks.
“you pointed out all the reasons there should be a steeper gradient than what we see ”
No, I pointed out the reasons the gradient should be SMALL, not LARGE. Only your continued misunderstandings make you expect that we would predict a large gradient for your coffee pot.
Tim,
“No, I pointed out the reasons the gradient should be SMALL, not LARGE.”
The pot is being delivered enough so that the water can boil, yes?
If you’re arguing that CHF is SMALL, then you’re arguing that CSR is LARGE.
CSR != CHF
QED
Your hypothesis is that CSR = CHF. You admitted CHF must get really small, so why purchase an electrically heated water boiler, if it can only produce an equally small CSR? The kind that can’t boil water at the top and have it escape as steam.
The video is for water, and water only. Air is unfair, as due to convection, the mass is not constant. As for the ceramic container, it’s also heating an unfixed mass.
Still need your response to this one Zoe:
“… OUT OF THE SURFACE, NOT IN THE GROUND.”
https://wattsupwiththat.com/2020/02/28/the-hot-and-cold-of-space/#comment-2928112
Is the wall of a mineshaft not a “surface” which emits IR into the same gaseous mixture as the air above the Earth’s surface? Please explain why (allegedly) only some of the IR photons emit from the “surface” of a mine’s walls but not the whole 335 W/m^2, which you claimed emits from the earth’s surface?
Please explain in plain English so I can easily understand your reasoning, thank you.
I don’t understand what the point of your question is.
It’s hot in a mine shaft. 335 is for the surface. It is much greater at depth. Certainly mineshafts wouldn’t be hot, if geothermal delivered 92 mW/m^2.
I think you understand it perfectly well and wish to avoid further embarrassment.
Do a day tour to the ore body of a deep mine given you’re so observations oriented.
The reason I have trouble understanding you, is because you’re arguing my point, while pretending it somehow refutes me. It looks to me to be some type of reverse psychology trick.
Space is a (near) vaccuum, so it doesn’t need to be insulated. It already is, just like a thermos bottle.
Willis, Thot = 388.5 K, Tcold = 222.8 K. The heat flows are 1227 watts radiating away from the hot side, and 133 watts radiating away from the cold side.
I think my answer is a bit different than the others because I used T = 4 K for the temperature of space.
Works for me, Loren. You’ve gotten the answer that just about everyone here got. Well, everyone but Zoe, who claims that both sides (the heated and the unheated side) of the concrete block will have the same temperature, absolute zero, viz:
Shaking my head …
w.
Willis, you call your self an honest man, yet you did not give the answer I gave – ~120C both sides.
Zoe, I quoted your answer. It appears you’ve given two answers. And yes, I am indeed an honest man, which is why I quoted your actual answer without altering it in any way.
In fact, your first answer was
Now, that’s no answer at all … but of course, you claimed it was an answer:
Zoe Phin March 1, 2020 at 8:08 am Edit
Finally, you gave this answer, which I quoted the conclusion of above:
So you aren’t even telling the truth about your own answers.
BZZZZT! Nice try. Vanna White, what prizes do we have for our failed contestants?
w.
Willis,
I gave one answer.
The thing you quoted was not an answer, you LIAR.
Loren,
What experiments convinced you?
Getting back to the question of whether it is hot or cold in space…
I think it is clear enough, but no one has to take my word for it.
Let hear what the man who wrecked the Enterprise, almost killed Kirk, and BTW also was the inventor of Corinthian Leather, let’s hear what he has to say:
https://youtu.be/5vwHLMs04XA
Getting back to the question of whether it is hot or cold in space…
I think it is clear enough, but no one has to take my word for it.
Let hear what the man who wrecked the Enterprise, almost k!lled Kirk, and BTW also was the inventor of Corinthian Leather, let’s hear what he has to say:
https://youtu.be/5vwHLMs04XA
I think that ultimately, this is where Zoe goes wrong. She incorrectly attributes this idea to Willis (and by extension, to all engineers and physicists):
“He believes that
Hot Side Radiation = Conductive Heat Flux = Cold Side Radiation.”
where (with a few simplifications in coefficients) Zoe defines
HSR = σ(Th)⁴
CSR = σ(Tc)⁴
CHF = Th-Tc
What we actually believe in this scenario is that
Hot Side Heat = Conductive Heat Flux = Cold Side Heat
The ‘HSH’ is the NET heat into to the hot side. This is not the same as ‘HSR’. In fact they have opposite signs. ‘HSR’ = σ(Th)⁴ is the radiation FROM the hot side, not radiation TO the hot side. The hot side input (call it HSI) is fixed at 1360 W/m^2 and is not a function of Th.
(HSI-HSR) = HSH = CHF = CSR
[ PS CSR is the same as CSH, since the only heat leaving the cold side is radiation = σ(Tc)⁴ ]
No, the issue that your cult believes matter would split its energy between matter and space. I don’t believe that, and so I correctly identify that by Willis’ way HSR = CHF.
If you believe in backradiation, you must believe in backconduction as well.
Both are retarded views, actually.
Light has a pressure, remember?
Willis’ block can’t backradiate to its source, because such a pressure would cancel out what it receives.
So you guys must really believe the block gets 130W on the hot side. The hot side can’t be 383K by this. And so you have 221K on both sides, and CHF != CSR.
What you’re trying to debunk is very well thought out. But it means nothing to your because you don’t understand EM pressure and reality in general. Your premise of backradiation is false.
https://www.britannica.com/science/light/Radiation-pressure
Zoe Phin March 3, 2020 at 9:36 pm
Zoe, I neither said nor do I believe that the hot side radiation is equal to the conductive heat flux. That’s not true.
Note that, unlike you, I don’t call you a “liar”. That’s a bridge too far.
I simply know that you are mistaken. I said that AT STEADY STATE conductive heat flux (CHF) must be equal to the cold side radiation (CSR). Not the hot side. The cold side.
Now, I asked you several times—if at steady state the conductive heat flux is greater than the cold side radiation … where does the excess heat go? It can’t go into heating the block, it’s at steady state.
And if at steady state the heat flux is less than the cold side radiation … where does the extra heat come from? The “ether”?
Each time, you’ve tap-danced around the question … and you may well do so again. However, be very clear that people see that you have been extremely unwilling to answer the question, and have drawn the obvious conclusion.
w.
Gosh, Willis, you’re attempts at ridicule are hilarious.
You continue to compare a differential with an absolute.
Do you know the difference between profit/loss and assets/liabilities?
Just as I predicted, you have dodged the question, and come up with some nonsensical side-track.
Zoe, do you truly not realize that people clearly see that you don’t have the courage to actually engage with the issues? Are you completely unaware that people are pointing at you and laughing at your plain and fancy tap-dancing to avoid answering a simple question?
w.
Oh Willis, you are so stupid.
Why do you compare kinetic energy to a difference of kinetic energies of two locations. Why?
Your definition of “tap dancing” is me trying to reason with a fool that can’t see or refuses to see his foolishness.
Aaaand, once again Zoe doesn’t answer the question …
This is my surprised face.
w.
PS—You ask “Do you know the difference between profit/loss and assets/liabilities?”
In my last job, I was the Chief Financial Officer for a business with sales of $40 million per year … so yes, dear Zoe, I do understand the difference, and once again your arrogance comes back to bite you in the fundamental orifice.
Willis don’t you understand that you’re were arguing that profits must equal total liabilities?
Why are you comparing a difference of kinetic energies at two different locations to kinetic energy at one of them?
You keep accusing others of what you do: tap dancing around questions asked of you.
I have answered enough of your questions. I have presented empirical evidence, and you, and you, didn’t even bother looking for any.
All this shows that your integrity is not that high.
More tap-dancing and hand-waving, more personal attacks, and in all of it, Zoe STILL hasn’t answered the simple question about conservation of energy …
w.
Suppose you have a hot rock — say 100 C. This rock can be placed in a room where everything is either 80 C, 20 C, or -40 C
In which room will the rock cool most quickly to 90 C?
a) the -40 C room.
b) all will take the same time.
How does your answer change if the walls are still those temperatures, but all the air can be pumped out to eliminate conduction and convection through the air, and so that radiation is the primary means of cooling?
a) the -40 C room.
b) all will take the same time.
c) the rock cannot cool.
Intuition and simple calculations confirm that the rock will cool quickest in the coolest surroundings — with or without air in the room.
But if you believe “Willis’ block can’t backradiate to its source, because such a pressure would cancel out what it receives”, ie because the ‘hot side of the concrete’ is cooler than the sun, then you also believe that the walls can’t ‘backradiate’ (also simply known as ‘radiate’) to the warmer rock. If there is no radiation from cooler walls to warmer rock, then the rock would radiate identically in all rooms and cool identically.
PS. The sun only takes up about 0.001% of the hemisphere facing the ‘hot side’ of the cube. This means that in about any direction, the whole question of “can’t backradiate to its source” is moot. The hot side radiation almost never is radiating back to its source, so it has no problem, at all radiating to the 99.999% of its view that is 2.7 K.
This is not a backradiation problem.
Willis’ block starts at zero kelvin, right?
The rock here is the hottest thing, and it’s not a constant raw energy source.
You inverted everything and demonstrated nothing.
It only looks like a backradiation problem to you because you believe in two way photon flow.
There is no such thing. The potential for heat flow is one way. And it’s greatest for greatest T diff.
You can’t believe in radiation pressure and two way photon flow.
That’s the point.
I choose the ideas that lead to the least contradiction, best if not at all.
Don’t forget that the walls are blocking the rock from cooling to 3K space somewhere behind the walls.
The rock must heat up. LMAO
“The sun only takes up about 0.001% of the hemisphere facing the ‘hot side’ of the cube.”
And that’s why the sun’s radiation from source was reduced to 1361 from 63000000. And that 1361 is now 100% of its view. And you want to send 1162 (was it? I won’t check) right back in 1361’s face.
1 metre by 1 metre by 1 metre concrete block floating in outer space.
The block is insulated on four sides, a perfect insulator, no heat at all is lost from the four insulated sides.
the emissivity “epsilon” and absorptivity across the spectrum are both 0.95.
the thermal conductivity “k” of the concrete is equal to 0.8 watts per metre per kelvin (0.8 W/m K^-1)
it gets full-time sunshine on the front side at a rate of 1360 watts per square metre (W/m2).
what will be the temperature T_hot of the hot side and the temperature T_cold of the opposite cold side?
–
At a 1 molecule thinness the temperature on both sides would be equal hence half as hot as expected if the back surface was also insulated. 65.67C
At a million metres the back surface would be at a very low temperature just above 62 K.
This would be enough to drain the minute amount of energy that makes it across the block.
Th surface of the block receiving radiation has to heat up to a higher temperature to force heat across the concrete gradient. The maximum it can heat to is double the energy it absorbs.
The soldering iron I think someone referred to it as.
–
129.77 C or 512.92K is the Temp of the hot side.
Similar range to the surface of the moon different albedo.
–
The cold side is more difficult. The bulk of the thermal mass built up by absorption of energy is at the heated end which radiates most of the radiation back out.
The small amount that “conducts” 0.8 watts per metre per kelvin finally gives that level to the other side which immediately radiates it into space giving it a temp of -210.4 C or 62.75 K
–
1 metre by 1 metre by 1 metre concrete block floating in outer space.
The block is insulated on four sides, a perfect insulator, no heat at all is lost from the four insulated sides.
the emissivity “epsilon” and absorptivity across the spectrum are both 0.95.
the thermal conductivity “k” of the concrete is equal to 0.8 watts per metre per kelvin (0.8 W/m K^-1)
it gets full-time sunshine on the front side at a rate of 1360 watts per square metre (W/m2).
what will be the temperature T_hot of the hot side and the temperature T_cold of the opposite cold side?
–
At a 1 molecule thinness the temperature on both sides would be equal hence half as hot as expected if the back surface was also insulated. 65.67C
At a million metres the back surface would be at a very low temperature just above 62 K.
This would be enough to drain the minute amount of energy that makes it across the block.
Th surface of the block receiving radiation has to heat up to a higher temperature to force heat across the concrete gradient. The maximum it can heat to is double the energy it absorbs.
The soldering iron I think someone referred to it as.
–
129.77 C or 412.92K is the Temp of the hot side.
Similar range to the surface of the moon different albedo.
–
The cold side is more difficult. The bulk of the thermal mass built up by absorption of energy is at the heated end which radiates most of the radiation back out.
The small amount that “conducts” 0.8 watts per metre per kelvin finally gives that level to the other side which immediately radiates it into space giving it a temp of -210.4 C or 62.75 K
–
Musing. Reflectance, or albedo, seems to break the rule of equal and opposite reaction. Why is this?
If 10% say of incoming energy is reflected into space where is the 10% of energy that should be going in the other direction!
Puzzled.
Willis, this is an excellent example of the fact that the energy leaving the physical domain cannot be specified as a boundary condition in mathematical models: https://judithcurry.com/2018/05/22/energy-budgets-climate-system-domains-and-internal-variability/
Application of results from the divergence theorem, outlined in that post, leads to the equation for overall conservation of energy for the system in this problem: sum of energy out = energy in.
Dan Hughes, thank you for that reference. I remember seeing your piece on Judith Curry’s website, but now I have gone back and read it all more carefully, along with Willis’s comments and replies to others. About the “boundary condition” notion applied to climate models in respect to greenhouse gases, I have long thought, “That can’t be right.” The variable emitter of longwave energy out to space is supplied by a variable-performance heat engine. The concept of the atmosphere as a radiative insulating blanket is only a part of the picture. Willis has been doing great work over the years, observing and describing emergent climate phenomena such as thunderstorms, and the governor concept.